A body is projected with a veocity of 40ms−1. After 2s it fcrosses a vertical pole of height 20.4m Find the angle of projection and horizontal range of projectile. (g = 9.8 ms^(-2).
Let θbe the angle of projection of projectile with horizontal. Taking vertical upward motion of the projectile from point projection up to the top of vertical pole, we have
uy=usinθ=40sinθ,ay=−9.8m/s2,
t=2s,y=20.4m
As y=u−yt+12a−yt2
:. 20.4=40sinθ×2+12(−9.80×22
or isθ=(20.4+19.6)/80=1/2orθ=302
Horizontal range, R=u2sin2θg
=4029.8sin(2×30∘)=141.4m.
Topper's Solved these Questions
- KINEMATICSBOOK - PRADEEPCHAPTER - KINEMATICSEXERCISE - NCRT Exercises
22 Videos
- KINEMATICSBOOK - PRADEEPCHAPTER - KINEMATICSEXERCISE - Additional Exercises
13 Videos
- KINEMATICSBOOK - PRADEEPCHAPTER - KINEMATICSEXERCISE - 6 Long answer (NCERT)
9 Videos
- GRAVIATIONBOOK - PRADEEPCHAPTER - GRAVIATIONEXERCISE - Assertion-Reason Type Questions
19 Videos
- LAWS OF MOTIONBOOK - PRADEEPCHAPTER - LAWS OF MOTIONEXERCISE - Assertion- Reason Type Questions
17 Videos
Similar Questions
A body is projected with a velocity 30ms−1 at an angle of 30∘ with the vertical. Find (i) the maximum height (ii) time of flight and (iii) the horizontal range of the projectile. Take g=10m/s∘.
View SolutionA body is projected with a velocity of 30ms−1 at an angle of 30∘ with the vertical. Find the maximum height, time of flight and the horizontal range.
View Solution
Knowledge Check
A body is projected with a initial velocity of (8ˆi+6ˆj)ms−1 . The horizontal range is (g=10ms−2)
A9.6 mB14 mC50 mD100 mA stone is projected horizontally with a velocity 9.8ms−1 from a tower of height 100 m. Its velocity one second after projection is (g=9.8ms−2).
A9.8ms−1B4.9ms−1C9.8√2ms−1D4.9√2ms−1A particle is projected with velocity of 10ms at an angle of 15° with horizontal.The horizontal range will be(g=10ms2)
A10mB5mC2.5mD1m
A ball projected with a velocity of 10m/s at angle of 30∘ with horizontal just clears two vertical poles each of height 1m. Find separation between the poles.
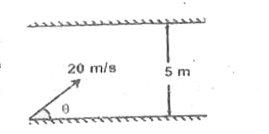
View SolutionA projectile is projected with a speed = 20 m/s from the floor of a 5 m high room as shown. Find the maximum horizontal range of the projectile and the corresponding angle of projection θ.
View SolutionA body is projected at 45∘ with a velocity of 20ms−1 has a range of 30m .The decrease in range due to air resistance is?(g=10ms−2)
View SolutionA stone is thrown with a speed of 10ms−1 at an angle of projection 60∘. Find its height above the point of projection when it is at a horizontal distance of 3m from the thrower ? (Take g=10ms−2)
View SolutionA body is projected horizontally with speed 20ms−1 . The speed of the body after 5s is nearly
View Solution
