a. Calculate the inductance of an air core solenoid containing 300 turns if the length of the solenoid is 25.0cm and its cross -sectional area is 4.00cm2.
b. Calculate the self -induced emf in the solenoid if the current through it is decreasing at the rate of 50.0A/s.
To solve the problem step by step, we will break it down into two parts: (a) calculating the inductance of the solenoid and (b) calculating the self-induced emf in the solenoid.
Part (a): Calculate the Inductance of the Solenoid
1. Identify Given Values:
- Number of turns, N=300
- Length of the solenoid, l=25.0cm=0.25m
- Cross-sectional area, A=4.00cm2=4.00×10−4m2
2. Calculate the Number of Turns per Unit Length:
n=Nl=3000.25=1200turns/m
3. Use the Formula for Inductance of a Solenoid:
The inductance L of a solenoid is given by:
L=μ0n2Al
where μ0=4π×10−7H/m (permeability of free space).
4. Substitute the Values into the Formula:
L=(4π×10−7)×(1200)2×(4×10−4)×(0.25)
5. Calculate the Inductance:
- First, calculate n2:
n2=12002=1440000
- Now substitute:
L=(4π×10−7)×(1440000)×(4×10−4)×(0.25)
- Calculate:
L=(4×3.14×10−7)×(1440000)×(4×10−4)×(0.25)
L≈180×10−6H=180μH
Part (b): Calculate the Self-Induced EMF
1. Identify Given Values:
- Rate of change of current, didt=−50.0A/s
2. Use the Formula for Induced EMF:
The self-induced emf E is given by:
E=−Ldidt
3. Substitute the Values:
E=−(180×10−6)×(−50)
4. Calculate the Induced EMF:
E=180×10−6×50=9×10−3V=9mV
Final Answers:
- (a) The inductance of the solenoid is 180μH.
- (b) The self-induced emf in the solenoid is 9mV.
- Number of turns, N=300
- Length of the solenoid, l=25.0cm=0.25m
- Cross-sectional area, A=4.00cm2=4.00×10−4m2
n=Nl=3000.25=1200turns/m
The inductance L of a solenoid is given by:
L=μ0n2Al
where μ0=4π×10−7H/m (permeability of free space).
L=(4π×10−7)×(1200)2×(4×10−4)×(0.25)
- First, calculate n2:
n2=12002=1440000
- Now substitute:
L=(4π×10−7)×(1440000)×(4×10−4)×(0.25)
- Calculate:
L=(4×3.14×10−7)×(1440000)×(4×10−4)×(0.25)
L≈180×10−6H=180μH
- Rate of change of current, didt=−50.0A/s
The self-induced emf E is given by:
E=−Ldidt
E=−(180×10−6)×(−50)
E=180×10−6×50=9×10−3V=9mV
- (a) The inductance of the solenoid is 180μH.
- (b) The self-induced emf in the solenoid is 9mV.
Topper's Solved these Questions
- ELECTROMAGNETIC INDUCTIONBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Check point
60 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Taking it together
119 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Level 2 Subjective
20 Videos
- CURRENT ELECTRICITYBOOK - DC PANDEY ENGLISHCHAPTER - CURRENT ELECTRICITYEXERCISE - Medical entrances gallery
97 Videos
- ELECTROMAGNETIC WAVESBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROMAGNETIC WAVESEXERCISE - Sec C
22 Videos
Similar Questions
A winding wire which is used to frame a solenoid can bear a maximum 10 A. current If length of solenoid is 80 cm and its corss sectional raedius is 3 cm , then required length of winding wire is (B =0.2T)
View Solution(a) Calculate the self-inductance of a solenoid that is tightly wound with wire of diameter 0.10cm, has a cross-sectional area 0.90cm2 and is 40cm long
(b) If the current through the solenoid decreases uniformly from 10 A to 0A in 0.10s, what is the emf induced between the ends of the solenoid?
View Solution
Knowledge Check
A solenoid of cross-sectional area 2×10−4m2 and 900 turns has 0.6Am2 magnetic moment. Then the current flowing through it is
A2.4AB2.34mAC3.33AD3.33mAThe self inductance of a solenoid that has a cross-sectional area of 1cm2, a length of 10 cm and 1000 turns of wire is
A0.86mHB1.06mHC1.26mHD1.46mH
What will happen to the inductance of a solenoid
a. when the number of turns and the length are double keeping the area of cross section same?
b. when the air inside the solenoid is replaced by iron of relative permeability μr?
View SolutionA long solenoid of cross-sectional area 5.0cm2 is wound with 25 turns of wire per centimetre. It is placed in the middle of a closely wrapped coil of 10 turns and radius 25 cm as shown.
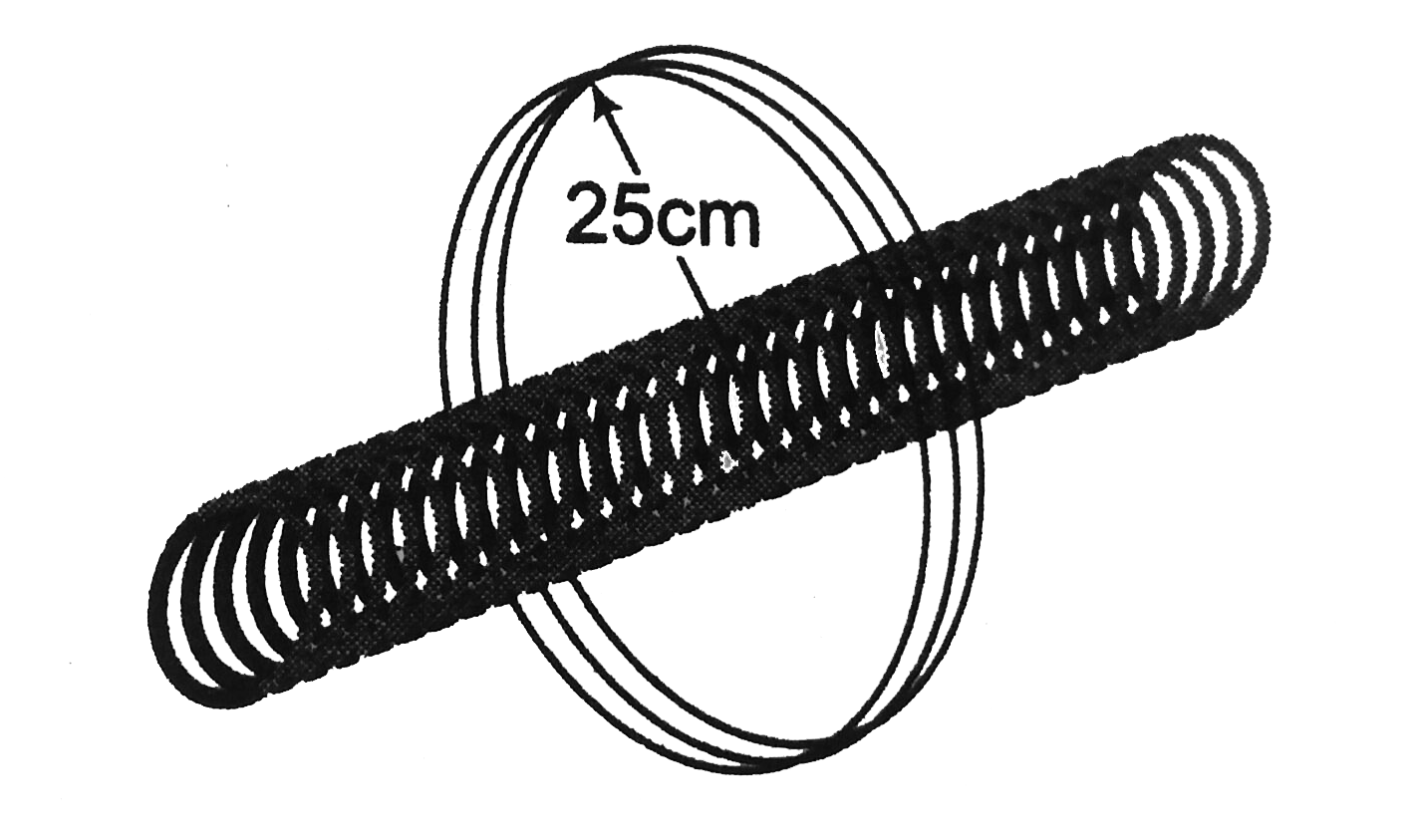
(a) What is the emf induced in the coil when the current through the solenoid is decreasing at a rate −0.20A/s ?
(b) What is the electric field induced in the coil?
View SolutionWhat is the self inductance of an air core solenoid 50cm long and 2cm radius if it has 500 turns? Find the magnetic flux when a current of 2 amp passes through it
View SolutionA solenoid has 2000 turns would over a length of 0.30 m. The area of its cross-section is 1.2×10−3m2. If an initial current of 2 A in the solenoid is reversed in 0.25 s, then the emf induced in the coil is
View SolutionA straight solenoid of length 1m has 5000 turns in the primary and 200 turns in the secondary. If the area of cross section is 4cm2, the mutual inductance will be
View SolutionA long solenoid having 200 turns per centimeter carries a current of 1.5A. At the center of the solenoid is placed a coil of 100 turns of cross-sectional area 3.14×10−4m2 having its axis parallel to the field produced by the solenoid. When the direction of current in teh solenoid is reversed within 0.05s, the induced emf in the coil is
View Solution
