The magnetic flux linked with a vector area →A in a uniform magnetic field →B is
B×A
AB
B.A
BA
The correct Answer is:C
To find the magnetic flux linked with a vector area →A in a uniform magnetic field →B, we can follow these steps:
1. Understand the Concept of Magnetic Flux:
Magnetic flux (ΦB) is defined as the product of the magnetic field (→B) and the area (→A) through which the field lines pass. It quantifies the number of magnetic field lines passing through a given area.
2. Formula for Magnetic Flux:
The magnetic flux linked with an area vector in a magnetic field is given by the dot product of the magnetic field vector and the area vector. The formula is:
ΦB=→B⋅→A
3. Dot Product Explanation:
The dot product takes into account both the magnitude of the vectors and the angle between them. It is defined as:
→B⋅→A=|→B||→A|cos(θ)
where θ is the angle between the magnetic field vector and the area vector.
4. Conclusion:
From the above understanding, we can conclude that the magnetic flux linked with the vector area →A in a uniform magnetic field →B is given by:
ΦB=→B⋅→A
5. Select the Correct Option:
Among the given options, the correct answer is:
Option 3: →B⋅→A
Magnetic flux (ΦB) is defined as the product of the magnetic field (→B) and the area (→A) through which the field lines pass. It quantifies the number of magnetic field lines passing through a given area.
The magnetic flux linked with an area vector in a magnetic field is given by the dot product of the magnetic field vector and the area vector. The formula is:
ΦB=→B⋅→A
The dot product takes into account both the magnitude of the vectors and the angle between them. It is defined as:
→B⋅→A=|→B||→A|cos(θ)
where θ is the angle between the magnetic field vector and the area vector.
From the above understanding, we can conclude that the magnetic flux linked with the vector area →A in a uniform magnetic field →B is given by:
ΦB=→B⋅→A
Among the given options, the correct answer is:
Option 3: →B⋅→A
Topper's Solved these Questions
- BOOK - DC PANDEY ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Taking it together119videos
ELECTROMAGNETIC INDUCTION
- BOOK - DC PANDEY ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Medical entrance special format questions17videos
ELECTROMAGNETIC INDUCTION
- BOOK - DC PANDEY ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Example71videos
ELECTROMAGNETIC INDUCTION
- BOOK - DC PANDEY ENGLISHCHAPTER - CURRENT ELECTRICITYEXERCISE - Medical entrances gallery97videos
CURRENT ELECTRICITY
- BOOK - DC PANDEY ENGLISHCHAPTER - ELECTROMAGNETIC WAVESEXERCISE - Sec C22videos
ELECTROMAGNETIC WAVES
Similar Questions
What is the direction of the force acting on a charged particle q, moving with a velocity →v a uniform magnetic field →B ?
View SolutionAn electron is launched with velocity →v in a uniform magnetic field →B. The angle θ between →vand→B lies between 0 and π2. Its velocity vector →v returns to its initial value in a time interval of
View Solution
Knowledge Check
- Question 1 - Select One
The torque and magnetic potential energy of a magnetic dipole in most stable position ina uniform magnetic field →B having magnetic moment →m will be
A−mB,zeroBmB,zeroCzero,mBDzero,−mB
Is magnetic flux a scalar or vector?
View SolutionAn alpha particle moving with the velocity →v=uˆi+ˆj ,in a uniform magnetic field →B=Bˆk . Magnetic force on alpha particle is
View SolutionA conductor (shown in the figure) carrying constant current I is kept in the x−y plane in a uniform magnetic field →B. If →F is the magnitude of the total magnetic force acting on the conductor, then the correct statement(s) is (are)
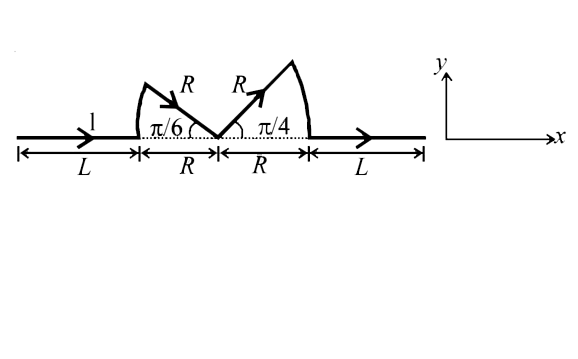 View Solution
View SolutionDefine one tesla using the expression fo the magnetic force acting on a particle of charge .q. moving with velocity →v in a magnetic field →B.
View SolutionThe horizontal component of earth's magnetic field is 3×10−5Wb/m2. The magnetic flux linked with a coil of area 1m2 and having 5 turns, whose plane is normal to the magnetic field, will be
View SolutionA portion is fired from origin with velocity →v=v0ˆj+v0ˆk in a uniform magnetic field →B=B0ˆj. In the subsequent motion of the proton
View SolutionThe rigid conducting thin wire frame carries an electric current I and this frame is inside a uniform magnetic field →B as shown in Fig. 1.146. Then,
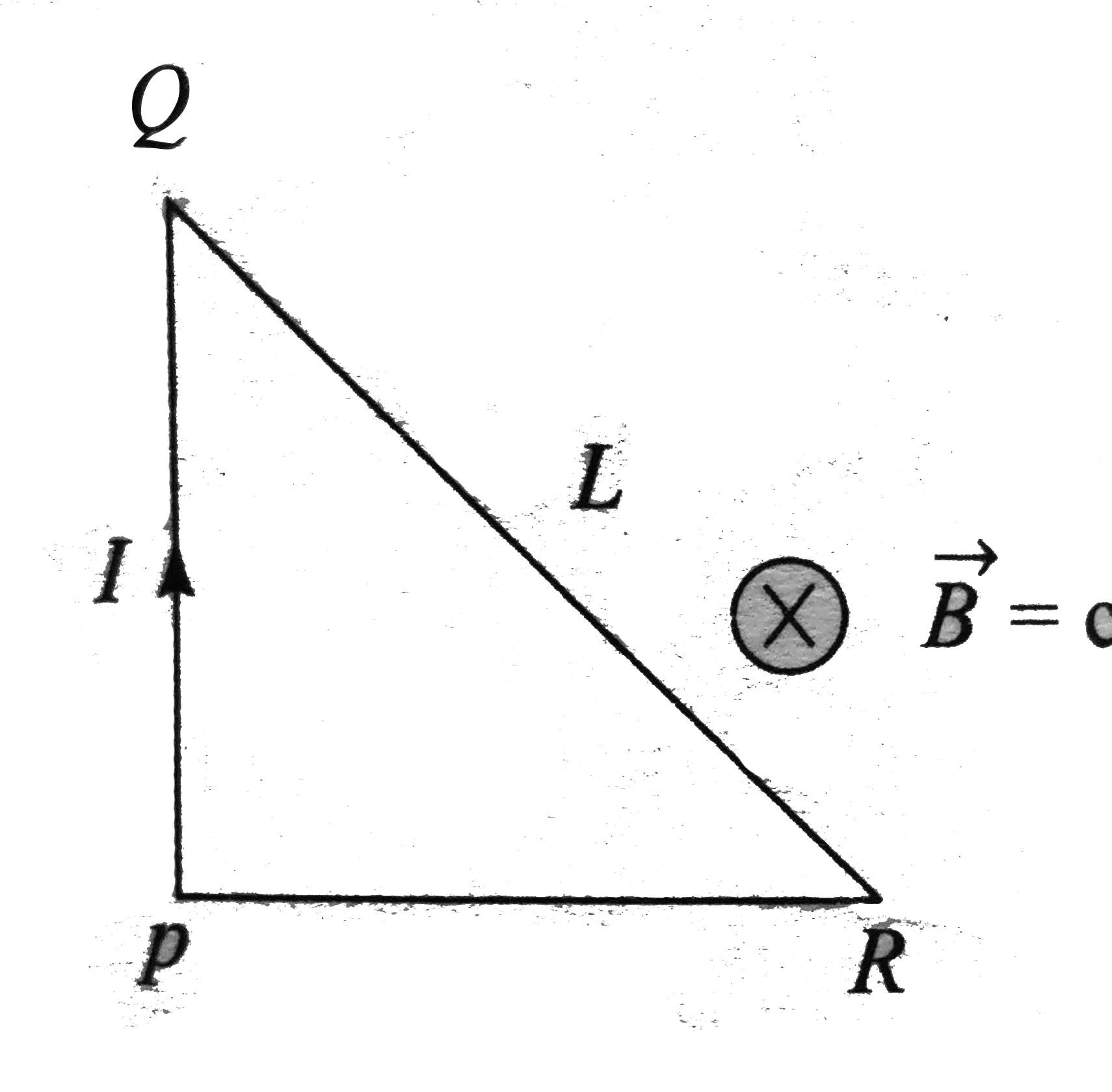 View Solution
View Solution

