A capacitor is charged through a potential difference of 200 V, when 0.1C charge is stored in it. The amount of energy released by it, when it is discharged is
5 J
10 J
20 J
2.5 J
The correct Answer is:B
To find the amount of energy released by a capacitor when it is discharged, we can use the formula for the energy stored in a capacitor. Here’s a step-by-step solution:
Step 1: Identify the formula for energy stored in a capacitor
The energy (U) stored in a capacitor is given by the formula:
U=12CV2
where:
- U is the energy stored,
- C is the capacitance,
- V is the voltage across the capacitor.
However, we can also express this in terms of charge (Q):
U=12QV
where:
- Q is the charge stored in the capacitor.
Step 2: Substitute the known values
From the problem, we know:
- Q=0.1C
- V=200V
Now we can substitute these values into the formula:
U=12×Q×V
U=12×0.1C×200V
Step 3: Calculate the energy
Now we perform the calculation:
U=12×0.1×200
U=12×20
U=10J
Step 4: Conclusion
The amount of energy released by the capacitor when it is discharged is:
Energy released=10J
The energy (U) stored in a capacitor is given by the formula:
U=12CV2
where:
- U is the energy stored,
- C is the capacitance,
- V is the voltage across the capacitor.
U=12QV
where:
- Q is the charge stored in the capacitor.
From the problem, we know:
- Q=0.1C
- V=200V
U=12×Q×V
U=12×0.1C×200V
Now we perform the calculation:
U=12×0.1×200
U=12×20
U=10J
The amount of energy released by the capacitor when it is discharged is:
Energy released=10J
Topper's Solved these Questions
- ELECTROSTATIC POTENTIAL AND CAPACITANCE BOOK - NCERT FINGERTPS ENGLISHCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITANCE EXERCISE - HOTS
5 Videos
- ELECTROSTATIC POTENTIAL AND CAPACITANCE BOOK - NCERT FINGERTPS ENGLISHCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITANCE EXERCISE - EXEMPLAR PROBLEMS
3 Videos
- ELECTROMAGNETIC WAVESBOOK - NCERT FINGERTPS ENGLISHCHAPTER - ELECTROMAGNETIC WAVESEXERCISE - Assertion And Reason
15 Videos
- MAGNETISM AND MATTER BOOK - NCERT FINGERTPS ENGLISHCHAPTER - MAGNETISM AND MATTER EXERCISE - NCERT Exemplar
5 Videos
Similar Questions
A capacitor when charged by a potential difference of 200 Volts, stores a charge of 0.1C. By discharging energy liberated by the capacitor is-
View SolutionAn ideal capacitor of capacitance 0.2μF is charged to a potential difference of 10V. The charging battery is than disconnected. The capacitor is then connected to an ideal inductor of self inductance 0.5mH. The current at a time when the potential difference across the capacitor is 5V is :
View SolutionA capacitor of capacitance C is charged to a potential difference V0. The charged battery is disconnected and the capacitor is connected to a capacitor of unknown capacitance Cx. The potential difference across the combination is V. The value of Cx should be
View SolutionA parallel plate capacitor is charged to a potential difference of 50 volts. It is then discharged through a resistance for 2 seconds and its potential drops by 10 volts. Calculate the fraction of energy stored in the capacitance.
View SolutionA capacitor of capacitance 5μF is charged to a potential difference 200 V and then allowed to dischage through a resistance 1kΩ. The cahrge on the capacitor at the instant the current through the resistance is 100 Ma, is (in μC):
View SolutionA capacitor of capacitance C is charged to a potential differece V0. The terminals of the charged capacitor are then connected to those of an uncharged capacitor of capacitance C/2.
a. Compute the original charge of the system.
b. Find the final potential differce across each capacitor.
c. Find the final energy of the system.
d. Calculate the decrease in energy when the capacitors are connected.
e. Where did the ''lost'' energy go?
View Solutionn the circuit shown in the figure, the capacitor C is charged to a potential Vo The heat generated and charge flow, in the circuit to when the switch S is closed, is
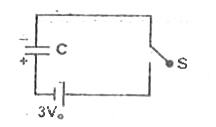
View SolutionA capacitor of capacitance C1 is charged to a potential V1 while another capacitor of capacitance C2 is charged to a potential difference V2 . The capacitors are now disconnected from their respective charging batteries and connected in parallel to each other .
(a) Find the total energy stored in the two capacitors before they are connected.
(b) Find the total energy stored in the parallel combination of the two capacitors.
(c ) Explain the reason for the difference of energy in parallel combination in comparison to the total energy before they are connected.
View SolutionA capacitor is charged to a certain potential and then allowed to discharge through a resistance R. The ratio of charge on the capacitor to current in the circuit
View SolutionA capacitor of capacity 2μF is charged to a potential difference of 12V. It is then connected across an inductor of inductance 6μH. At an instant when potential difference across the capacitor is 6V, what is the current(in A)?
View Solution
