A certain resonance curve describes a mechanical oscillating system with logarithmic damping decrement λ=1.60. For this curve find the ration of the maximum displacemetn amplitude to the displacement amplitude at a very low frequency.
In general for displacement amplitude
a=F0m1√(ω20−ω2)2+4β2ω2
=F0m1√(ω2−ω20+2β2)2+4β2(ω20−β2)
Thus η=aresalow=ω20√4β2(ω20−β2).=ω202β√ω20−β2
But βω0=λ/2π√1+(λ/2π)2,λ2π=β√ω20−β2
Hence η=ω202β2.λ2π=121+(λ2π)2λ2π=2.90
Topper's Solved these Questions
- OSCILLATIONS AND WAVESBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - OSCILLATIONS AND WAVESEXERCISE - Electric Oscillations
56 Videos
- OSCILLATIONS AND WAVESBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - OSCILLATIONS AND WAVESEXERCISE - Elastic Waves
39 Videos
- OPTICSBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - OPTICSEXERCISE - Exercise
2 Videos
- PHYSICAL FUNDAMENTALS OF MECHANICSBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - PHYSICAL FUNDAMENTALS OF MECHANICSEXERCISE - Relativistic Mechanics
49 Videos
Similar Questions
A ball of mass m when suspended by a spring stretches the latter by Δl. Due to external vertical lforce varying according to a harmonic law with amplitude F0 the ball performs forced oscillations. The logarighmic damping decrement if equal to λ. Neglecting the mass the spring, find the angular frequency of the external force at which the displacemetn amplitude of the ball is maximum. What is the magnitude of that amplitude ?
View SolutionThe forced harmonic oscillations have equal displacement amplitude at frequencies ω1=400s−1 and ω2=600s−1. Find the resonance frequency at which the displacement amplitude is maximum.
View Solution
Knowledge Check
A particle executes SHM in a straight line path .The amplitude of oscillation is 2cm. When the displacement of the particle from the mean position is 1cm the numerical value of acceleration is equal to the numerical value of velocity. Then find the frequency of SHM.
A√3/2πB3/2πC3/√2πD√3/π
A string 120cm in length sustains a standing wave with the points of the string at which the displacement amplitude is equal to 3.5mm being separated by 1.50cm. Find the maximum displacement amplitude . To which overtone do these oscillations correspond ?
View SolutionA point performs damped oscillations with frequency ω and damping coefficient β according to the (4.1b). Find the initial amplitude a0 and the initial phase α if at the moment t=0 the displacement of the point and its velocity projection are equal to
(a) x(0)=0 and ux(0)=.x0 ,
(b) x(0)=x0 and vx(0)=0.
View SolutionA body A mass m1=1kg and body B of mass m2=4.1kg. The body A perform free vertical harmonic oscillations with the amplitude 1.6cm and frequency 25Hz. Neglecting the mass of the spring , find the maximum and m inimum value of force that the system exerts on the hearing surface.
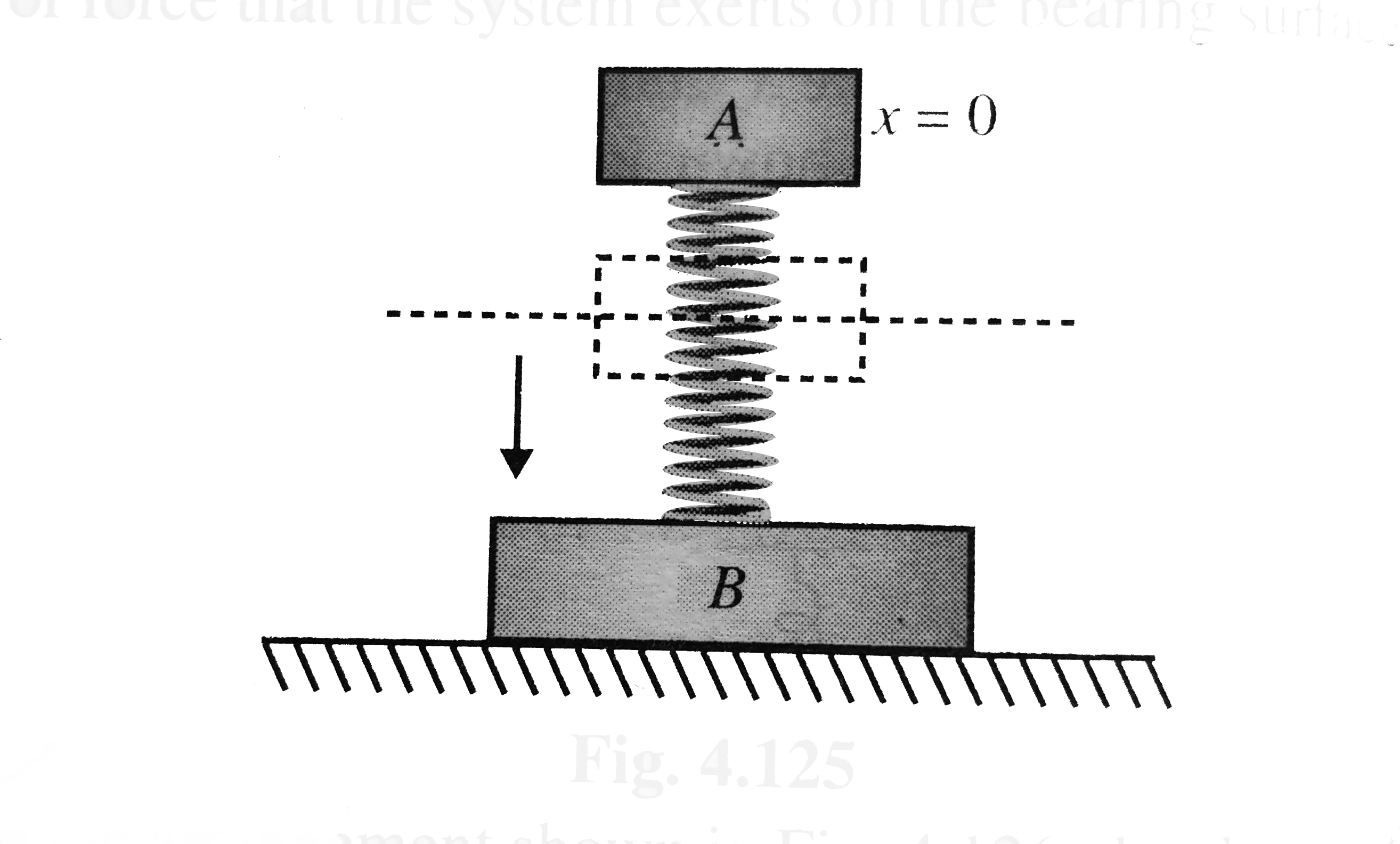
View SolutionA point performs dampled oscillation with frequency ω=25s−1. Find the damping coefficient β if at the initial moment the velocity of the point is equal to zero and its displacement from the equalibrium position is η=1.020 times lesss than the amplitude at that moment.
View SolutionA point isotropic source generates sound oscillations with frequency v=1.45kHz. At a distance r0=5.0m from the source the displacement amplitude of particles of the medium is equal to the a0=50μm, and at the point A located at a distance r=10.0m from the source the displacement ampitude is η=3.0 times less than a0 . Find :
(a) the damping coefficient γ of the wave,
(b0 the velocity oscillation amplitude of particles of the medium at the point A.
View SolutionA string of mass m is fixed at both ends. The fundamental tone oscillations are excited with circular frequency ω and maximum displacement amplitude amax . Find :
(a) the maximum kinetic energy of the string,
(b) the mean kinetic energy of the string averaged over one oscillation period.
View SolutionA particle executes SHM on a straight line path. The amplitude of oscillation is 2cm. When the displacement of the particle from the mean position is 1cm, the numerical value of magnitude of acceleration is equal to the mumerical value of velocity. Find the frequency of SHM (in Hz).
View Solution
