A clock fixed on a wall shows time 04:25:37. What time will its image in a plane mirror hanging on an opposite vertical wall show?
07:43:32
07:43:32
07:34:23
43:27:36
The correct Answer is:C
To find out what time the image of a clock showing 04:25:37 will display in a plane mirror, we can follow these steps:
1. Understand the Clock's Position:
The clock shows the time as 04:25:37, which means it is 4 hours, 25 minutes, and 37 seconds past midnight.
2. Determine the Reference Time:
In a mirror, the image of the clock will be inverted. To find the inverted time, we can use a reference time of 6:00:00 (6 o'clock). This is because the clock's face can be thought of as a circle where 12 o'clock is at the top and 6 o'clock is at the bottom.
3. Calculate the Time Remaining to 6:00:00:
We need to calculate how much time is left from 04:25:37 to 06:00:00.
- From 04:25:37 to 05:00:00 is 34 minutes and 23 seconds.
- From 05:00:00 to 06:00:00 is 1 hour.
- Therefore, the total time from 04:25:37 to 06:00:00 is:
- 1 hour + 34 minutes + 23 seconds = 1 hour, 34 minutes, and 23 seconds.
4. Add the Remaining Time to 6:00:00:
Now, we add the remaining time to 6:00:00 to find the time displayed in the mirror:
- 6:00:00 + 1:34:23 = 7:34:23.
5. Final Result:
Thus, the time shown by the image of the clock in the mirror will be 07:34:23.
Final Answer:
The image of the clock in the mirror will show 07:34:23.
---
The clock shows the time as 04:25:37, which means it is 4 hours, 25 minutes, and 37 seconds past midnight.
In a mirror, the image of the clock will be inverted. To find the inverted time, we can use a reference time of 6:00:00 (6 o'clock). This is because the clock's face can be thought of as a circle where 12 o'clock is at the top and 6 o'clock is at the bottom.
We need to calculate how much time is left from 04:25:37 to 06:00:00.
- From 04:25:37 to 05:00:00 is 34 minutes and 23 seconds.
- From 05:00:00 to 06:00:00 is 1 hour.
- Therefore, the total time from 04:25:37 to 06:00:00 is:
- 1 hour + 34 minutes + 23 seconds = 1 hour, 34 minutes, and 23 seconds.
Now, we add the remaining time to 6:00:00 to find the time displayed in the mirror:
- 6:00:00 + 1:34:23 = 7:34:23.
Thus, the time shown by the image of the clock in the mirror will be 07:34:23.
The image of the clock in the mirror will show 07:34:23.
Topper's Solved these Questions
- GEOMETRICAL OPTICSBOOK - AAKASH SERIESCHAPTER - GEOMETRICAL OPTICSEXERCISE - PRACTICE SHEET (EXERCISE I LEVEL -II (ADVANCED) MORE THAN ONE CORRECT TYPE QUESTIONS)
2 Videos
- GEOMETRICAL OPTICSBOOK - AAKASH SERIESCHAPTER - GEOMETRICAL OPTICSEXERCISE - PRACTICE SHEET (EXERCISE I LEVEL -II (ADVANCED) LINKED COMPREHENSION TYPE QUESTIONS)
6 Videos
- GEOMETRICAL OPTICSBOOK - AAKASH SERIESCHAPTER - GEOMETRICAL OPTICSEXERCISE - PRACTICE SHEET (EXERCISE I LEVEL -I (MAIN) STRAIGHT OBJECTIVE TYPE QUESTIONS)
11 Videos
- ELEMENTS OF VECTORSBOOK - AAKASH SERIESCHAPTER - ELEMENTS OF VECTORSEXERCISE - QUESTIONS FOR DESCRIPTIVE ANSWERS
10 Videos
- LAWS OF MOTIONBOOK - AAKASH SERIESCHAPTER - LAWS OF MOTIONEXERCISE - PRACTICE EXERCISE
106 Videos
Similar Questions
Figure shows a torch sending a parallel beam of light fixed on a wall and a plane mirror which forms a spot on the wall. As the mirror moves towards the wall, the spot on the wall moves

View SolutionAn unnumbered wall clock shows time 04:25:37 where 1st term represents hours 2ndrepresents minutes and the last term represents seconds. What time will its image in a plane mirror show.
View SolutionA watch shows time as 3:25 when seen through a mirror, time appeared will be
View SolutionStatement I: Keeping a point object fixed, if a plane mirror is moved, the image will also move.
Statement II: In case of a plane mirror, distance of object and its image is equal from any point on the mirror
View SolutionDraw a ray diagram showing the formation of image of a point object by a plane mirror.
View SolutionA person 1.6 m tall is standing at the center between two walls three meter high. What is the minimum size of a plane mirror fixed on the wall in front of him, if he is to see the full height of the wall behind him?
View SolutionA man of height =3/2m,wants to see himself in plane mirror from top to bottom. The plane mirror is inclined with vertical wall at angle α=53∘. If the least size of mirror to see him 3nm, the distance of eye from mirror is d=3m, find the value of m
View Solution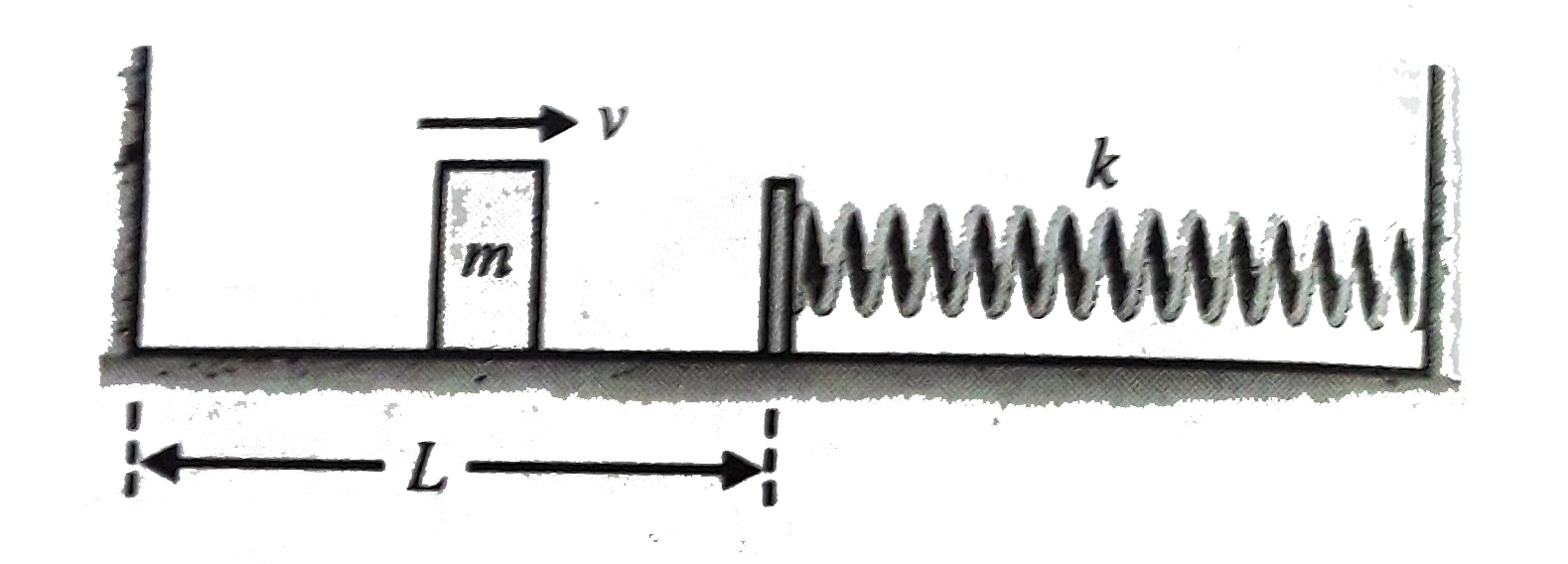
A spring having a spring constant k is fixed to a vertical wall as shown in Fig. A block of mass m moves with velocity v torards the spring from a parallel wall opposite to this wall. The mass hits the free end of the spring compressing it and is decelerated by the spring force and comes to rest and then turns the spring is decelerated by the spring force and comes to rest and then turns back till the spring acquires its natural length and contact with the spring is broken. In this process, it regains its angular speed in the opposite direction and makes a perfect elastice collision on the opposite left wall and starts moving with same speed as before towards right. The above processes are repeated and there is periodic oscillation.
Q. What is the time period of oscillation ?
View SolutionA plane mirror is placed at a distance of 50 cm from a concave mirror of focal length 16 cm. Where should a short object be placed between the mirrors and facing both the mirrors so that its virtual image in the plane mirror coincides with the real image in concave mirror? What is the ratio of the sizes of the two images?
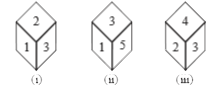
View SolutionA dice is trowns three times and its three different positions are given below. Find the number on the face opposite the face showing 3.
View Solution
