A current of exists in a square loop of edge . Find the magnetic field B at the centre of the square loop.
Solution: The magnetic fields at the centre due to the four
sides will be equal in magnitude and direction. The field
due to one side will be
Thus,
Hence, the net field at the centre of the loop will be
4 xx 5*66 xx (10^-6) T= 22*6 xx (10^-6) T .
Topper's Solved these Questions
- BOOK - HC VERMACHAPTER - MAGNETIC FIELD DUE TO CURRENTEXERCISE - Short Answer12videos
MAGNETIC FIELD DUE TO CURRENT
- BOOK - HC VERMACHAPTER - MAGNETIC FIELD DUE TO CURRENTEXERCISE - Objective 112videos
MAGNETIC FIELD DUE TO CURRENT
- BOOK - HC VERMACHAPTER - MAGNETIC FIELD DUE TO CURRENTEXERCISE - Exercises61videos
MAGNETIC FIELD DUE TO CURRENT
- BOOK - HC VERMACHAPTER - MAGNETIC FIELDEXERCISE - Exercises61videos
MAGNETIC FIELD
- BOOK - HC VERMACHAPTER - MAGNETIC PROPERTIES OF MATTEREXERCISE - Exercises9videos
MAGNETIC PROPERTIES OF MATTER
Similar Questions
Figure shows a square loop made from a uniform
wire. Find the magnetic field at the centre of the square
if a battery is connected between the points A and C.
 View Solution
View SolutionFigure shows a square loop made from a uniform wire. Find the magnetic field at the centre of the square if a battary is connected between points A and C.
 View Solution
View Solution
Knowledge Check
- Question 1 - Select One
A square loop of side a carries a current I. The magnetic field at the center of the loop is
A2μ0I√2πaBμ0I√2πaC4μ0I√2πaDμ0Iπa - Question 2 - Select One
A square loop of edge 'a' carries a current l. The magnetic field at the centre of loop is
Aμ0l2√2πaB2√2μ0lπaCμ0l√2πaDμ0l√2πa - Question 3 - Select One
Find magnetic field at centre P if length of side of square loop is 20 cm.
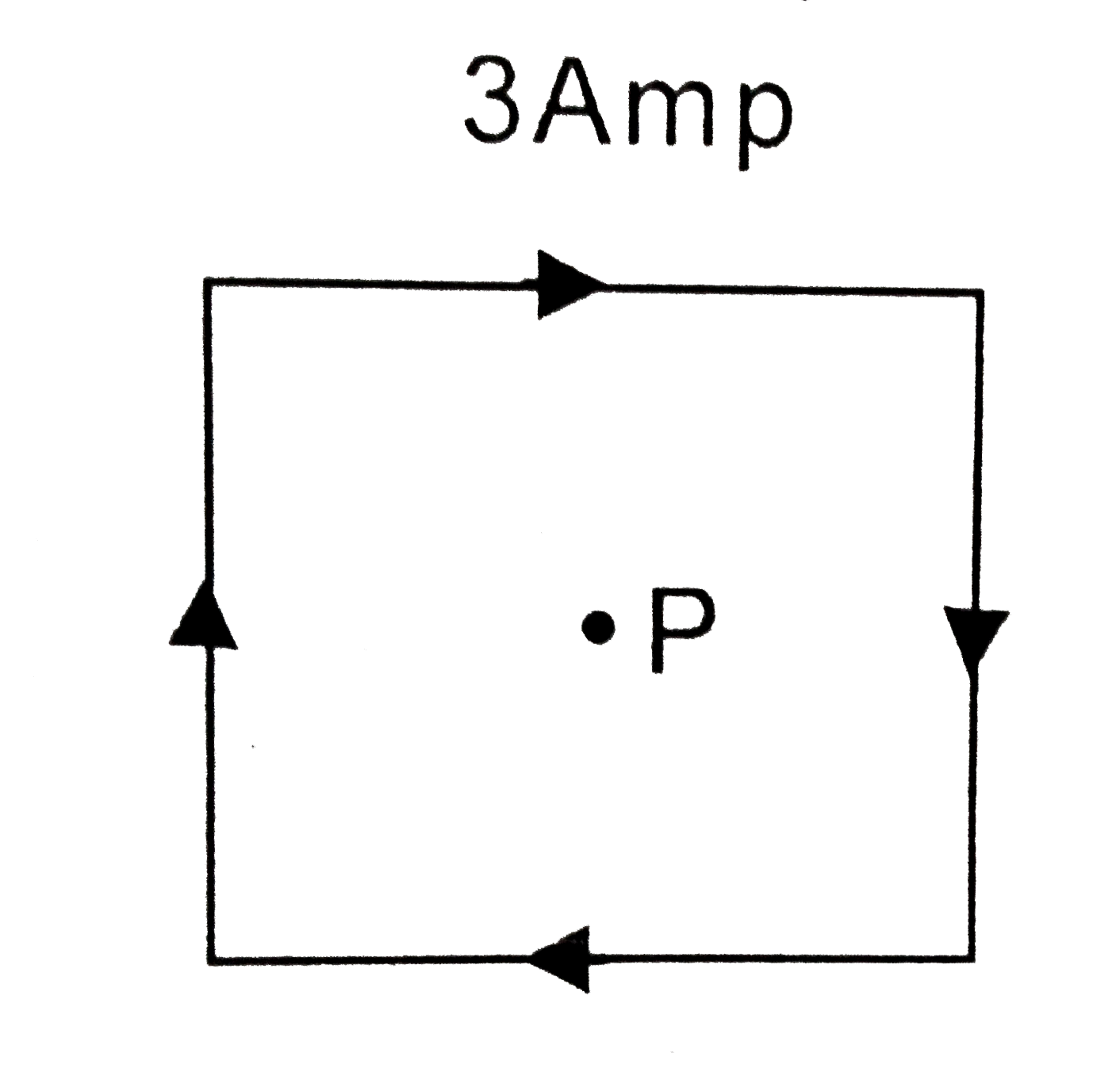 A12√2×10−6TB12×10−6TC6×10−6TD6√2×10−6T
A12√2×10−6TB12×10−6TC6×10−6TD6√2×10−6T
If a straight conductor of length 40cm bent in the form of a square and the current 2A is allowed to pass through square, then find the magnetic induction at the centre of the square loop.
View SolutionConsider the situation described in the previous problem. Suppose the current I enters the loop at the point A and leaves it at the point B. Find the magnetic field at the centre of the loop.
View SolutionA square conducting loop of side length L carries a current I.The magnetic field at the centre of the loop is
View SolutionA circular loop of radius 0.0157 m carriesa current of 2.0 A. The magnetic field at the centre of the loop is
View SolutionA current carrying wire is in form of square loop of side length 10 cm. current in wire is 5A. Find out magnetic field at centre of loop.
View Solution

