A cylinder of radius R and length L is placed in a uniform electric field E parallel to the axis. The total flux for the surface of the cylinder is given by
2πR2E
πR2/E
(πR2/πR)/E
zero
The correct Answer is:D
To find the total electric flux through the surface of a cylinder placed in a uniform electric field parallel to its axis, we can follow these steps:
1. Understand the Configuration:
- We have a cylinder of radius R and length L.
- The electric field E is uniform and parallel to the axis of the cylinder.
2. Apply Gauss's Law:
- Gauss's Law states that the total electric flux Φ through a closed surface is equal to the charge enclosed Qenclosed divided by the permittivity of free space ϵ0:
Φ=Qenclosedϵ0
3. Determine the Charge Enclosed:
- In this case, since the cylinder is placed in a uniform electric field and there are no charges inside the cylinder, we have:
Qenclosed=0
4. Calculate the Total Flux:
- Substituting the value of Qenclosed into Gauss's Law gives:
Φ=0ϵ0=0
5. Conclusion:
- Therefore, the total electric flux through the surface of the cylinder is:
Φ=0
Final Answer:
The total flux for the surface of the cylinder is 0.
---
- We have a cylinder of radius R and length L.
- The electric field E is uniform and parallel to the axis of the cylinder.
- Gauss's Law states that the total electric flux Φ through a closed surface is equal to the charge enclosed Qenclosed divided by the permittivity of free space ϵ0:
Φ=Qenclosedϵ0
- In this case, since the cylinder is placed in a uniform electric field and there are no charges inside the cylinder, we have:
Qenclosed=0
- Substituting the value of Qenclosed into Gauss's Law gives:
Φ=0ϵ0=0
- Therefore, the total electric flux through the surface of the cylinder is:
Φ=0
The total flux for the surface of the cylinder is 0.
Topper's Solved these Questions
- ELECTROSTATICSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATICSEXERCISE - Assertion and Reason
15 Videos
- ELECTROSTATICSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATICSEXERCISE - Match the columns
5 Videos
- ELECTROSTATICSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATICSEXERCISE - Check point 1.5
20 Videos
- ELECTROSTATIC POTENTIAL AND CAPACITORSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITORSEXERCISE - (C) Chapter exercises
50 Videos
- GRAVITATIONBOOK - DC PANDEY ENGLISHCHAPTER - GRAVITATIONEXERCISE - All Questions
135 Videos
Similar Questions
A cylinder of radius R and length l is placed in a uniform electric field E parallel to the axis of the cylinder. The total flux over the curved surface of the cylinder is
View SolutionConsider a cylindrical surface of radius R and length l in a uniform electric field E. Compute the electric flux if the axis of the cylinder is parallel to the field direction.
View SolutionIn a uniform electric field find the total flux associated with the given surfaces
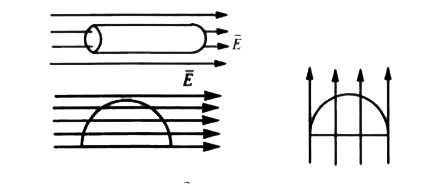
View SolutionA Gaussian surface in the cylinder of cross-section πa2 and length L is immersed in a uniform electric field E with the cylinder axis parallel to the field. The flux ϕ of the electric field through the closed surface is
View SolutionA hollow half cylinder surface of radius R and length l is placed in a uniform electric field →E. Electric field is acting perpendicular on the plane ABCD. Find the flux through the curved surface of the hollow cylindrical surface

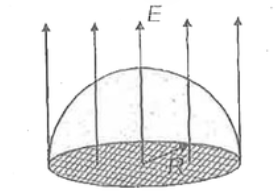
View SolutionA hemispherical surface of radius R is kept in a uniform electric field E as shown in figure. The flux through the curved surface is
View SolutionA hemispherical body of radius R is placed in a uniform electric field E. What is the flux linked with the curved surface if, the field is (a) parallel to the base, (b) perpendicular to the base.
View SolutionA hemispherical body of radius R is placed in a uniform electric field E. What is the flux linked with the curved surface if, the field is (a) parallel to the base, (b) perpendicular to the base.
View Solutiona hemispherical hollow body is placed in a uniform electric field E. the total flux linked with the curved surface is

View Solutiona hemispherical hollow body is placed in a uniform electric field E. the total flux linked with the curved surface is

View Solution
