An infinite number of particles each of mass are placed on the positive X-axis of from the origin. Find the magnitude of the resultant gravitational force on mass kept at the origin.
The correct Answer is:D
To find the magnitude of the resultant gravitational force on a mass m kept at the origin due to an infinite number of particles each of mass m placed at positions 1m,2m,4m,8m,… on the positive X-axis, we will follow these steps:
Step 1: Understand the Gravitational Force Formula
The gravitational force F between two masses m1 and m2 separated by a distance r is given by:
F=Gm1m2r2
In our case, both masses are m, so the force exerted by each mass on the mass at the origin will be:
F=Gm2r2
Step 2: Identify the Positions and Distances
The particles are located at distances r1=1m,r2=2m,r3=4m,r4=8m,…. The general position of the n-th particle can be expressed as:
rn=2n−1 for n=1,2,3,…
Step 3: Calculate the Gravitational Force from Each Particle
The gravitational force exerted by the n-th particle on the mass at the origin is:
Fn=Gm2(2n−1)2=Gm24n−1
Step 4: Sum the Forces from All Particles
The total gravitational force F0 on the mass at the origin is the sum of the forces from all particles:
F0=∞∑n=1Fn=∞∑n=1Gm24n−1
Step 5: Recognize the Series as a Geometric Series
The series can be rewritten as:
F0=Gm2∞∑n=014n
This is a geometric series with first term a=1 and common ratio r=14.
Step 6: Calculate the Sum of the Geometric Series
The sum of an infinite geometric series is given by:
S=a1−r
Substituting the values:
S=11−14=134=43
Step 7: Substitute Back to Find F0
Now substituting back into the expression for F0:
F0=Gm2⋅43
Final Result
Thus, the magnitude of the resultant gravitational force on the mass m kept at the origin is:
F0=43Gm2
The gravitational force F between two masses m1 and m2 separated by a distance r is given by:
F=Gm1m2r2
In our case, both masses are m, so the force exerted by each mass on the mass at the origin will be:
F=Gm2r2
The particles are located at distances r1=1m,r2=2m,r3=4m,r4=8m,…. The general position of the n-th particle can be expressed as:
rn=2n−1 for n=1,2,3,…
The gravitational force exerted by the n-th particle on the mass at the origin is:
Fn=Gm2(2n−1)2=Gm24n−1
The total gravitational force F0 on the mass at the origin is the sum of the forces from all particles:
F0=∞∑n=1Fn=∞∑n=1Gm24n−1
The series can be rewritten as:
F0=Gm2∞∑n=014n
This is a geometric series with first term a=1 and common ratio r=14.
The sum of an infinite geometric series is given by:
S=a1−r
Substituting the values:
S=11−14=134=43
Now substituting back into the expression for F0:
F0=Gm2⋅43
Thus, the magnitude of the resultant gravitational force on the mass m kept at the origin is:
F0=43Gm2
Topper's Solved these Questions
Similar Questions
Infinite number of bodies, each of mass 2kg are situated on X-axis at distance 1m,2m,4m,8m, respectively from the origin, What is the resulting gravitational potential due to this system at the origin ?
View SolutionInfinite number of masses, each of mass 3 kg are placed along the y-axis at y = 1 m, 3 m, 9 m 27 m ... The magnitude of resultant gravitational potential in terms of gravitatinal constant at the origin (y = 0) is
View Solution
Knowledge Check
- Question 1 - Select One
A mass m is placed at point P lies on the axis of a ring of mass M and radius R at a distance R from its centre. The gravitational force on mass m is
AGMm√2R2BGMm2R2CGMm2√2R2DGMm4R2
Infinite number of bodies, each of mass 2kg, are situated on x-axis at distance 1m,2m,4m,8m......... respectively, from the origin. The resulting gravitational potential due to this system at the origin will be
View SolutionInfinite number of bodies, each of mass 2kg, are situated on x-axis at distance 1m,2m,4m,8m......... respectively, from the origin. The resulting gravitational potential the to this system at the origing will be
View SolutionInfinite number of masses, each of 1kg, are placed along the x-axis at x=±1m,±2m,±4m,±8m,±16m.. The gravitational of the resultant gravitational potential in term of gravitaitonal constant G at the origin (x=0) is
View SolutionIf four different masses m1,m2,m3 and m4 are placed at the four corners of a square of side a, the resultant gravitational force on a mass m kept at the centre is
View SolutionA particle of mass m is situated at a distance d from one end of a rod of mass M and length L as shown in diagram. Find the magnitude of the gravitational force between them
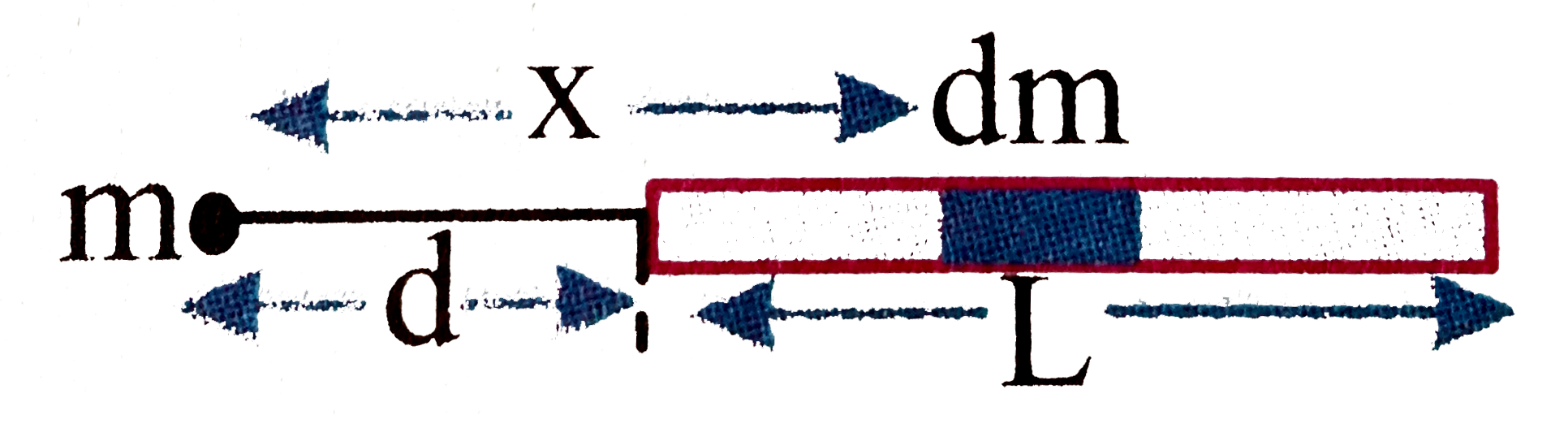 View Solution
View SolutionWhen n number of particles each of mass m are at distances x1=1,x2=2,x3=3,……….,xn=n units from origin on the x-axis, then find the distance of their centre of mass from origin.
View SolutionAn infinite number of charges, each of magnitude q, are placed along x-axis at x = 1m, 2m, 4m, 8m, 16m and so on but the consecutive charges are of opposite sign starting with +q at x = 1m. A point charge q0 , kept at the origin, experiences a force of magnitude :
View Solution

