(a) Determine the value of phase difference between the current and the voltage in the given series LCR circuit. Fig. 7.36

(b) Calculate the value of the additional capacitor which may be joined suitably to the capacitor C that would make the power factor of the circuit unity.
(a) In the given LCR series circuit R =400Ω,C=2μF=2×10−6F,L=100mH=0.1H and ω=100s−1
∴XC=1Cω=12×10−6×1000=500Ω and XL=Lω=0.1×1000=100Ω
If current leads the voltage in phase by ϕ, then
tanϕ=XC−XLR=(500−100)ω400Ω=1⇒ϕ=45∘ or π4
(b) If we want to make the power factor (cosϕ) of the circuit unity, we have cosϕ=1 and it means that ϕ=0∘. It is possible only if X.C−XL=0, where X.C is the new value of capacitive reactance.
⇒X.C=XL=100Ω⇒1C.ω=100
⇒C.=1100ω=1100×1000=10×10−6F=10μF
To make the resultant capacitance C. = 10 uF, a capacitor of 10 -2 = 8 uF capactiance should be joined in parallel to the given capacitor of 2 uF.
Topper's Solved these Questions
- ALTERNATING CURRENT BOOK - U-LIKE SERIESCHAPTER - ALTERNATING CURRENT EXERCISE - LONG ANSWER QUESTIONS -II
12 Videos
- ALTERNATING CURRENT BOOK - U-LIKE SERIESCHAPTER - ALTERNATING CURRENT EXERCISE - SELF ASSESSMENT TEST (SECTION -A) (MULTIPLE CHOICE QUESTIONS)
4 Videos
- ALTERNATING CURRENT BOOK - U-LIKE SERIESCHAPTER - ALTERNATING CURRENT EXERCISE - SHORT ANSWER QUESTIONS
32 Videos
- ATOMSBOOK - U-LIKE SERIESCHAPTER - ATOMSEXERCISE - SELF ASSESSMENT TEST (SECTION C)
2 Videos
Similar Questions
Find the value of phase lag/lead between the current and voltage in the given CLR circuit, fig. Without making any other change, find the value of additional capacitor, such that when joined switably to C=2μF, would make the power factor of this circuit unity.
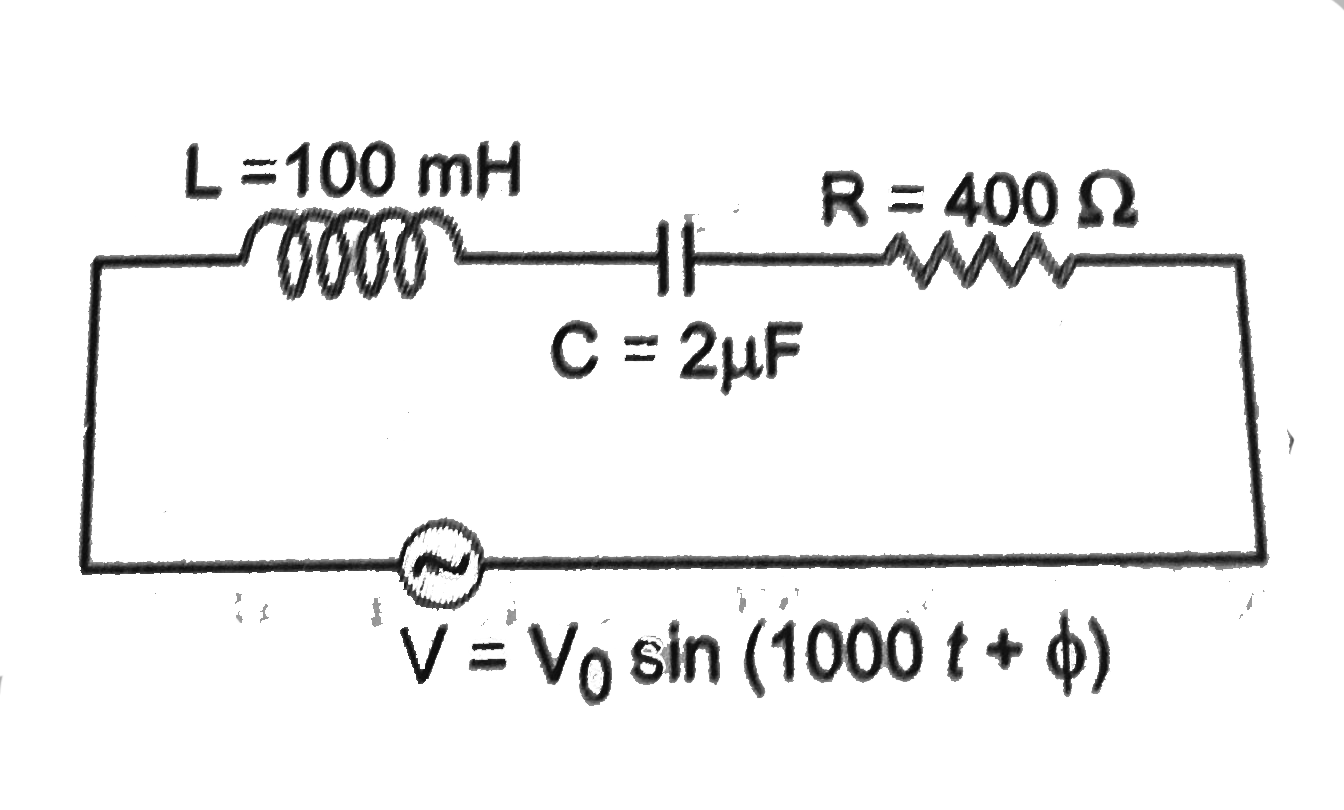
View SolutionFind the value of phase lag/lead between the current and voltage in the given CLR circuit, fig. Without making any other change, find the value of additional capacitor, such that when joined switably to C=2μF, would make the power factor of this circuit unity.

View Solution
Knowledge Check
The phase difference between the current and voltage of a LCR series a.c. circuit at resonance is
A0Bπ2Cπ4DπThe phase difference between the current and voltage of LCR circuit in series combination at resonance is
A0Bπ/2CπD−πThe phase difference between voltage and current in an LCR series circuit is
Azero alwaysBπ/4 alwaysCπDbetween 0 and π/2
(i) Find the value of the phase difference between the current and the voltage in the series LCR circuit shown below. Which one leads in phase : current or voltage ?
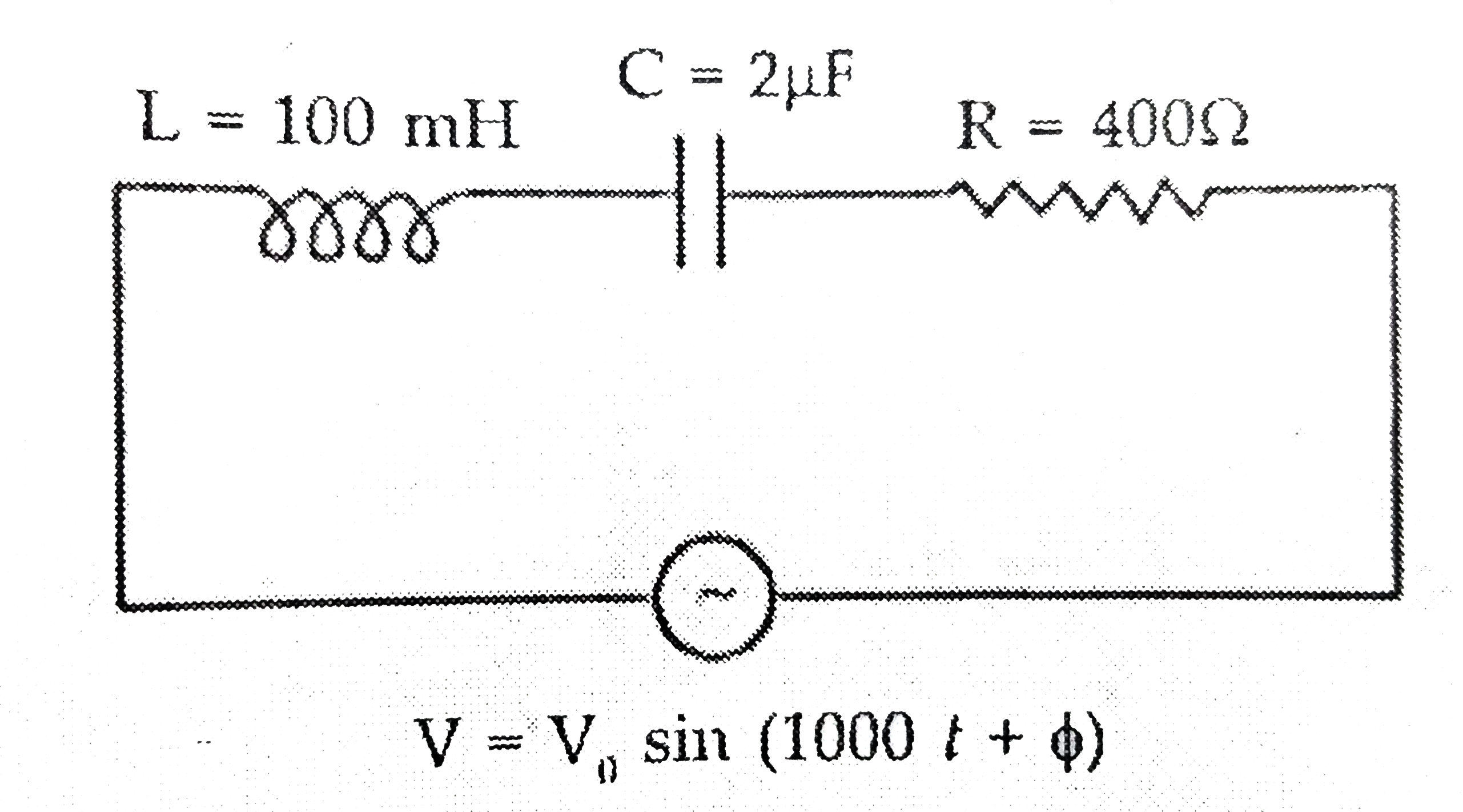
(ii) What making any other change, find the value of the additional capacitor C, to be connected in parallel with the capacitor C, in order to make the power factor of the circuit unity.
View SolutionFind the values of the phase difference between the current and the voltage in the series LCR circuit shown below, which one leads in phase: current or voltage?
View SolutionThe phase difference between voltage and current in series L-C circuit is
View Solution(i) Find the value of the phase difference between the current and the voltage in the series LCR circuit shown below. Which one leads in phase : current or voltage ?

(ii) What making any other change, find the value of the additional capacitor C, to be connected in parallel with the capacitor C, in order to make the power factor of the circuit unity.
View SolutionThe phase difference between voltage and current in series L-C circuit is
View Solution
