A disc of mass 2 kg and radius 0.2 m is rotating with angular velocity 30rad s−1. What is angular velocity, if a mass of 0.25 kg is put on periphery of the disc ?
24 rad s−1
36 rad s−1
15 rad s−1
26 rad s−1
The correct Answer is:A
To solve the problem, we will follow these steps:
Step 1: Calculate the Moment of Inertia of the Disc
The moment of inertia I of a disc is given by the formula:
I=12mr2
where m is the mass of the disc and r is its radius.
Given:
- Mass of the disc, m=2kg
- Radius of the disc, r=0.2m
Substituting the values:
I=12×2×(0.2)2=12×2×0.04=0.04kg m2
Step 2: Calculate the Moment of Inertia of the Added Mass
The moment of inertia Im of a point mass m at a distance r from the axis of rotation is given by:
Im=mr2
where m=0.25kg (the mass added at the periphery) and r=0.2m.
Substituting the values:
Im=0.25×(0.2)2=0.25×0.04=0.01kg m2
Step 3: Calculate the New Moment of Inertia
The new moment of inertia I′ after adding the mass is:
I′=I+Im=0.04+0.01=0.05kg m2
Step 4: Apply Conservation of Angular Momentum
Since no external torque is acting on the system, the angular momentum before adding the mass must equal the angular momentum after adding the mass.
The initial angular momentum L1 is:
L1=Iω1=0.04×30=1.2kg m2/s
where ω1=30rad/s.
The final angular momentum L2 is:
L2=I′ω2
where ω2 is the new angular velocity we want to find.
Setting L1=L2:
1.2=0.05ω2
Step 5: Solve for the New Angular Velocity
Rearranging the equation to find ω2:
ω2=1.20.05=24rad/s
Final Answer
The new angular velocity ω2 after adding the mass is:
ω2=24rad/s
---
The moment of inertia I of a disc is given by the formula:
I=12mr2
where m is the mass of the disc and r is its radius.
- Mass of the disc, m=2kg
- Radius of the disc, r=0.2m
I=12×2×(0.2)2=12×2×0.04=0.04kg m2
The moment of inertia Im of a point mass m at a distance r from the axis of rotation is given by:
Im=mr2
where m=0.25kg (the mass added at the periphery) and r=0.2m.
Im=0.25×(0.2)2=0.25×0.04=0.01kg m2
The new moment of inertia I′ after adding the mass is:
I′=I+Im=0.04+0.01=0.05kg m2
Since no external torque is acting on the system, the angular momentum before adding the mass must equal the angular momentum after adding the mass.
L1=Iω1=0.04×30=1.2kg m2/s
where ω1=30rad/s.
L2=I′ω2
where ω2 is the new angular velocity we want to find.
1.2=0.05ω2
Rearranging the equation to find ω2:
ω2=1.20.05=24rad/s
The new angular velocity ω2 after adding the mass is:
ω2=24rad/s
Topper's Solved these Questions
- ROTATIONBOOK - DC PANDEY ENGLISHCHAPTER - ROTATIONEXERCISE - Check point 9.3
15 Videos
- ROTATIONBOOK - DC PANDEY ENGLISHCHAPTER - ROTATIONEXERCISE - (A) Chapter Exercises
83 Videos
- ROTATIONBOOK - DC PANDEY ENGLISHCHAPTER - ROTATIONEXERCISE - Check point 9.1
20 Videos
- RAY OPTICSBOOK - DC PANDEY ENGLISHCHAPTER - RAY OPTICSEXERCISE - Integer type q.
14 Videos
- ROTATIONAL MECHANICSBOOK - DC PANDEY ENGLISHCHAPTER - ROTATIONAL MECHANICSEXERCISE - Subjective Questions
2 Videos
Similar Questions
A disc of mass 1 kg and radius 0.1 m is rotating with angular velocity 20 rad s−1. What is angular velocity, if a mass of 0.25 kg is put on periphery of the disc?
View SolutionA disc of mass 1 kg and radius 0.1 m is rotating with angular velocity 20 rad/s. What is angular velocity (in rad/s) if a mass of 0.5 kg is put on the circumference of the disc ?
View Solution
Knowledge Check
A wheel of mass 5kg and radius 0.40m is rolling on a road without sliding with angular velocity 10rads−1. The moment of ineria of the wheel about the axis of rotation is 0.65kgm2. The percentage of kinetic energy of rotate in the total kinetic energy of the wheel is.
A22.4%B11.2%C88.8%D44.8%
A uniform circular disc of mass 50kg and radius 0.4 m is rotating with an angular velocity of 10rads−1 about its own axis, which is vertical. Two uniform circular rings, each of mass 6.25 kg and radius 0.2 m, are gently placed symmetrically on the disc in such a manner that they are touching each other along the axis of the disc and are horizontal. Assume that the friction is large enough such that the rings are at rest relative to the disc and the system rotates about the original axis. The new angular velocity (∈reds−1) of the system is
View SolutionA solid cylinder of mass 2 kg and radius 0.2m is rotating about its own axis without friction with angular velocity 3 rads/s. A particle of mass 0.5 kg and moving with a velocity 5 m/s strikes the cylinder and sticks to it as shown in figure. The angular momentum of the cylinder before collision will be?
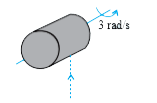
View SolutionA soild cylinder of mass 2 kg and radius 0.2 m is rotating about its owm axis without friction with an angular velocity of 3 rad s−1 . Angular momentum of the cylinder is
View SolutionA ring of radius 0.5 m and mass 10 kg is rotating about its diameter with angular velocity of 20rads−1. Its kinetic energy is
View SolutionA disc of mass 4.8 kg and radius 1 m is rolling on a horizontal surface without sliding with angular velocity of 600 rotations/min. What is the total kinetic energy of the disc ?
View SolutionAn insect of mass m=3kg is inside a vertical drum of radius 2m that is rotating with an angular velocity of 5rads−1 . The insect doesn't fall off. Then, the minimum coefficient of friction required is
View SolutionA disc is rotating with angular velocity ω. If a child sits on it, what is conserved?
View Solution
