A hemisphere of radius R and mass 4 m is free to slide with its base on a smooth horizontal table . A particle of mass m is placed on the top of the hemisphere . The angular velocity of the particle relative to centre of hemisphere at an angular displacement θ when velocity of hemisphere has become v is
5vRcosθ
2vRcosθ
3vRsinθ
5vRsinθ
To solve the problem, we need to find the angular velocity of the particle of mass m relative to the center of the hemisphere when it has moved to an angle θ and the velocity of the hemisphere is v.
1. Understand the System:
- We have a hemisphere of radius R and mass 4m on a smooth horizontal table.
- A particle of mass m is placed on the top of the hemisphere.
2. Define Displacements:
- The horizontal displacement of the hemisphere is x.
- The vertical displacement of the particle from the top of the hemisphere when it has moved to an angle θ is Rsinθ.
3. Center of Mass Consideration:
- The total mass of the system is 4m+m=5m.
- The center of mass of the system must remain stationary since there are no external horizontal forces acting on it.
4. Set Up the Equation:
- The displacement of the particle with respect to the ground is the sum of the displacement of the particle with respect to the hemisphere and the displacement of the hemisphere:
Displacement of particle=Displacement of hemisphere+Displacement of particle w.r.t hemisphere
- This gives us:
0=5m⋅x+Rsinθ
- Rearranging gives:
5mx=−mRsinθ
- Simplifying (cancelling m):
5x=−Rsinθ
5. Differentiate with Respect to Time:
- We know the velocity of the hemisphere is v, which is dxdt.
- Differentiate both sides of the equation:
5dxdt=−Rcosθdθdt
- Substituting dxdt=v:
5v=−Rcosθdθdt
6. Solve for Angular Velocity:
- Rearranging gives:
dθdt=−5vRcosθ
- The negative sign indicates the direction of rotation, but we are interested in the magnitude:
ω=5vRcosθ
Final Answer:
The angular velocity of the particle relative to the center of the hemisphere at an angular displacement θ when the velocity of the hemisphere is v is:
ω=5vRcosθ
- We have a hemisphere of radius R and mass 4m on a smooth horizontal table.
- A particle of mass m is placed on the top of the hemisphere.
- The horizontal displacement of the hemisphere is x.
- The vertical displacement of the particle from the top of the hemisphere when it has moved to an angle θ is Rsinθ.
- The total mass of the system is 4m+m=5m.
- The center of mass of the system must remain stationary since there are no external horizontal forces acting on it.
- The displacement of the particle with respect to the ground is the sum of the displacement of the particle with respect to the hemisphere and the displacement of the hemisphere:
Displacement of particle=Displacement of hemisphere+Displacement of particle w.r.t hemisphere
- This gives us:
0=5m⋅x+Rsinθ
- Rearranging gives:
5mx=−mRsinθ
- Simplifying (cancelling m):
5x=−Rsinθ
- We know the velocity of the hemisphere is v, which is dxdt.
- Differentiate both sides of the equation:
5dxdt=−Rcosθdθdt
- Substituting dxdt=v:
5v=−Rcosθdθdt
- Rearranging gives:
dθdt=−5vRcosθ
- The negative sign indicates the direction of rotation, but we are interested in the magnitude:
ω=5vRcosθ
The angular velocity of the particle relative to the center of the hemisphere at an angular displacement θ when the velocity of the hemisphere is v is:
ω=5vRcosθ
Topper's Solved these Questions
- CENTRE OF MASS, IMPULSE AND MOMENTUMBOOK - DC PANDEY ENGLISHCHAPTER - CENTRE OF MASS, IMPULSE AND MOMENTUMEXERCISE - More a than one option is Correct
1 Videos
- CENTRE OF MASS, IMPULSE AND MOMENTUMBOOK - DC PANDEY ENGLISHCHAPTER - CENTRE OF MASS, IMPULSE AND MOMENTUMEXERCISE - Comprehension type questions
15 Videos
- CENTRE OF MASS, IMPULSE AND MOMENTUMBOOK - DC PANDEY ENGLISHCHAPTER - CENTRE OF MASS, IMPULSE AND MOMENTUMEXERCISE - Comprehension type questions
15 Videos
- CENTRE OF MASSBOOK - DC PANDEY ENGLISHCHAPTER - CENTRE OF MASSEXERCISE - Medical entrances gallery
27 Videos
- CENTRE OF MASS, LINEAR MOMENTUM AND COLLISIONBOOK - DC PANDEY ENGLISHCHAPTER - CENTRE OF MASS, LINEAR MOMENTUM AND COLLISIONEXERCISE - Level 2 Subjective
21 Videos
Similar Questions
A particle of mass m moves along line PC with velocity v as shown. What is the angular momentum of the particle about O?

View SolutionA particle of mass m is projected with a velocity v at an angle of θ with horizontal. The angular momentum of the particle at the highest point of its trajectory is equal to :
View Solution
Knowledge Check
A particle rests on the top of a hemishpere of radius R. Find the smallest horizontal velocity that must be imparted to the particle if it is yo leave the hemisphere without sliding down it.
A√gRB√2gRC√3gRD√5gR

A uniform solid hemisphere of radius 10 m and mass 64 kg is placed with its curved surface on the smooth horizontal surface and a string AB of length 4 m is attached to point A on its rim as shown in the figure Find the tension in the string if hemisphere is in equilibrium.
View SolutionCentripetal acceleration of a particle of mass m moving in a circle of radius r is 4/r2. What is the angular momentum of the particle ?
View SolutionA satellite of mass m is circulating around the earth with constant angular velocity. If the radius is R0 and mass of earth is M, then the angular momentum about the centre of the earth is
View SolutionA chain of length l and mass m lies of the surface of a smooth hemisphere of radius R>l with one end tied to the top of the hemisphere. Taking base of the hemisphere as reference line, find the gravitational potential energy of the chain.
View SolutionA sphere of mass m falls on a smooth hemisphere of mass M resting with its plane face on smooth horizontal table, so that at the moment of impact, line joining the centres makes an angle with the vertical. The velocity of sphere just before impact is u and e is the coefficient of restitution.
View SolutionA hoop of radius r mass m rotating with an angular velocity ω0 is placed on a rough horizontal surface. The initial velocity of the centre of the hoop is zero. What will be the velocity of the centre of the hoop when it cases to slip ?
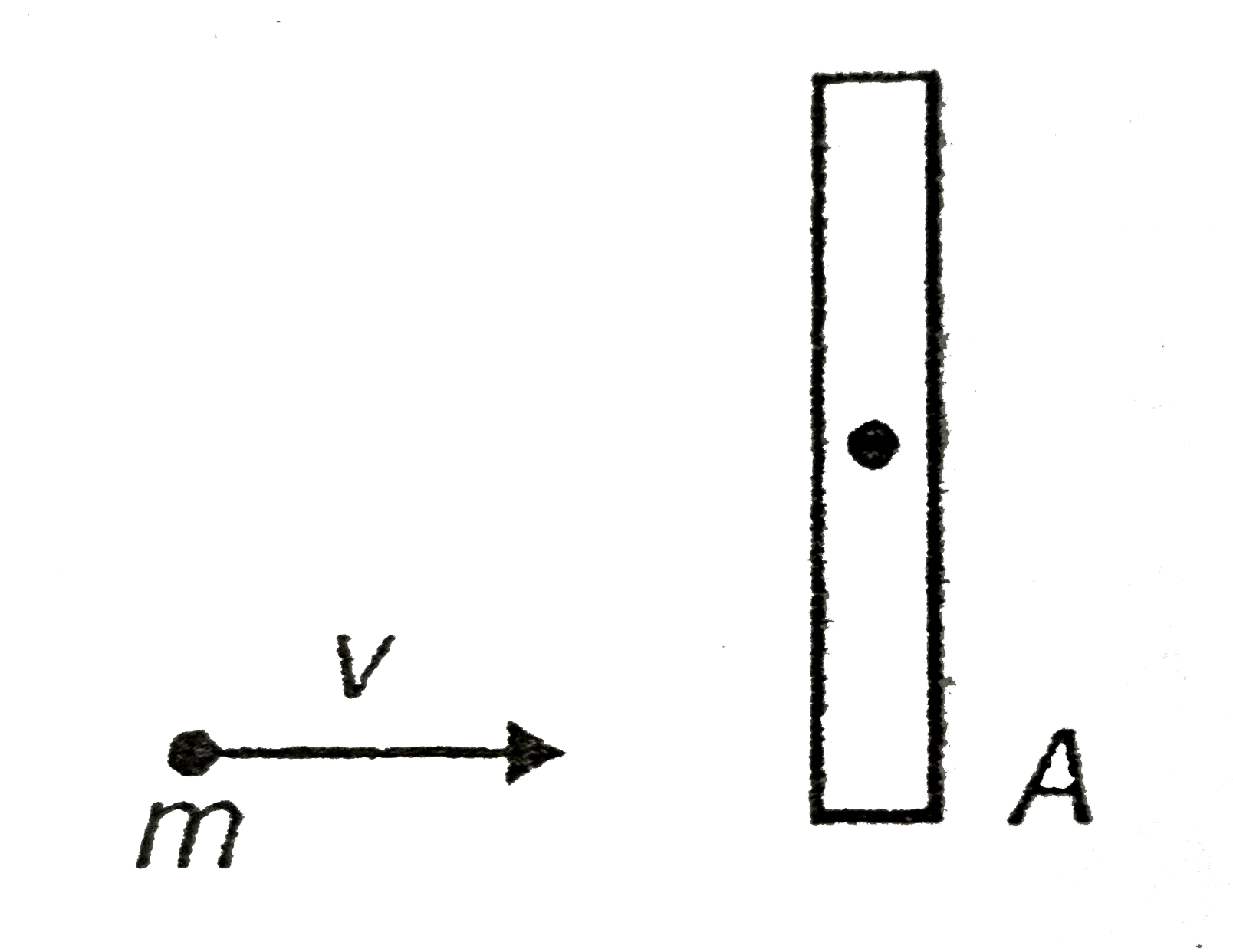
View SolutionA rod of mass m and length l hinged at the centre is placed on a horizontal surface. A bullet of mass m moving with velocity v strikes the end A of the rod and gets embedded in it. The angular velocity with which the systems rotates about its centre of mass after the bullet strikes the rod
View Solution
