A hollow cylinder of mass m and radius r is kept on a smooth horizontal surface. Horizontal force F is applied at a height above the centre. Find the value of h/R so that ring performs pure rolling on the surface.
1
The correct Answer is:A
To solve the problem of finding the value of hr such that a hollow cylinder performs pure rolling on a smooth horizontal surface when a horizontal force F is applied at a height h above the center, we can follow these steps:
1. Understanding the System:
- We have a hollow cylinder (ring) of mass m and radius r on a smooth horizontal surface.
- A horizontal force F is applied at a height h above the center of the cylinder.
2. Conditions for Pure Rolling:
- For pure rolling to occur, the point of contact with the surface must have zero velocity relative to the surface. This means that the linear acceleration a of the center of mass and the angular acceleration α must satisfy the relation:
a=αr
3. Equations of Motion:
- The net force acting on the cylinder in the horizontal direction is given by:
F=ma
- The torque τ about the center of mass due to the force F applied at height h is:
τ=F⋅(h+r)
4. Moment of Inertia:
- The moment of inertia I of a hollow cylinder about its center is:
I=mr2
5. Torque and Angular Acceleration:
- The torque can also be expressed in terms of angular acceleration:
τ=Iα
- Substituting the moment of inertia:
F⋅(h+r)=(mr2)α
6. Substituting for Angular Acceleration:
- From the condition for pure rolling, we have α=ar. Substituting this into the torque equation gives:
F⋅(h+r)=(mr2)(ar)
- This simplifies to:
F⋅(h+r)=mra
7. Relating Force and Acceleration:
- From the net force equation F=ma, we can express a as:
a=Fm
- Substituting this into the torque equation yields:
F⋅(h+r)=mr(Fm)
- This simplifies to:
F⋅(h+r)=Fr
8. Solving for h:
- Dividing both sides by F (assuming F≠0):
h+r=r
- Rearranging gives:
h=r−r=0
- This implies:
h=r
9. Finding the Ratio:
- Therefore, the ratio hr is:
hr=rr=1
Final Answer:
hr=1
- We have a hollow cylinder (ring) of mass m and radius r on a smooth horizontal surface.
- A horizontal force F is applied at a height h above the center of the cylinder.
- For pure rolling to occur, the point of contact with the surface must have zero velocity relative to the surface. This means that the linear acceleration a of the center of mass and the angular acceleration α must satisfy the relation:
a=αr
- The net force acting on the cylinder in the horizontal direction is given by:
F=ma
- The torque τ about the center of mass due to the force F applied at height h is:
τ=F⋅(h+r)
- The moment of inertia I of a hollow cylinder about its center is:
I=mr2
- The torque can also be expressed in terms of angular acceleration:
τ=Iα
- Substituting the moment of inertia:
F⋅(h+r)=(mr2)α
- From the condition for pure rolling, we have α=ar. Substituting this into the torque equation gives:
F⋅(h+r)=(mr2)(ar)
- This simplifies to:
F⋅(h+r)=mra
- From the net force equation F=ma, we can express a as:
a=Fm
- Substituting this into the torque equation yields:
F⋅(h+r)=mr(Fm)
- This simplifies to:
F⋅(h+r)=Fr
- Dividing both sides by F (assuming F≠0):
h+r=r
- Rearranging gives:
h=r−r=0
- This implies:
h=r
- Therefore, the ratio hr is:
hr=rr=1
hr=1
Topper's Solved these Questions
- BOOK - MODERN PUBLICATIONCHAPTER - SYSTEMS OF PARTICLES AND ROTATIONAL MOTIONEXERCISE - Chapter Practice Test (for Board Examination)16videos
SYSTEMS OF PARTICLES AND ROTATIONAL MOTION
- BOOK - MODERN PUBLICATIONCHAPTER - SYSTEMS OF PARTICLES AND ROTATIONAL MOTIONEXERCISE - COMPETITION FILE (OBJECTIVE TYPE QUESTIONS (MATRIX MATCH TYPE QUESTIONS) )1videos
SYSTEMS OF PARTICLES AND ROTATIONAL MOTION
- BOOK - MODERN PUBLICATIONCHAPTER - PHYSICAL WORLD EXERCISE - Revision exercises (Long answer questions)6videos
PHYSICAL WORLD
- BOOK - MODERN PUBLICATIONCHAPTER - THERMAL PROPERTIES OF MATTEREXERCISE - CHAPTER PRACTICE TEST15videos
THERMAL PROPERTIES OF MATTER
Similar Questions
A uniform sphere is placed on a smooth horizontal surface and a horizontal force F is applied on it at a distance h above the surface. The acceleration of the centre :
View SolutionA solid sphere of radius R is resting on a smooth horizontal surface. A constant force F is applied at a height h from the bottom. Vhoose the correct alternative.
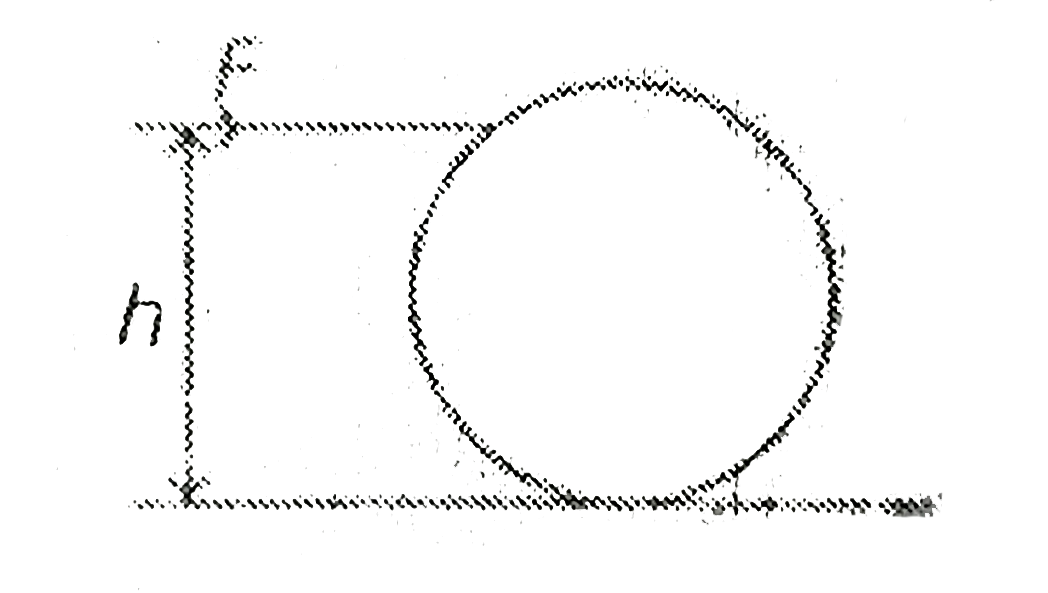 View Solution
View Solution
Knowledge Check
- Question 1 - Select One
A solid sphere of mass M and radius R is placed on a rough horizontal surface. It is stuck by a horizontal cue stick at a height h above the surface. The value of h so that the sphere performs pure rolling motion immediately after it has been stuck is
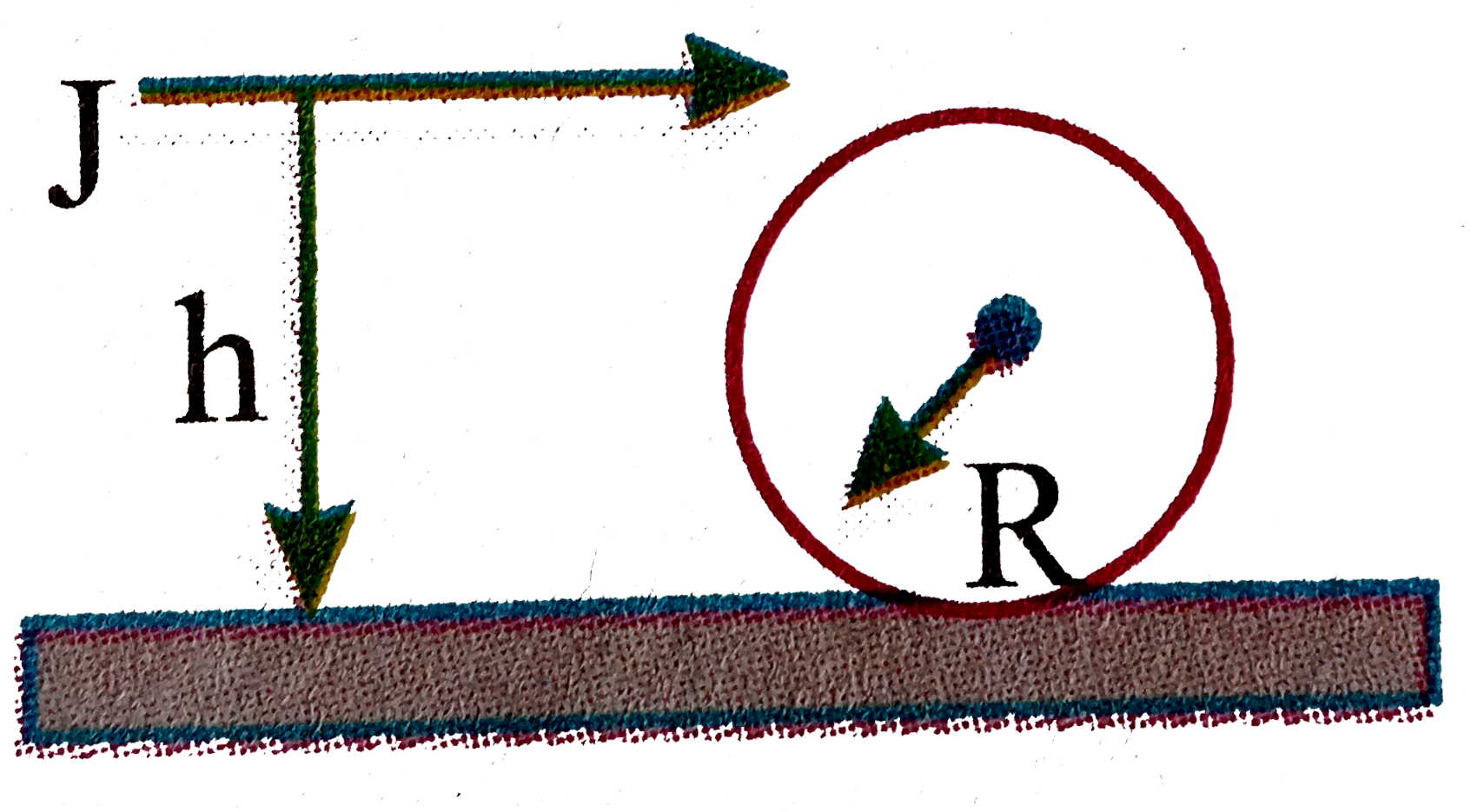 A2R5B5R2C7R5D9R5
A2R5B5R2C7R5D9R5 - Question 2 - Select One

A uniform solid cylinder of mass m and radius R is placed on a rough horizontal surface. A horizontal constant force F is applied at the top point P of the cylinder so that it start pure rolling. The acceleration of the cylinder isAF/3mB2F/3mC4F/3mD5F/3m - Question 3 - Select One
A solid sphere of radius R is placed on a smooth horizontal surface. A horizontal force F is applied at height h from the lowest point. For the maximum acceleration of the centre of mass
Ah=RBh=2RCh=0Dthe acceleration will be same whatever h may be

A solid sphere of mass ma nd radis R is placed on a rough horizontal surface A horizontal force F is applied to sphere at a height h, (0≤h≤R) from centre. If sphere rolls slipping then,View SolutionA solid sphere of mass M and radius R is placed on a smooth horizontal surface. It is given a horizontal impulse J at a height h above the centre of mass and sphere starts rolling then, the value of h and speed of centre of mass are –
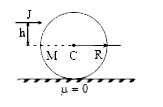 View Solution
View SolutionA uniform solid sphere of mass M and radius R is placed on a smooth horizontal surface. It is given a horizontal impulse J at a height h above the centre of mass and sphere starts rolling. Then the value of h and speed of ccentre of mass are
View SolutionA hollow sphere of mass 2kg is kept on a rough horizontal surface. A force of 10N is applied at the centre of the sphere as shown in the figure. Find the minimum value of μ so that the sphere starts pure rolling. (take g=10ms−2)
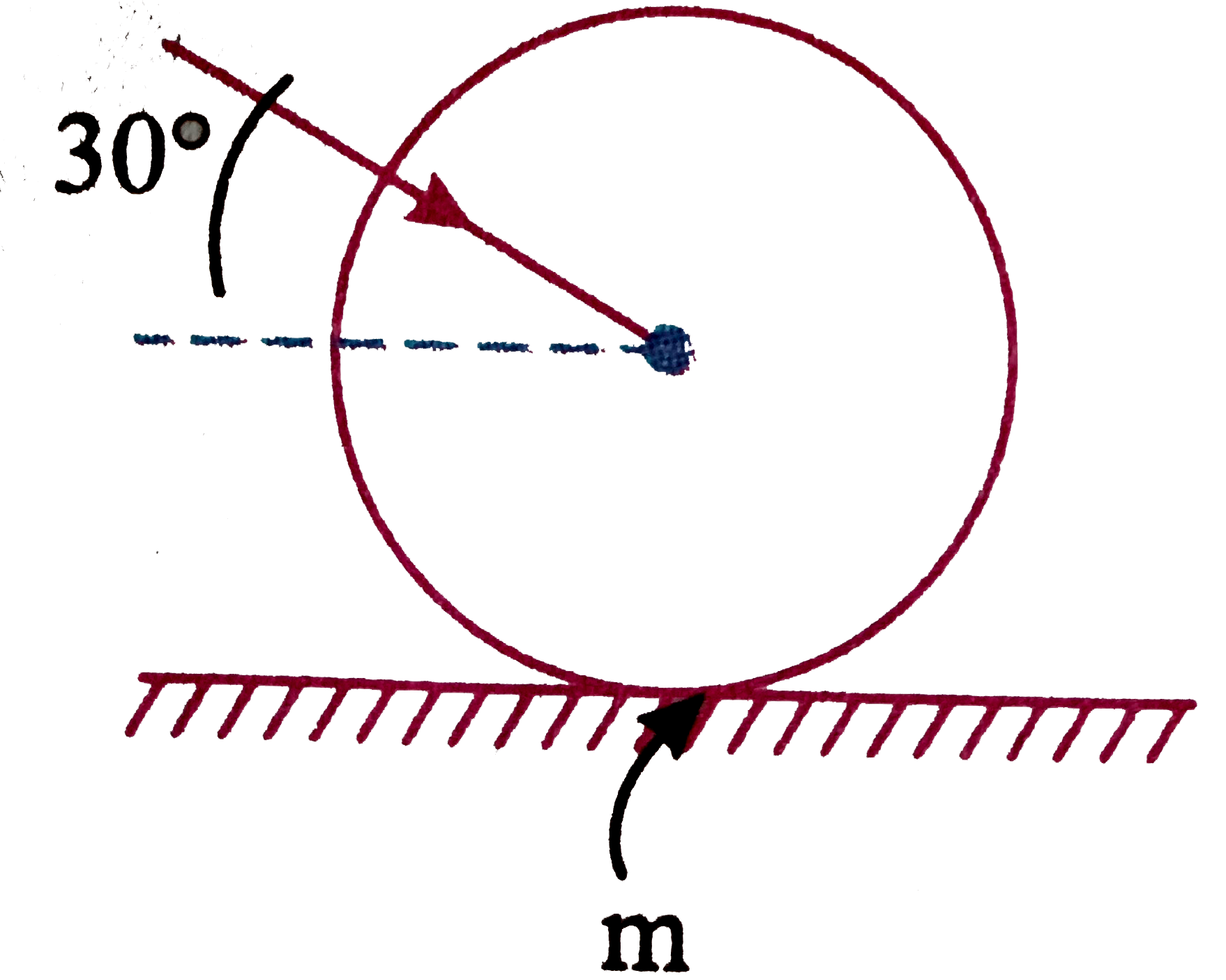 View Solution
View SolutionA loop of mass M and radius R is rolling on a smooth horizontal surface with speed 'v'. It's total kinetic energy is
View Solution

