A ladder of mass 20 kg is resting against a vertical smooth wall. Ladder makes an angle 37° with the wall. Other end of the ladder is resting on a rough horizontal floor. Find normal force and force of friction applied on the ladder by horizontal surface.
To solve the problem of finding the normal force and force of friction applied on the ladder by the horizontal surface, we will follow these steps:
Step 1: Identify the forces acting on the ladder
1. Weight of the ladder (W): This acts downwards at the center of the ladder (point C). The weight can be calculated as:
W=mg=20kg×10m/s2=200N
2. Normal force from the wall (N): This acts horizontally towards the ladder from the smooth wall.
3. Normal force from the ground (N'): This acts vertically upwards from the ground at point D.
4. Frictional force (f): This acts horizontally from the ground at point D, opposing the tendency of the ladder to slide.
Step 2: Draw the Free Body Diagram (FBD)
- Label the points: A (top of the ladder against the wall), B (bottom of the ladder on the ground), C (midpoint of the ladder), and D (bottom of the ladder on the ground).
- The forces acting on the ladder are:
- Weight (W) acting downwards at point C.
- Normal force (N) acting horizontally from the wall at point A.
- Normal force (N') acting upwards from the ground at point D.
- Frictional force (f) acting horizontally to the left at point D.
Step 3: Apply the equilibrium conditions
1. Vertical equilibrium (sum of vertical forces = 0):
N′−W=0⟹N′=W=200N
2. Horizontal equilibrium (sum of horizontal forces = 0):
N−f=0⟹N=f
Step 4: Apply the moment equilibrium condition
- Choose point B (the bottom of the ladder) to take moments about:
- The moments due to the forces are:
- Moment due to normal force from the wall (N):
Moment=N⋅Lcos(37∘)
- Moment due to the weight of the ladder (W):
Moment=W⋅(L2sin(37∘))
- Moment due to normal force from the ground (N'):
Moment=N′⋅Lsin(37∘)
Setting the sum of the moments about point B to zero:
N⋅Lcos(37∘)−W⋅(L2sin(37∘))−N′⋅Lsin(37∘)=0
Step 5: Substitute known values and solve for N
- Substitute W=200N and N′=200N:
N⋅Lcos(37∘)−200⋅(L2sin(37∘))−200⋅Lsin(37∘)=0
- Cancel L from all terms:
Ncos(37∘)−100sin(37∘)−200sin(37∘)=0
Ncos(37∘)=300sin(37∘)
N=300sin(37∘)cos(37∘)=300tan(37∘)
Step 6: Calculate the values
Using tan(37∘)≈0.75:
N=300⋅0.75=225N
Step 7: Find the frictional force
Since N=f:
f=225N
Final Answer
- Normal force N′=200N
- Frictional force f=225N
1. Weight of the ladder (W): This acts downwards at the center of the ladder (point C). The weight can be calculated as:
W=mg=20kg×10m/s2=200N
2. Normal force from the wall (N): This acts horizontally towards the ladder from the smooth wall.
3. Normal force from the ground (N'): This acts vertically upwards from the ground at point D.
4. Frictional force (f): This acts horizontally from the ground at point D, opposing the tendency of the ladder to slide.
- Label the points: A (top of the ladder against the wall), B (bottom of the ladder on the ground), C (midpoint of the ladder), and D (bottom of the ladder on the ground).
- The forces acting on the ladder are:
- Weight (W) acting downwards at point C.
- Normal force (N) acting horizontally from the wall at point A.
- Normal force (N') acting upwards from the ground at point D.
- Frictional force (f) acting horizontally to the left at point D.
1. Vertical equilibrium (sum of vertical forces = 0):
N′−W=0⟹N′=W=200N
N−f=0⟹N=f
- Choose point B (the bottom of the ladder) to take moments about:
- The moments due to the forces are:
- Moment due to normal force from the wall (N):
Moment=N⋅Lcos(37∘)
- Moment due to the weight of the ladder (W):
Moment=W⋅(L2sin(37∘))
- Moment due to normal force from the ground (N'):
Moment=N′⋅Lsin(37∘)
N⋅Lcos(37∘)−W⋅(L2sin(37∘))−N′⋅Lsin(37∘)=0
- Substitute W=200N and N′=200N:
N⋅Lcos(37∘)−200⋅(L2sin(37∘))−200⋅Lsin(37∘)=0
- Cancel L from all terms:
Ncos(37∘)−100sin(37∘)−200sin(37∘)=0
Ncos(37∘)=300sin(37∘)
N=300sin(37∘)cos(37∘)=300tan(37∘)
Using tan(37∘)≈0.75:
N=300⋅0.75=225N
Since N=f:
f=225N
- Normal force N′=200N
- Frictional force f=225N
Topper's Solved these Questions
- BOOK - MODERN PUBLICATIONCHAPTER - SYSTEMS OF PARTICLES AND ROTATIONAL MOTIONEXERCISE - REVISION EXERCISES (Very Short Answer Questions Carrying 1 mark)29videos
SYSTEMS OF PARTICLES AND ROTATIONAL MOTION
- BOOK - MODERN PUBLICATIONCHAPTER - SYSTEMS OF PARTICLES AND ROTATIONAL MOTIONEXERCISE - REVISION EXERCISES (Additional Questions Carrying 1 mark )4videos
SYSTEMS OF PARTICLES AND ROTATIONAL MOTION
- BOOK - MODERN PUBLICATIONCHAPTER - SYSTEMS OF PARTICLES AND ROTATIONAL MOTIONEXERCISE - NCERT FILE (NCERT EXEMPLAR PROBLEMS (SUBJECTIVES QUESTIONS) (Very Short Answer Type Questions))9videos
SYSTEMS OF PARTICLES AND ROTATIONAL MOTION
- BOOK - MODERN PUBLICATIONCHAPTER - PHYSICAL WORLD EXERCISE - Revision exercises (Long answer questions)6videos
PHYSICAL WORLD
- BOOK - MODERN PUBLICATIONCHAPTER - THERMAL PROPERTIES OF MATTEREXERCISE - CHAPTER PRACTICE TEST15videos
THERMAL PROPERTIES OF MATTER
Similar Questions
A uniform ladder of mass 10 kg leans against a smooth vertical wall making an angle of 530 with it. The other end rests on a rough horizontal floor. Find the normal force and the frictional force that the floor exerts on the ladder
View SolutionA ladder 10m long is leaning against a vertical wall, it makes an angle of 60° with the ground.How far is the foot of the ladder from the wall?
View Solution
Knowledge Check
- Question 1 - Select One
A ladder 15 metres long just reaches the top of a vertical wall. If the ladder makes an angle of 60∘ with the wall, find the height of the wall.
A6.5mB7.5mC8.5mD9.5m - Question 2 - Select One
A ladder of mass 10kg is held at reat against a smooth wall on a rough ground as shown in figure. The normal reaction between the wall and the ladder is
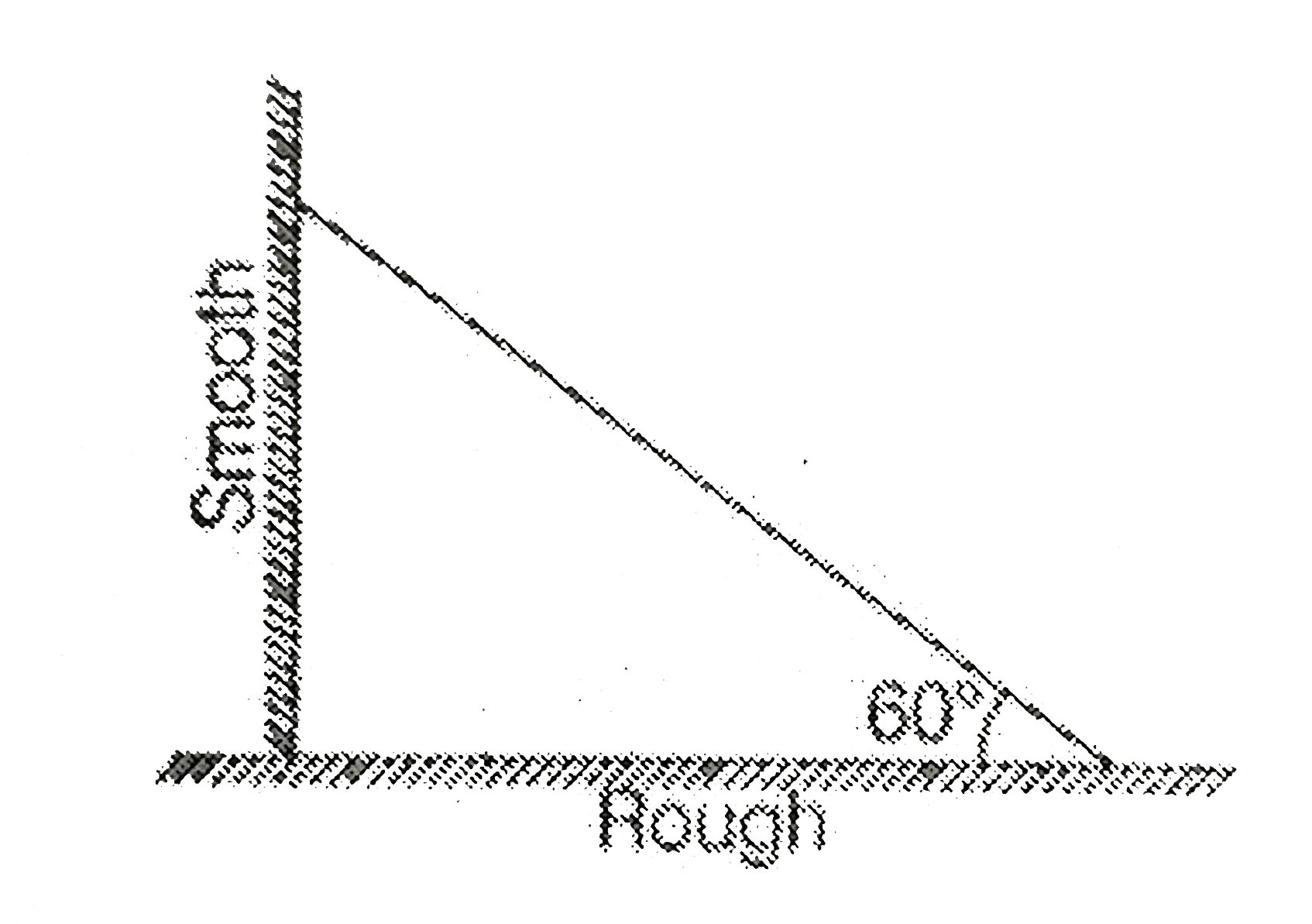 A50NB50√3NC60√3ND100N
A50NB50√3NC60√3ND100N
A ladder makes an angle of 30∘ with a wall. If the foot of the ladder is 5 m away from the wall, find the length of the ladder.
View SolutionA uniform ladder of length 10.0 m and mas 16.0 kg is resting against a vertical smooth wall making an angle of 37∘ with it. An electrian weighing 60.0 kg climbs up the ladder. If the stays on the ladder at a point 8.00 m from the lower end, what will be normal force and the force of friction on the ladder by the ground? What should be the minimum coefficient of friction for the electician to work safely?
View SolutionA ladder 20 m long is placed against a wall so that the foot of the ladder is 10 m from the wall. The angle of inclination of the ladder to the horizontal will be :
View SolutionA ladder leaning against a wall makes an angle of 60o with the ground. If the length of the ladder is 19 m, find the distance of the foot of the ladder from the wall.
View SolutionA ladder of mass 10 kg is resting with its top end touching a vertical wall and the bottom end on the floor. If the angle between the ground and the ladder is 45°, find the horizontal force required at the bottom to prevent the ladder from sliding. Neglect friction and take g=10ms−2.
View SolutionA ladder leaning against a wall makes an angle of 60° with the horizontal. If the foot of the ladder is 2.5 m away from the wall, find the length of the ladder.
View Solution

