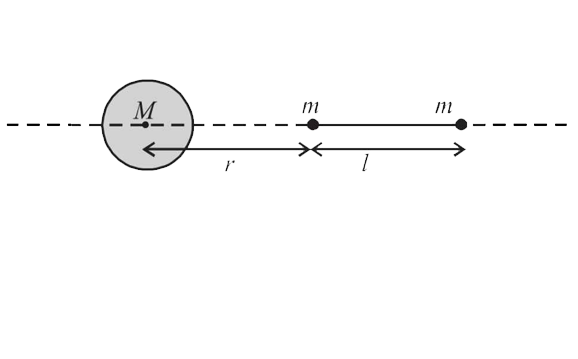
A larger spherical mass M is fixed at one position and two identical point masses m are kept on a line passing through the centre of M. The point masses are connected by rigid massless rod of length l and this assembly is free to move along the line connecting them. All three masses interact only throght their mutual gravitational interaction. When the point mass nearer to M is at a distance r =3l form M, the tensin in the rod is zero for $m = k (\frac{M}{288}) .$ The value of k is

Video Solution

Text Solution

Verified by Experts

The correct Answer is:7

Two particles of masses $m_{1}$ and $m_{2}$ are connected to a rigid massless rod of length r to constitute a dumb bell which is free to move in the plane. The moment of inertia of the dumb bell about an axis perpendicular to the plane passing through the centre of mass is
A $\frac{m_{1} m_{2} r^{2}}{m_{1} + m_{2}}$
B $(m_{1} + m_{2}) r^{2}$
C $\frac{m_{1} m_{2} r^{2}}{m_{1} - m_{2}}$
D $(m_{1} - m_{2}) r^{2}$
Two point masses m and M are separated by a distance L . The distance of the centre of mass of the system from m is
A $L (m / M)$
B $L (M / m)$
C $L (\frac{M}{m + M})$
D $L (\frac{m}{m + M})$
A system consists of two masses connected by a massless rod lies along x-axis. The distance of centre of mass from $O$ is

A $2 m$
B $3 m$
C $5 m$
D $7 m$