A point mass is placed at a distance a from one end of a rod of mass M and length l on the line which passes through the central axis of the rod. The potential energy of the system.

A

$U = - \frac{G M m}{a} {log}_{e} (\frac{a + 1}{a})$

B

$U = - G M m (\frac{1}{a} - \frac{1}{a + 1})$

C

$U = - \frac{G M m}{1} {log}_{e} (\frac{a + 1}{a})$

D

$U = - \frac{G M m}{a}$

Text Solution

Verified by Experts

The correct Answer is:C

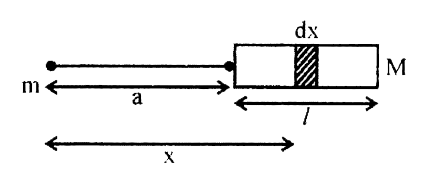
Mass per unit length of the rod $= \frac{M}{1}$
Consider a small element of width dx of the rod at a distance x from m.
P.E. of the element $d U = - \frac{G m (\frac{M}{1}) d x}{x}$
$∴ U = - \frac{G M m}{1} \int_{0}^{a + 1} \frac{d x}{x}$
$= - \frac{G M m}{1} {[{log}_{e} (x)]}_{a}^{a + 1}$
$U = - \frac{G m m}{1} {log}_{e} (\frac{a + l}{a})$

|

Updated on:21/07/2023

Class 12 PHYSICS QUESTION BANK

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
Find the potential energy of the gravitational interaction of a point mass $m$ and a rod of mass $m$ and length $l$ , if they are along a straight line. Point mass is at a distance of a from the end of the rod.

Find the potential energy of the gravitational
A $U = \frac{- G m^{2}}{l} ln (\frac{2 a + l}{2 a - l})$
B $U = \frac{- G m^{2}}{l^{2}} ln (\frac{2 a + l}{2 a - l})$
C $U = \frac{- G m^{2}}{l^{2}} ln (\frac{2 a - l}{2 a + l})$
D $U = \frac{- G m^{2}}{l^{2}} {ln (\frac{2 a - l}{2 a + l})}^{2}$
Question 2 - Select One
Moment of inertia of a rod of mass M and length L about an axis passing through a point midway between centre and end is
A $\frac{M L^{2}}{6}$
B $\frac{M L^{2}}{12}$
C $\frac{7 M L^{2}}{24}$
D $\frac{7 M L^{2}}{48}$
Question 3 - Select One
Moment of inertia of a thin rod of mass m and length l about an axis passing through a point l/4 from one end and perpendicular to the rod is
A $\frac{m l^{2}}{12}$
B $\frac{m l^{2}}{3}$
C $\frac{7 m l^{2}}{48}$
D $\frac{m l^{2}}{9}$

NTA MOCK TESTS-NTA TPC JEE MAIN TEST 48-PHYSICS

Home
Ask Doubt
Profile