A liquid of refractive index 1.6 is contained in the cavity of a glass specimen of refractive index 1.5 as shown in figure. If each of the curve surface has a radius of curvature of 0.20m, the arrangement obehaves as a
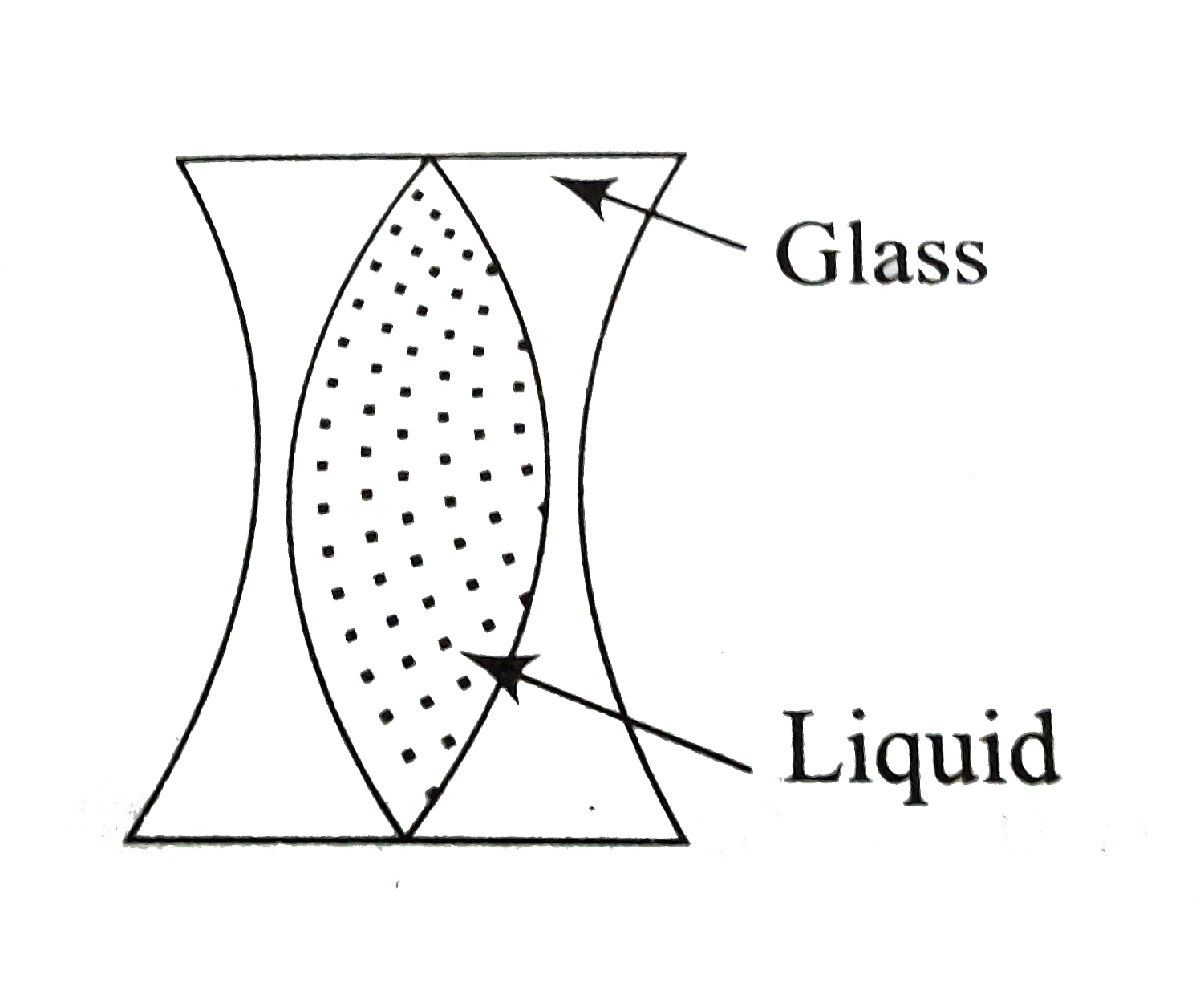
converging lens of focal length 0.25m
diverging lens of focal length 0.25m
diverging lens of focal length 0.17m
converging lens of focal length 0.72m
The correct Answer is:b.
Power of liquid lens
=(1.6−1)(20.20)=610×10=6D
Power of concave lens
=−(1.5−1)=−0.5×10D
Total power of two concave lenses =−10D
Power of system
=−10D+6D=−4D
Focal length =1−4=−0.25m
Topper's Solved these Questions
- GEOMETRICAL OPTICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - GEOMETRICAL OPTICSEXERCISE - Assertion-Reasoninig
2 Videos
- GEOMETRICAL OPTICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - GEOMETRICAL OPTICSEXERCISE - Linked Comprehension
51 Videos
- GEOMETRICAL OPTICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - GEOMETRICAL OPTICSEXERCISE - Subjective
26 Videos
- ELECTRON,PHONTS,PHOTOELECTRIC EFFECT & X-RAYSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ELECTRON,PHONTS,PHOTOELECTRIC EFFECT & X-RAYSEXERCISE - dpp 3.3
15 Videos
- HEATING EFFECT OF CURRENTBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - HEATING EFFECT OF CURRENTEXERCISE - Thermal Power in Resistance Connected in Circuit
27 Videos
Similar Questions
A point object is placed at the center of a glass sphere of radius 6cm and refractive index 1.5. The distance of virtual image from the surface is
View SolutionA concave lens of glass, refractive index 1.5, has both surfaces of same radius of curvature R. On immersion in a medium of refractive index 1.75, it will behave as a
View SolutionRefractive index of diamond with respect to glass is 1.6 and absolute refractive index of glass is 1.5. Find out the absolute refractive index of diamond.
View SolutionA plano convex lens is made of glass of refractive index 1.5. The radius of curvature of its convex surface is R. Its focal length is
View SolutionCalculate the polarizing angle for glass whose refractive index is 1.6.
View SolutionA glass lens of refractive index 1.5 is placed in a trough of liquid. What must be the refractive index of the liquid in order to mark the lens disappear?
View SolutionA plano-concave lens is made of glass of refractive index 1.5 and the radius of curvature of its curved face is 100 cm. What is the power of the lens?
View SolutionA lens is made of flint glass (refractive index =1.5). When the lens is immersed in a liquid of refractive index 1.25 , the focal length:
View SolutionThe adjacent figure shows a thin plano-convex lens of refractive index μ1 and a thin plano-concave lens of refractive index μ2, both having same radius of curvature R of their curved surfaces. The thin lens of refractive index μ3 has radius of curvature R of both its surfaces. This lens is so placed in between the plano-convex and plano-concave lenses that the plane surfaces are parallel to each other.
The focal length of the combination is

View SolutionA double convex lens of refractive index m1 is immersed in a liquid of refractive index m2. This lens will act as:
View Solution
