Question

A long straight wire of a circular cross-section (radius r) carries a steady current I. The current is distributed uniformly across the cross-section. Calculare the magnetic field at a point (a) outside the wire , (b) inside the wire. Draw a graph showing variation of magnetic field.

Explanation

(a) Consider a long straight thick wire of a circular cross-section of radius r carrying a steady current I which is distributed uniformly across the cross-section. For a point `P_1` situated at a normal distance R from central line of wire (where R > r) , consider a circular loop, as shown in fig. as the amperian loop. Then , we have
`oint vecB . vecdi = ont B dl - B oint dl - B. 2pi R = mu_0 I`
`implies B = (mu_0 I)/(2 pi R)`

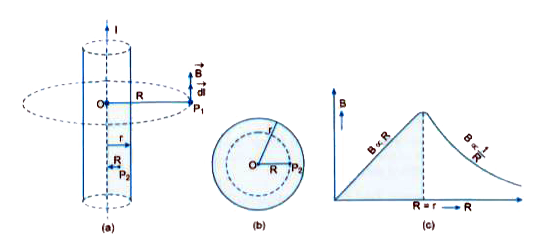
(b) For a point `P_2` situated at a distance R, where R < r, consider a circular amperian loop as shown in fig. Then
`oint vecB . vecd l = oint B dl = B oint dl = B . 2 pi R`
But now the current enclosed is only `I_e = I ((pi R^2)/(pi r^2)) = (I R^2)/(r^2)`. Hence, as per Ampere.s circuital law :
`B . 2 pi r = mu_0 I_e = mu_0 (I R^2)/(r^2) implies B = (mu_0 I R)/(2 pi r^2)`
Moreover field at the surface of wire `(R = r)` will be `B = (mu_0 I)/(2 pi r)`, which is maximum, Variation of magnetic field B with distance R from the axis of thick wire is shown in fig.