A long vertical wire carrying a current of 10A in the upward direction is placed in a region where a horizontal magnetic field of magnitude(2.0×10−3) T exists from south to north. Find the point where the resultant magnetic field is zero.
To solve the problem of finding the point where the resultant magnetic field is zero due to a long vertical wire carrying a current and an external magnetic field, we can follow these steps:
Step 1: Understand the Magnetic Fields
We have two magnetic fields to consider:
1. The magnetic field due to the long vertical wire (B1).
2. The external horizontal magnetic field (B2) directed from south to north.
Step 2: Determine the Direction of Magnetic Fields
- The magnetic field around a straight current-carrying wire is given by the right-hand rule. For an upward current, the magnetic field circles the wire in a counter-clockwise direction.
- At a point to the east of the wire, the magnetic field (B1) will point into the page (or towards you).
- The external magnetic field (B2) is directed from south to north.
Step 3: Set Up the Equation for Zero Resultant Magnetic Field
For the resultant magnetic field to be zero, the magnitudes of B1 and B2 must be equal:
B1=B2
Step 4: Calculate B1
The magnetic field (B1) at a distance d from a long straight wire carrying current I is given by:
B1=μ0I2πd
Where:
- μ0 is the permeability of free space (4π×10−7T m/A).
- I=10A (the current in the wire).
Step 5: Calculate B2
The external magnetic field (B2) is given as:
B2=2.0×10−3T
Step 6: Set the Equations Equal
Setting B1 equal to B2 gives:
μ0I2πd=2.0×10−3
Step 7: Substitute Known Values
Substituting the known values into the equation:
(4π×10−7)(10)2πd=2.0×10−3
Step 8: Simplify the Equation
Cancel out π:
(4×10−7×10)2d=2.0×10−3
4×10−62d=2.0×10−3
2×10−6d=2.0×10−3
Step 9: Solve for d
Cross-multiplying gives:
2×10−6=2.0×10−3d
d=2×10−62.0×10−3
d=1×10−3m
d=0.001m=1mm
Conclusion
The point where the resultant magnetic field is zero is located at a distance of 1 mm from the wire.
---
We have two magnetic fields to consider:
1. The magnetic field due to the long vertical wire (B1).
2. The external horizontal magnetic field (B2) directed from south to north.
- The magnetic field around a straight current-carrying wire is given by the right-hand rule. For an upward current, the magnetic field circles the wire in a counter-clockwise direction.
- At a point to the east of the wire, the magnetic field (B1) will point into the page (or towards you).
- The external magnetic field (B2) is directed from south to north.
For the resultant magnetic field to be zero, the magnitudes of B1 and B2 must be equal:
B1=B2
The magnetic field (B1) at a distance d from a long straight wire carrying current I is given by:
B1=μ0I2πd
Where:
- μ0 is the permeability of free space (4π×10−7T m/A).
- I=10A (the current in the wire).
The external magnetic field (B2) is given as:
B2=2.0×10−3T
Setting B1 equal to B2 gives:
μ0I2πd=2.0×10−3
Substituting the known values into the equation:
(4π×10−7)(10)2πd=2.0×10−3
Cancel out π:
(4×10−7×10)2d=2.0×10−3
4×10−62d=2.0×10−3
2×10−6d=2.0×10−3
Cross-multiplying gives:
2×10−6=2.0×10−3d
d=2×10−62.0×10−3
d=1×10−3m
d=0.001m=1mm
The point where the resultant magnetic field is zero is located at a distance of 1 mm from the wire.
Topper's Solved these Questions
- MAGNETIC FIELD DUE TO CURRENTBOOK - HC VERMACHAPTER - MAGNETIC FIELD DUE TO CURRENTEXERCISE - Objective 2
7 Videos
- MAGNETIC FIELDBOOK - HC VERMACHAPTER - MAGNETIC FIELDEXERCISE - Exercises
61 Videos
- MAGNETIC PROPERTIES OF MATTERBOOK - HC VERMACHAPTER - MAGNETIC PROPERTIES OF MATTEREXERCISE - Exercises
9 Videos
Similar Questions
A long, vertical wire carrying a current of 10A in the upward direction is placed in a region where a horizontal magnetic field of magnitude 2.0×10−3T exist from south to north. Find the point where the resultant magnetic field is zero.
View SolutionA long, straight wire carrying a current of 30 A is placed in an external uniform magnetic field of 4.0×10−4 T exists from south to north. Find at a point 2.0 away from the wire.
View Solution
Knowledge Check
A vertical wire carrying a current in the upward direction is placed in horizontal magnetic field directed towards north. The wire will experience a force directed towards
ANorthBSouthCEastDWestA long straight wire carrying current of 30A is placed in an external unifrom magnetic field of induction 4×104T. The magnetic field is acting parallel to the direction of current. The maggnetic of the resultant magnetic inuduction in tesla at a point 2.0cm away form the wire is
A10−4B3×10−4C5×10−4D6×10−4A long straight wire carrying a current of 30 A is placed in an external unifom magnetic field of intensity 4×10−4 T. The magnetic field is acting parallel to the direction of current. The magnitude of the resultant magnetic induction in tesla at a point 2.0 cm away from the wire is (μ0=4π×10−7Hm−1)
A10−4B3×10−4C5×10−4D6×10−4
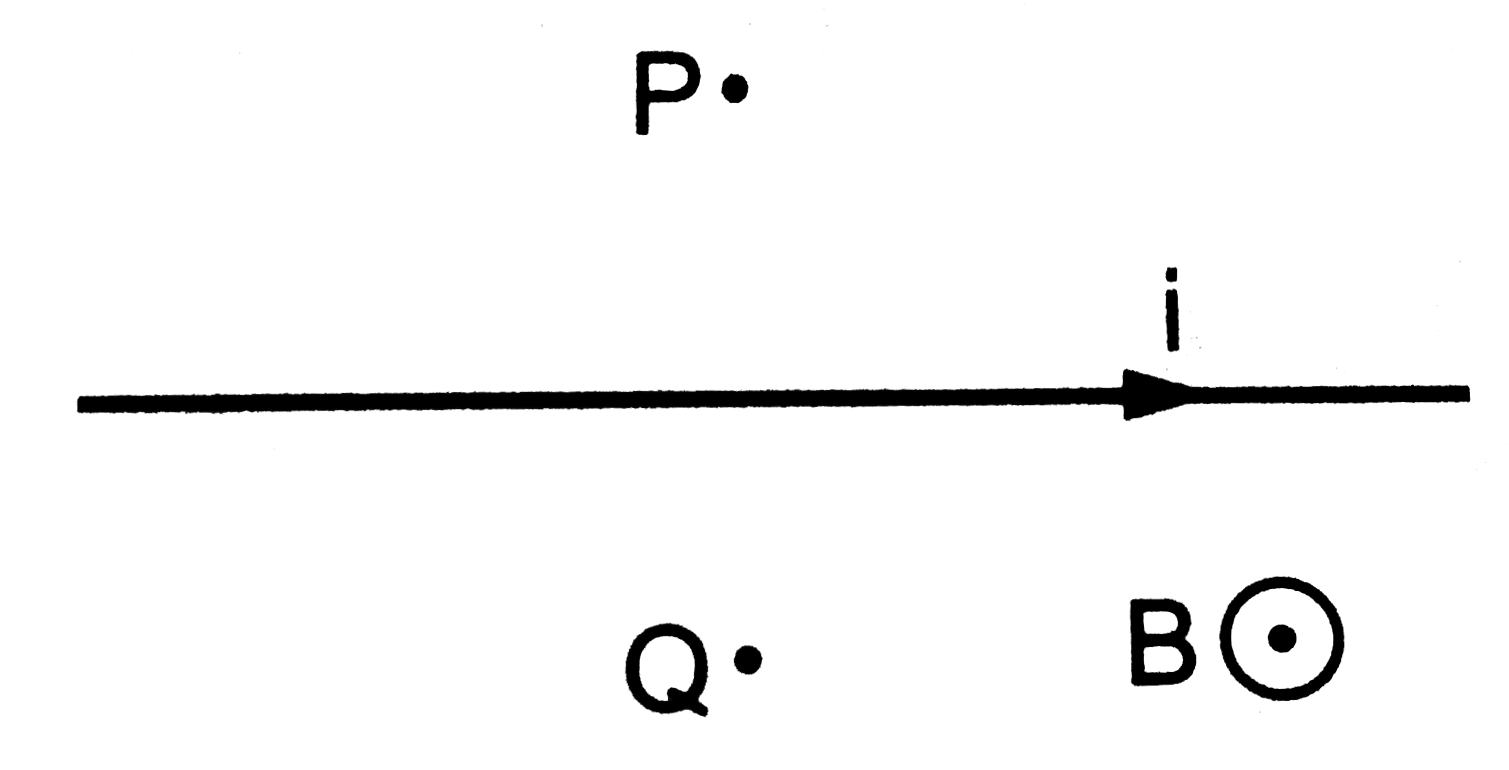
A long, straight wire carrying a current of 1.0 A is placed horizontally in a uniform magnetic field B= (1.0×10−5) T pointing vertically upward. Find the magnitude of the resultant magnetic field at the points P and Q, both situated at a distance of 2.0 cm from the wire in the same horizontal plane.
View SolutionAn alpha particle is projected vertically upward a speed of 3.0×104kms−1 in a region where a magnetic field of magnitude 1.0 T exists in the direction south to north. Find the magnetic force that acts on the a particle.
View SolutionThe horizontal component of the earth's magnetic field is 3.6×10−5T where the dip is 60∘. Find the magnitude of the earth's magnetic field.
View SolutionA long straight wire carrying current of 30A is placed in an external uniform magnetic field of induction 4×104T. The magnetic field is acting parallel to the direction of current. The magnitude of the resultant magnetic inuduction in tesla at a point 2.0cm away form the wire is
View SolutionA long vertical wire carriers a current of 10 amperes flowing upwards through it at place where the horizontal component of the earth's magnetic induction is 0.3 gauss. Then the total magnetic induction at a point 5cm from the wire due magnetic north of the wire is
View Solution
