A mass m = 50 g is dropped on a vertical spring of spring constant 500 N/m from a height h= 10 cm as shown in figure (18-E14). The mass sticks to the spring and executes simple harmonic oscillations after that. A concave mirror of focal length 12 cm facing the mass is fixed with its principal axis coinciding with the line of motion of the mass, its pole being at a distance of 30 cm from the free end of the spring. Find the length in which the image of the mass oscillates.
The correct Answer is:A, B, C
To solve the problem, we will follow these steps:
Step 1: Convert the mass and height to SI units
Given:
- Mass m=50g=50×10−3kg=0.05kg
- Height h=10cm=10×10−2m=0.1m
Step 2: Calculate the compression of the spring when the mass sticks to it
Using the equilibrium condition when the mass sticks to the spring:
mg=kx
Where:
- g=10m/s2 (acceleration due to gravity)
- k=500N/m (spring constant)
- x is the compression of the spring.
Substituting the values:
0.05×10=500×x
0.5=500x
x=0.5500=0.001m=0.1cm
Step 3: Determine the mean position of the mass
The mean position of the mass after it sticks to the spring is:
Mean Position=Distance from the pole+Compression
Given that the distance from the pole of the mirror to the free end of the spring is 30cm:
Mean Position=30cm+0.1cm=30.1cm
Step 4: Apply the work-energy theorem to find maximum compression
Using the work-energy theorem:
mg(h+δ)−12kδ2=0
Where δ is the maximum compression. Rearranging gives:
mg(h+δ)=12kδ2
Substituting the known values:
0.05×10×(0.1+δ)=12×500×δ2
0.5(0.1+δ)=250δ2
0.05+0.5δ=250δ2
Rearranging gives:
250δ2−0.5δ−0.05=0
Step 5: Solve the quadratic equation
Using the quadratic formula δ=−b±√b2−4ac2a:
Where a=250,b=−0.5,c=−0.05:
δ=−(−0.5)±√(−0.5)2−4×250×(−0.05)2×250
=0.5±√0.25+50500
=0.5±√50.25500
Calculating √50.25≈7.07:
δ=0.5±7.07500
Taking the positive root:
δ≈7.57500≈0.01514m=1.514cm
Step 6: Calculate the extremities of oscillation
The maximum compression gives the first extreme position:
First Extreme Position=30.1cm+1.514cm=31.614cm
The amplitude is:
Amplitude=1.514cm
The second extreme position (upward):
Second Extreme Position=30.1cm−1.514cm=28.586cm
Step 7: Use the mirror formula to find the image positions
Using the mirror formula:
1f=1v+1u
Where f=−12cm (focal length of the concave mirror), and u is the object distance (negative for real objects).
For the first extreme position u=−31.614cm:
1v=1−12−1−31.614
Calculating gives:
1v=−112+131.614≈−0.08333+0.03164≈−0.05169
Thus,
v≈−19.38cm
For the second extreme position u=−28.586cm:
1v=1−12−1−28.586
Calculating gives:
1v=−0.08333+0.03496≈−0.04837
Thus,
v≈−20.62cm
Step 8: Calculate the range of image oscillation
The range of image oscillation is:
Range=|v1−v2|=|(−19.38)−(−20.62)|=|20.62−19.38|=1.24cm
Final Answer
The length in which the image of the mass oscillates is 1.24 cm.
---
Given:
- Mass m=50g=50×10−3kg=0.05kg
- Height h=10cm=10×10−2m=0.1m
Using the equilibrium condition when the mass sticks to the spring:
mg=kx
Where:
- g=10m/s2 (acceleration due to gravity)
- k=500N/m (spring constant)
- x is the compression of the spring.
0.05×10=500×x
0.5=500x
x=0.5500=0.001m=0.1cm
The mean position of the mass after it sticks to the spring is:
Mean Position=Distance from the pole+Compression
Given that the distance from the pole of the mirror to the free end of the spring is 30cm:
Mean Position=30cm+0.1cm=30.1cm
Using the work-energy theorem:
mg(h+δ)−12kδ2=0
Where δ is the maximum compression. Rearranging gives:
mg(h+δ)=12kδ2
0.05×10×(0.1+δ)=12×500×δ2
0.5(0.1+δ)=250δ2
0.05+0.5δ=250δ2
Rearranging gives:
250δ2−0.5δ−0.05=0
Using the quadratic formula δ=−b±√b2−4ac2a:
Where a=250,b=−0.5,c=−0.05:
δ=−(−0.5)±√(−0.5)2−4×250×(−0.05)2×250
=0.5±√0.25+50500
=0.5±√50.25500
Calculating √50.25≈7.07:
δ=0.5±7.07500
Taking the positive root:
δ≈7.57500≈0.01514m=1.514cm
The maximum compression gives the first extreme position:
First Extreme Position=30.1cm+1.514cm=31.614cm
The amplitude is:
Amplitude=1.514cm
The second extreme position (upward):
Second Extreme Position=30.1cm−1.514cm=28.586cm
Using the mirror formula:
1f=1v+1u
Where f=−12cm (focal length of the concave mirror), and u is the object distance (negative for real objects).
1v=1−12−1−31.614
Calculating gives:
1v=−112+131.614≈−0.08333+0.03164≈−0.05169
Thus,
v≈−19.38cm
1v=1−12−1−28.586
Calculating gives:
1v=−0.08333+0.03496≈−0.04837
Thus,
v≈−20.62cm
The range of image oscillation is:
Range=|v1−v2|=|(−19.38)−(−20.62)|=|20.62−19.38|=1.24cm
The length in which the image of the mass oscillates is 1.24 cm.
Topper's Solved these Questions
- GEOMETRICAL OPTICSBOOK - HC VERMA ENGLISHCHAPTER - GEOMETRICAL OPTICSEXERCISE - EXAMPLE
9 Videos
- GEOMETRICAL OPTICSBOOK - HC VERMA ENGLISHCHAPTER - GEOMETRICAL OPTICSEXERCISE - Question For short Answer
18 Videos
- GEOMETRICAL OPTICSBOOK - HC VERMA ENGLISHCHAPTER - GEOMETRICAL OPTICSEXERCISE - Objective -2
7 Videos
- GAUSS LAWBOOK - HC VERMA ENGLISHCHAPTER - GAUSS LAWEXERCISE - Short Question
7 Videos
- LIGHT WAVESBOOK - HC VERMA ENGLISHCHAPTER - LIGHT WAVESEXERCISE - Question for short Answer
11 Videos
Similar Questions
A mass of 1.5 kg is connected to two identical springs each of force constant 300Nm−1 as shown in the figure. If the mass is displaced from its equilibrium position by 10cm, then the period of oscillation is

View SolutionA block of mass m suspended from a spring of spring constant k. Find the amplitude of S.H.M.
View Solution
Knowledge Check
An object is kept on the principal axis of a concave mirror of focal length 10cm, at a distance of 15 cm from its pole. The image formed by the mirror is:
AVirtual and magnifiedBVirtual and diminishedCReal and magnifiedDReal and diminished
Two identical springs of spring constant k are attached to a block of mass m and to fixed supports as shown in the figure. The time period of oscillation is

View SolutionA block of 200 g mass is dropped from a height of 2 m on to a spring and compress the spring to a distance of 50 cm. The force constant of the spring is
View SolutionA block of mass m is suspended by different springs of force constant shown in figure.

View SolutionA solid ball of mass m is made to fail from a height H on a pan suspended through a spring of spring constant K as shown in figure. If the does not rebound and the pan is massless, then amplitude of oscillation is
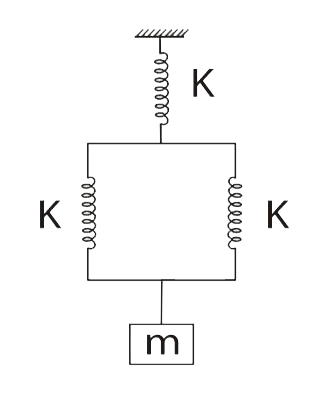
View SolutionA body of mass 'm' hangs from three springs, each of spring constant 'k' as shown in the figure. If the mass is slightly displaced and let go, the system will oscillate with time period–
View SolutionTwo identical springs of spring constant k are attached to a block of mass m and to fixed supports as shown in figure . Show that when the mass is displaced from its equilibrium position on either side, it executes a simple harmonic motion. Find the period of oscillation.

View SolutionA block of mass m hangs from a vertical spring of spring constant k. If it is displaced from its equilibrium position, find the time period of oscillations.
View Solution
