A particle having mass m and charge q is released from the origin in a region in which ele field and magnetic field are given by B=−B0ˆjand E=E0ˆk.
Find the y- component of the velocity and the speed of the particle as a function of it z-coordinate.
The correct Answer is:B
work is done only by electrostatic force. Hence, from work energy theorem.
=12mv2= work done by electrostatic force only =(qE0)z or speed v=√2qE0zm
particle rotates in a plane perpendicular in B. i.e. in xy-plane only. Hence vy=0
Topper's Solved these Questions
- BOOK - DC PANDEYCHAPTER - MAGNETICSEXERCISE - INTRODUCTORY EXERCISE1videos
MAGNETICS
- BOOK - DC PANDEYCHAPTER - MAGNETICSEXERCISE - SUBJECTIVE_TYPE1videos
MAGNETICS
- BOOK - DC PANDEYCHAPTER - MAGNETICSEXERCISE - MCQ_TYPE1videos
MAGNETICS
- BOOK - DC PANDEYCHAPTER - MAGNETIC FIELD AND FORCESEXERCISE - Medical entrance s gallery59videos
MAGNETIC FIELD AND FORCES
- BOOK - DC PANDEYCHAPTER - MAGNETISM AND MATTEREXERCISE - Medical gallery1videos
MAGNETISM AND MATTER
Similar Questions
A particle having mass m and charge q is released from the origin in a region in which electric field and megnetic field are given by →B=−B0ˆj and →E=→E0ˆk. Find the speed of the particle as a function of its z-coordinate.
View SolutionA particle having mass m and charge q is released from the origin in a region in which electric field and magnetic field are ginen by
B=+B0ˆj and →E=+E0ˆi.
Find the speed of the particle as a function of its X-coordinate.View Solution
Knowledge Check
- Question 1 - Select One
A particle of mass m and positive charge q is projected with a speed of v0 in y–direction in the presence of electric and magnetic field are in x–direction. Find the instant of time at which the speed of particle becomes double the initial speed.
A√3mv0qEB√2mv0qECmv0qED√3mv02qE - Question 2 - Select One or More
A particle is released from the origin with a velocity vˆe(i). The electric field in the region is Eˆe(i) and magnetic field is Bˆk. Then
AIf v=0andE=0 then particle will execute a circular path.BIf v=0,E≠0 then particle is positively charged then it executes clockwiseCIf v=0 and E≠0 then the particle will execute a cycloidal motion in the x-y plane.DIf v>0 and E≠0 and the particle is positievely charged and B>0 then the particle wil excute anticlockwise anticlock wise circle as seen from +z direction. - Question 3 - Select One
A particle with mass m and positive charge q released from rest at the origin as shown in figure. There is a uniform electric field E0in +y direction and uniform magnetic field B0 directed out of the page. The path of the particle as shown is called cycloid. The particle always moves in x−y plane. The velocity at any time t after the start is given as

The time for which the y− coordinate of the particle bomes maximum for the first time isAmπqB0B2πmqB0CπmqE0D2πm3qB0
A particle having mass m and charge q is released from the origin in a region in which electric field and magnetic field are given by
→B=−B0→Jand→E=E0→K.
Find the speed of the particle as a function of its z-coordinate.View SolutionTwo particles have equal mass m and electric charge of equal magnitude (q) and opposite sign. The particles are held at rest at co-ordinates (– a, 0) and (a, 0) as shown in the figure. The particles are released simultaneously. Consider only the electrostatic force between the particles and the force applied by the external magnetic field on them.
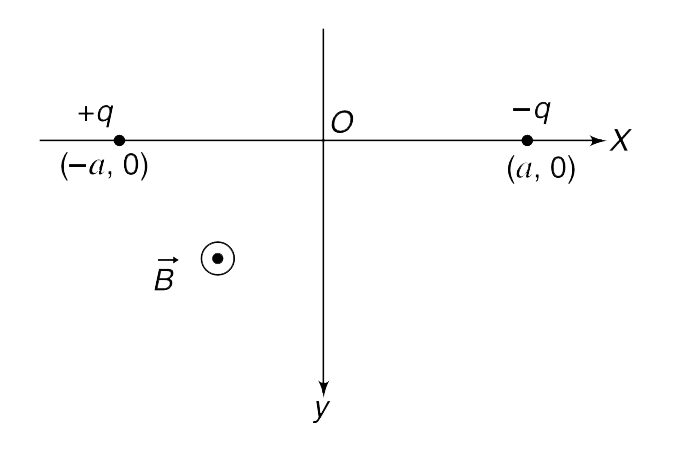
(a) Find the speed of negatively charged particle as function of its x co-ordinate.
(b) Find the y component of velocity of the negative particle as a function of its x co-ordinate.View SolutionA particle of charge q and mass m released from origin with velocity →v=v0ˆi into a region of uniform electric and magnetic fields parallel to y-axis. i.e., →E=E0ˆjand→B=B0ˆj. Find out the position of the particle as a functions of time
Strategy : Here →E∣∣→B
The electric field accelerates the particle in y-direction i.e., component of velocity goes on increasing with acceleration
ay=Fym=Fem=qE0m
The magnetic field rotates the particle in a circle in x-z plane (perpendicular to magnetic field) The resultant path of the particle is a helix with increasing pitch.
Velocity of the particle at time t would be
→v(t)=vxˆi+vyˆj+vzˆkView SolutionA particle of specific charge α is projected from origin with velocity v=v0ˆi−v0ˆk in a uniform magnetic field B=−B0ˆk. Find time dependence of velocity and position of the particle.
View SolutionA particle with mass m and positive charge q released from rest at the origin as shown in figure. There is a uniform electric field E0in +y direction and uniform magnetic field B0 directed out of the page. The path of the particle as shown is called cycloid. The particle always moves in x−y plane. The velocity at any time t after the start is given as

Speed of the particle at a general point P(x,y) is given byView Solution

