A particle I projected at an angle of elevation $α$ after time $t$ it makes an angle $β$ with horizontal. The velocity of projection is

A

$\frac{2 g t cos β}{sin (α - β)}$

B

$\frac{g t cos β}{sin (α - β)}$

C

$\frac{g t}{2 sin (α - β)}$

D

$\frac{4 g t}{sin (α - β)}$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:B

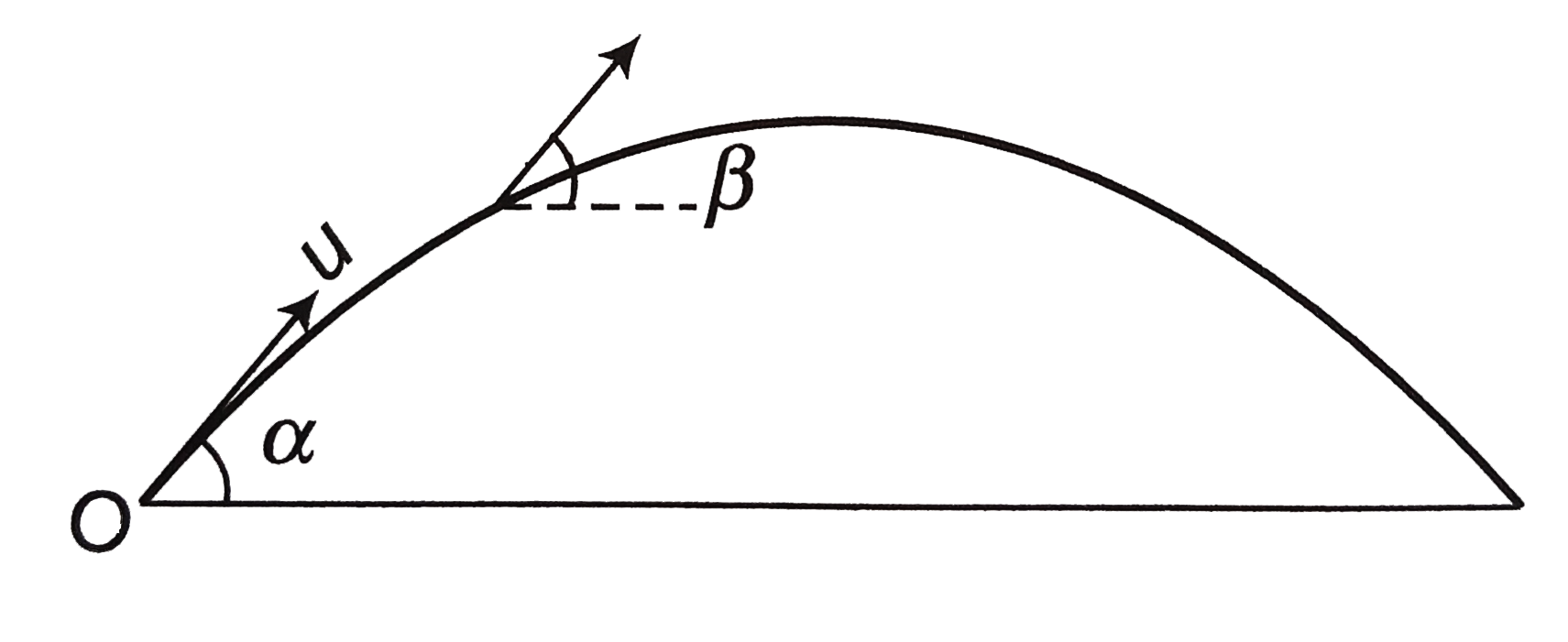
$tan β = \frac{u sin α - g t}{u cos θ}$
$\frac{sin β}{cos β} = \frac{u sin α - g t}{u cos α}$
$u cos α sin β = u sin α cos β β - g t cos β$
$g t cos β = u sin (α - β)$
$u = \frac{g t cos β}{sin (α - β)}$

|

Updated on:21/07/2023

Class 11 PHYSICS PROJECTILE MOTION

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
A particle is projected from the ground with an initial speed of v at an angle $θ$ with horizontal. The average velocity of the particle between its point of projection and highest point of trajectroy is :
A $\frac{v}{2} \sqrt{1 + 2 {cos}^{2} θ}$
B $\frac{v}{2} \sqrt{1 + 2 {cos}^{2} θ}$
C $\frac{v}{2} \sqrt{1 + 3 {cos}^{2} θ}$
D $v cos θ$
Question 2 - Select One
A particle is projected at an angle $α$ with the horizontal from the foot of an inclined plane making an angle $β$ with horizontal . Which of the following expressions holds good if the particle strikes the inclined plane normally?
A $cot β = - tan (α - β)$
B $cot β = 2 tan (α - β)$
C $cot α = tan (α - β)$
D $cot α = 2 tan (α - β)$
Question 3 - Select One
A particle is projected at an angle with horizontal. If $T$ is the time of flight and $R$ is a horizontal range, then $θ$ is
A ${cot}^{- 1} (\frac{g T^{2}}{R})$
B ${cot}^{- 1} (\frac{g T^{2}}{2 R})$
C ${tan}^{- 1} (\frac{2 R}{g T^{2}})$
D ${tan}^{- 1} (\frac{g T^{2}}{2 R})$

CP SINGH-MOTION IN A PLANE-Exercises

Home
Ask Doubt
Profile