A particle is moving along a straight line. The displacement of the particle becomes zero in a certain time (t>0). The perticle does not undergo any collision.
The acceleration of the particle may be zero always
The acceleration of the particle may be zero uniform
The velocity of the particle must be zero at some instant
The acceleration of the particle must change its direction
The correct Answer is:B, C
To solve the problem, we need to analyze the motion of a particle that returns to its original position after some time t>0 without any collision. This implies that the displacement of the particle is zero at that time.
1. Understanding Displacement:
Displacement is defined as the change in position of an object. If the displacement of the particle becomes zero after time t, it means that the particle has returned to its starting point.
Hint: Remember that displacement is a vector quantity that considers the initial and final positions of the particle.
2. Analyzing Velocity:
Since the particle returns to its starting position, it must have a point where its velocity becomes zero. This is because, for the particle to change direction and come back, it must stop momentarily.
Hint: Think about how a ball thrown upwards comes to a stop at its highest point before falling back down.
3. Considering Acceleration:
The problem states that the particle does not undergo any collision. Therefore, the motion is influenced by acceleration. If the particle is moving in one direction and then returns, the acceleration must act in the opposite direction to reduce the velocity to zero and then increase it in the opposite direction.
Hint: Recall that acceleration is the rate of change of velocity and can be in the opposite direction to the velocity.
4. Evaluating the Options:
- Option A: "Acceleration of the particle may be 0 always" - This is false. If acceleration is always zero, the particle would not change its velocity and would not return to the starting point.
- Option B: "Acceleration of the particle may be uniform" - This is true. The particle can have a constant acceleration (uniform) while returning to the starting position.
- Option C: "Velocity of the particle must be zero at some instant" - This is true. As discussed, the particle must stop at some point before changing direction.
- Option D: "Acceleration of the particle must change its direction" - This is false. The acceleration can remain constant in one direction while the velocity changes.
5. Conclusion:
Based on the analysis, the correct options are:
- Option B: Acceleration of the particle may be uniform.
- Option C: Velocity of the particle must be zero at some instant.
Therefore, the final answer is that options B and C are correct.
Displacement is defined as the change in position of an object. If the displacement of the particle becomes zero after time t, it means that the particle has returned to its starting point.
Since the particle returns to its starting position, it must have a point where its velocity becomes zero. This is because, for the particle to change direction and come back, it must stop momentarily.
The problem states that the particle does not undergo any collision. Therefore, the motion is influenced by acceleration. If the particle is moving in one direction and then returns, the acceleration must act in the opposite direction to reduce the velocity to zero and then increase it in the opposite direction.
- Option A: "Acceleration of the particle may be 0 always" - This is false. If acceleration is always zero, the particle would not change its velocity and would not return to the starting point.
- Option B: "Acceleration of the particle may be uniform" - This is true. The particle can have a constant acceleration (uniform) while returning to the starting position.
- Option C: "Velocity of the particle must be zero at some instant" - This is true. As discussed, the particle must stop at some point before changing direction.
- Option D: "Acceleration of the particle must change its direction" - This is false. The acceleration can remain constant in one direction while the velocity changes.
Based on the analysis, the correct options are:
- Option B: Acceleration of the particle may be uniform.
- Option C: Velocity of the particle must be zero at some instant.
Topper's Solved these Questions
- KINEMATICSBOOK - DC PANDEY ENGLISHCHAPTER - KINEMATICSEXERCISE - More Than One Correct
6 Videos
- KINEMATICSBOOK - DC PANDEY ENGLISHCHAPTER - KINEMATICSEXERCISE - Comprehension
7 Videos
- KINEMATICSBOOK - DC PANDEY ENGLISHCHAPTER - KINEMATICSEXERCISE - Objective
45 Videos
- GRAVITATIONBOOK - DC PANDEY ENGLISHCHAPTER - GRAVITATIONEXERCISE - (C) Chapter Exercises
45 Videos
- KINEMATICS 1BOOK - DC PANDEY ENGLISHCHAPTER - KINEMATICS 1EXERCISE - INTEGER_TYPE
15 Videos
Similar Questions
Knowledge Check
Acceleration (a)-displacement(s) graph of a particle moving in a straight line is shown here. The initial velocity of the particle is zero. The v-s graph of the particle would be
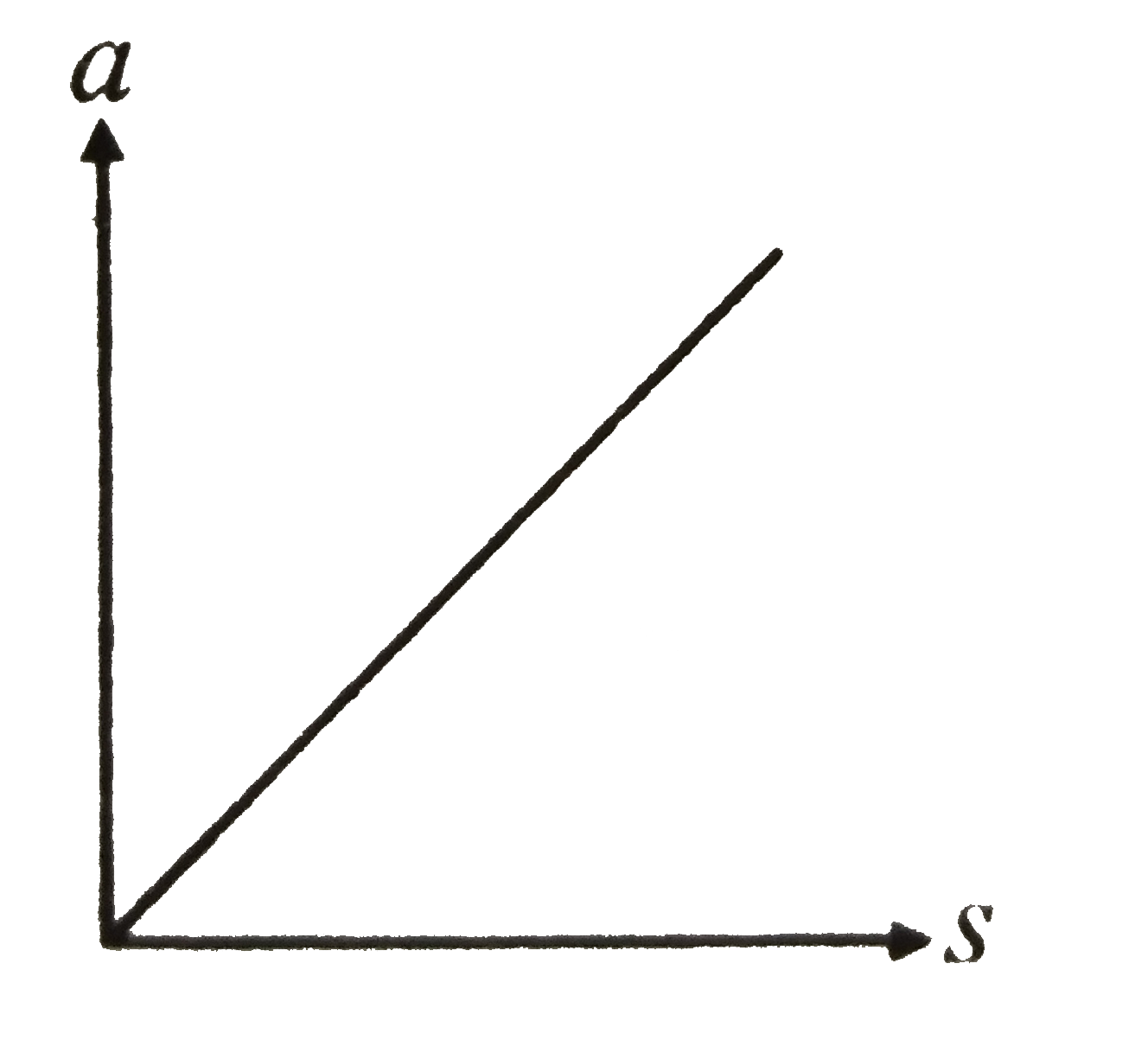 A
A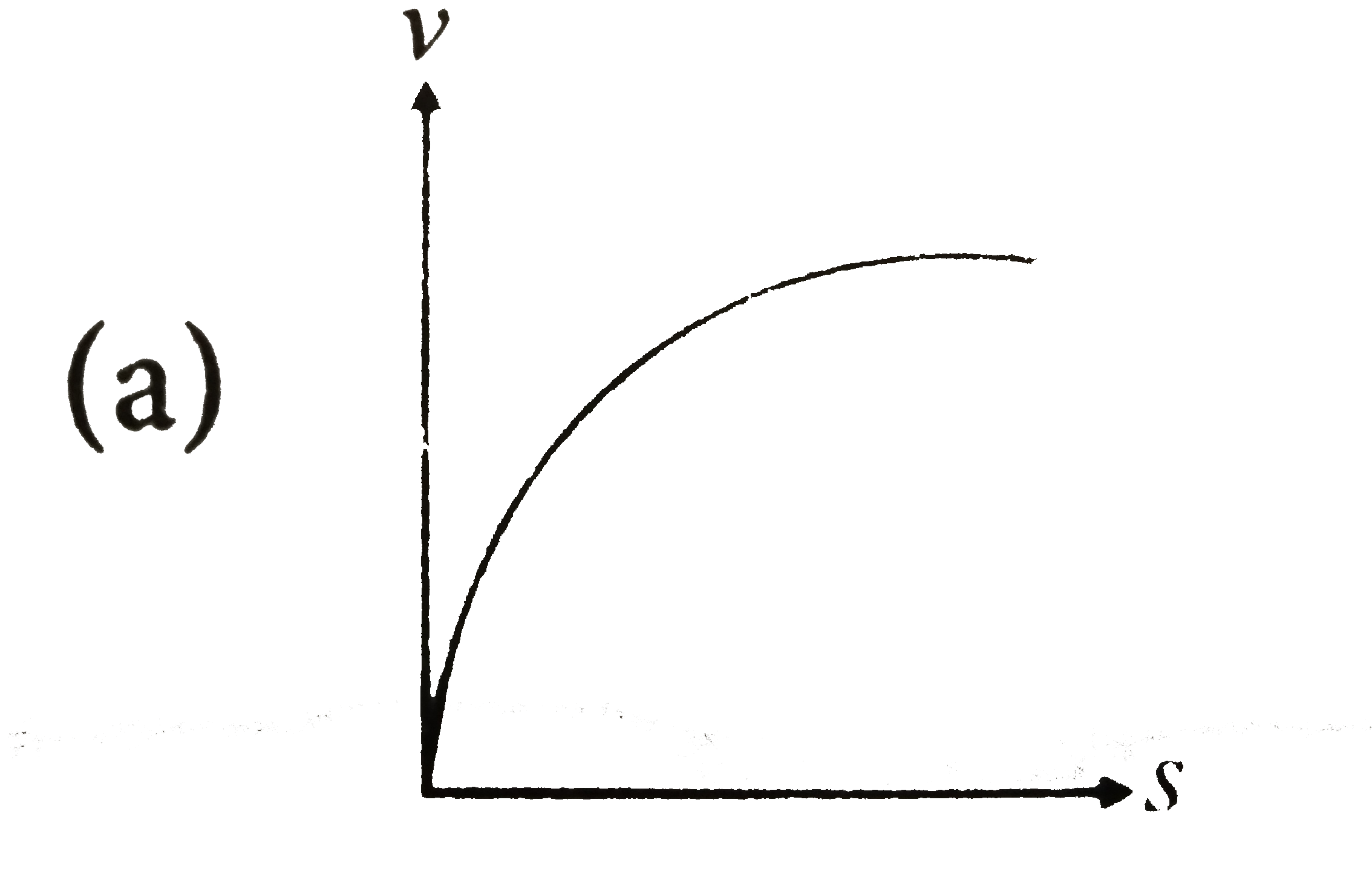 B
B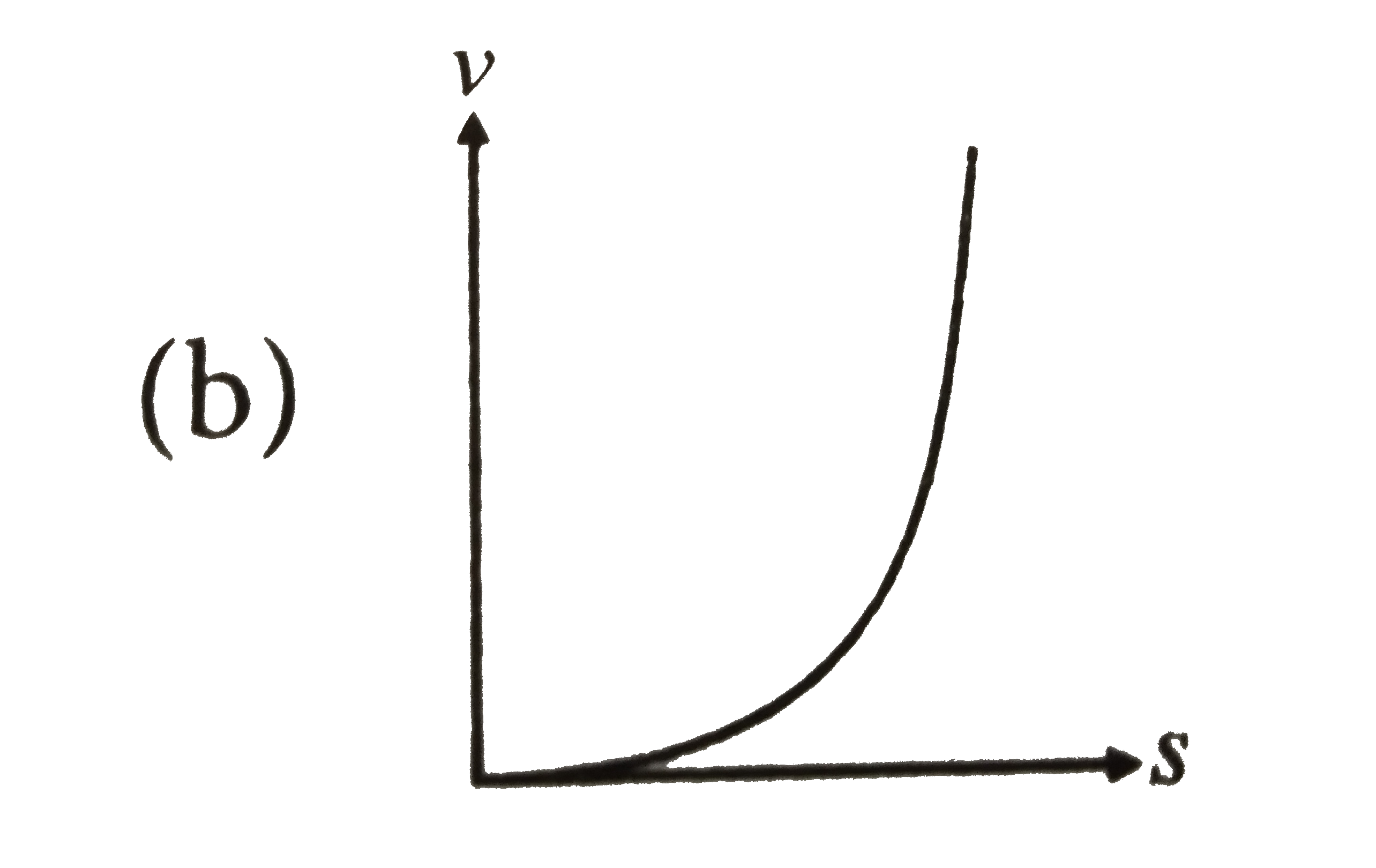 C
C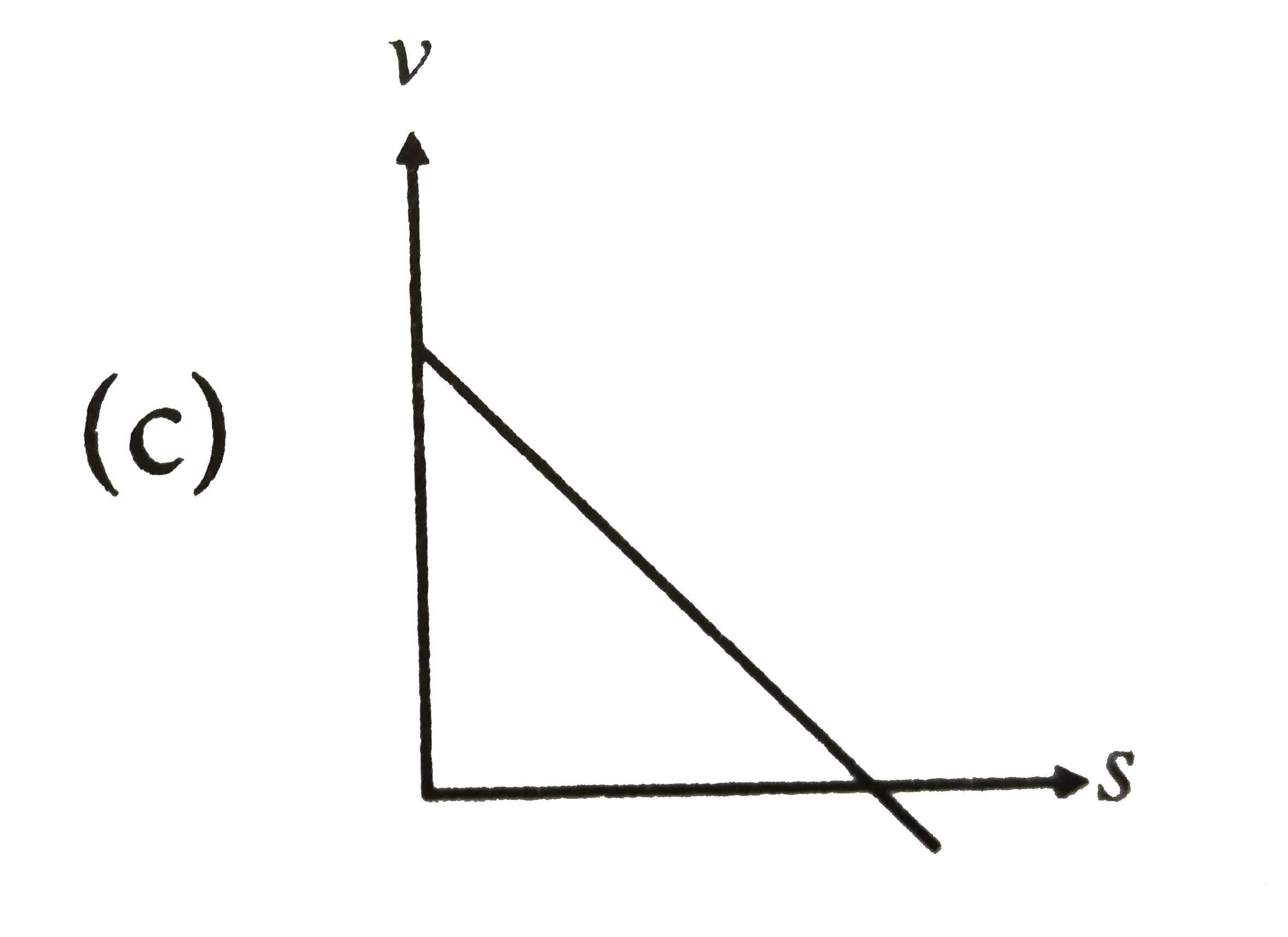 D
D
For a particle moving in a straight line, the displacement of the particle at time t is given by
S=t3−6t2+3t+7
What is the velocity of the particle when its acceleration is zero?
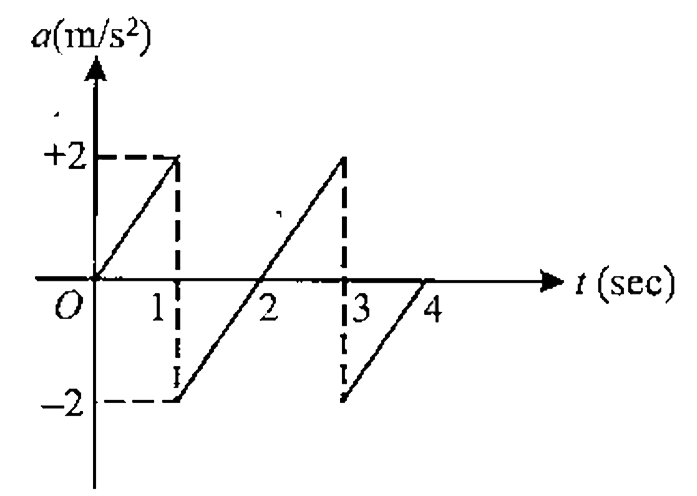
View SolutionAcceleration vs time graph is shown in the figure for a particle moving along a straight line. The particle is initially at rest. Find the time instant(s) when the particle comes to rest?
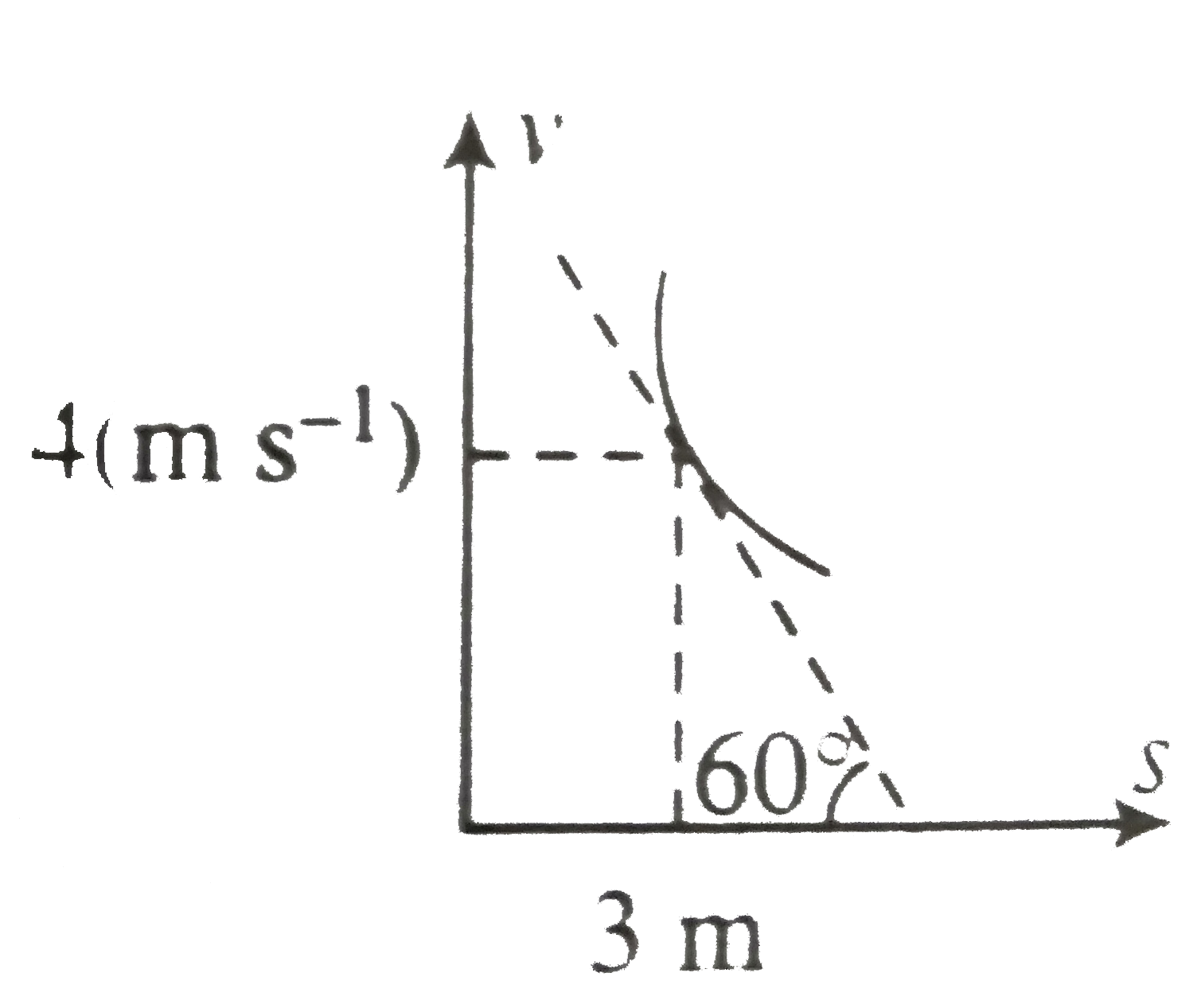
View SolutionA particle is moving along a straight line whose velocity - displacement graph is shown in figure. What is the acceleration when displacement is 3 m?
View SolutionA particle is moving with a constant speed along a straight line path. A force is not required to
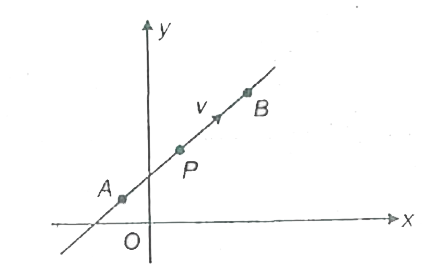
View SolutionA particle P is moving along a straight line as shown in the figure. During the motion of the particle from A to B the angular momentum of the particle about O
View SolutionA particle moves in straight line. Acceleration of particle changes with velocity as showns in graph:
View SolutionThe acceleration (a) of a particle moving in a straight line varies with its displacement (s) as a=2s. The velocity of the particle is zero at zero displacement. Find the corresponding velocity-displacement equation.
View Solution
