A car is travelling on a road. The maximum velocity the car can attain is 24ms−1 and the maximum deceleration is 4ms−2. If car starts from rest and comes to rest after travelling 1032m in the shortest time of 56 s, the maximum acceleration that the car can attain is
6ms−2
1.2ms−2
12ms−2
3.6ms−2
The correct Answer is:B
To solve the problem step by step, we will analyze the motion of the car in three phases: acceleration, constant velocity, and deceleration.
Step 1: Identify the known values
- Maximum velocity (V_max) = 24 m/s
- Maximum deceleration (a_d) = 4 m/s²
- Total distance (s) = 1032 m
- Total time (T) = 56 s
Step 2: Understand the motion phases
The car starts from rest, accelerates to its maximum velocity, travels at that velocity for some time, and then decelerates to come to rest. We can denote:
- Time of acceleration = t_a
- Time of constant velocity = t_c
- Time of deceleration = t_d
The total time is given by:
T=ta+tc+td
Step 3: Calculate the time of deceleration
The time taken to decelerate from maximum velocity to rest can be calculated using:
td=Vmaxad=24m/s4m/s2=6s
Step 4: Set up the equation for total time
Now we can express the total time:
56=ta+tc+6
This simplifies to:
ta+tc=50s(1)
Step 5: Calculate the distance covered during deceleration
The distance covered during deceleration can be calculated using the formula:
sd=12adt2d+Vmaxtd
Substituting the values:
sd=12×4×62+24×6=72+144=216m
Step 6: Set up the equation for total distance
The total distance can be expressed as:
s=sa+sc+sd
Where:
- sa = distance during acceleration
- sc = distance during constant velocity
We know:
s=1032
So:
1032=sa+sc+216
This simplifies to:
sa+sc=816m(2)
Step 7: Calculate distance during acceleration
The distance covered during acceleration can be calculated using the formula:
sa=12aat2a
Where aa is the acceleration we want to find. The distance during constant velocity is:
sc=Vmax⋅tc=24⋅tc
Substituting tc=50−ta into the equation:
sa+24(50−ta)=816
Step 8: Solve for ta
Substituting sa:
12aat2a+24(50−ta)=816
Step 9: Calculate acceleration
From the earlier equations, we can derive aa using the known values. We can express aa in terms of ta, but since we already know the maximum velocity and deceleration, we can find the maximum acceleration directly.
Step 10: Final calculation
From the earlier calculations, we can determine:
ta=20s
And therefore:
aa=Vmaxta=2420=1.2m/s2
Conclusion
The maximum acceleration that the car can attain is:
1.2m/s2
- Maximum velocity (V_max) = 24 m/s
- Maximum deceleration (a_d) = 4 m/s²
- Total distance (s) = 1032 m
- Total time (T) = 56 s
The car starts from rest, accelerates to its maximum velocity, travels at that velocity for some time, and then decelerates to come to rest. We can denote:
- Time of acceleration = t_a
- Time of constant velocity = t_c
- Time of deceleration = t_d
T=ta+tc+td
The time taken to decelerate from maximum velocity to rest can be calculated using:
td=Vmaxad=24m/s4m/s2=6s
Now we can express the total time:
56=ta+tc+6
This simplifies to:
ta+tc=50s(1)
The distance covered during deceleration can be calculated using the formula:
sd=12adt2d+Vmaxtd
Substituting the values:
sd=12×4×62+24×6=72+144=216m
The total distance can be expressed as:
s=sa+sc+sd
Where:
- sa = distance during acceleration
- sc = distance during constant velocity
s=1032
So:
1032=sa+sc+216
This simplifies to:
sa+sc=816m(2)
The distance covered during acceleration can be calculated using the formula:
sa=12aat2a
Where aa is the acceleration we want to find. The distance during constant velocity is:
sc=Vmax⋅tc=24⋅tc
sa+24(50−ta)=816
Substituting sa:
12aat2a+24(50−ta)=816
From the earlier equations, we can derive aa using the known values. We can express aa in terms of ta, but since we already know the maximum velocity and deceleration, we can find the maximum acceleration directly.
From the earlier calculations, we can determine:
ta=20s
And therefore:
aa=Vmaxta=2420=1.2m/s2
The maximum acceleration that the car can attain is:
1.2m/s2
Topper's Solved these Questions
- KINEMATICSBOOK - DC PANDEY ENGLISHCHAPTER - KINEMATICSEXERCISE - More Than One Correct
6 Videos
- KINEMATICSBOOK - DC PANDEY ENGLISHCHAPTER - KINEMATICSEXERCISE - Comprehension
7 Videos
- KINEMATICSBOOK - DC PANDEY ENGLISHCHAPTER - KINEMATICSEXERCISE - Objective
45 Videos
- GRAVITATIONBOOK - DC PANDEY ENGLISHCHAPTER - GRAVITATIONEXERCISE - (C) Chapter Exercises
45 Videos
- KINEMATICS 1BOOK - DC PANDEY ENGLISHCHAPTER - KINEMATICS 1EXERCISE - INTEGER_TYPE
15 Videos
Similar Questions
A car is travelling on a straight road. The maximum velocity the car Can attain is 24ms−1. The maximum acceleration and deceleration it can attain are 1ms−2 and 4ms−2 respectively. The shortest time the car takes from rest to rest in a distance of 200 m is,
View SolutionA car of mass m has an engine which can deliver constant power P.the maximum speed that the car can attain in t seconds in
View SolutionA car is travelling at 36 kmph on a road. If μ=0.5 between the tyres and the road, the minimum turning radius of the car is: (g=10ms−2)
View SolutionA car starting from rest acquires a velocity 180 m s−1 in 0.05 h. Find the acceleration.
View SolutionA car starts from rest and accelerates uniformly to a speed of 180kmh−1 in 10 s. The distance covered by the car in the time interval is
View SolutionA particle starting from rest attains a velocity of 50ms−1 in 5 s. The acceleration of the particle is
View SolutionA car accelerates from rest with 2ms−2 for 2s and then decelerates constantly with 4ms−2 for t0 second to come to rest. The graph for the motion is shown in figure.

a. Find the maximum speed attained by the car.
b. Find the value of t0.
View SolutionA car is to be hoisted by elevator to the fourth floor of a parking garage, which is 14 m above the ground. If the elevator can have maximum acceleration of 0.2m/s2 and maximum deceleration of 0.1m/s2 and can reach a maximum speed of 2.5 m//s, determine the shortest time to make the lift, starting from rest and ending at rest.
View SolutionA car travelling with a speed 125 KMPH along a straight line comes to rest after travelling a distance 245 m.The time taken by the car to come to rest,in second is
View SolutionA car is moving on a straight road. The velocity of the car varies with time as shown in the figure. Initially (at t=0), the car was at x=0, where, x is the position of the car at any time 't'.
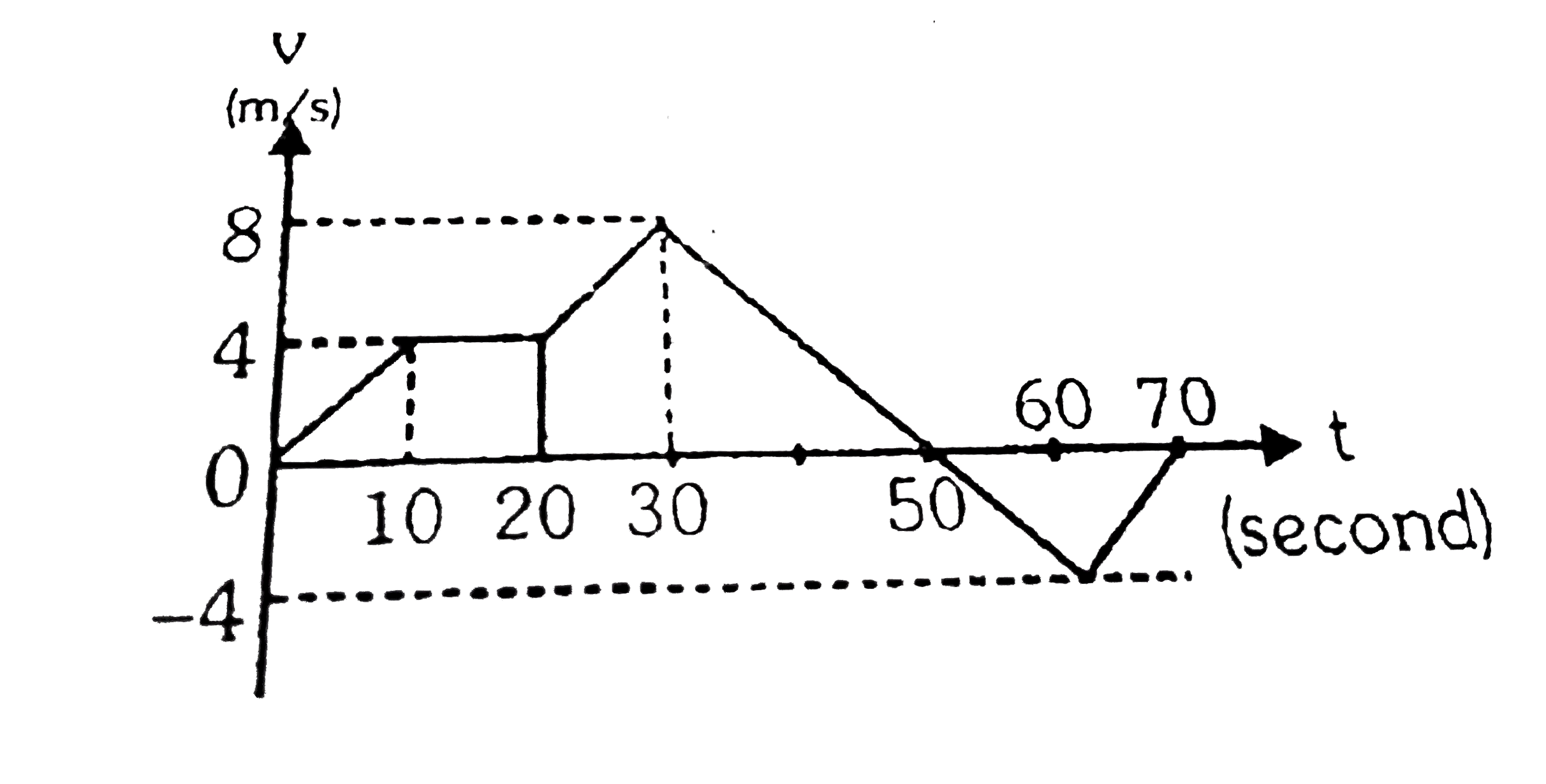
The maximum displacement from the starting position will be :-
View Solution
