A particle is projected from the ground . Point of projection is taken as origin, horizontal direction as X-axis and verticle upward direction ass Y-axis. Equation of trajectory of the partcle is y=αx−βx2. Horizontal range of the projectile is
α+β
α+β
α/β
β/α
The correct Answer is:C
(c): We can understant that y-coordinate of the particle becomes zero when it hits the ground .
y=αx−βx2=0
⇒x=α/β
Thus α/β is the range of projectile . Hence , option (c) is correct .
Topper's Solved these Questions
- BOOK - MODERN PUBLICATIONCHAPTER - MOTION IN A PLANE EXERCISE - COMPETITION FILE OBJECTIVE TYPE QUESTIONS (B. Multiple Choice Questions)38videos
MOTION IN A PLANE
- BOOK - MODERN PUBLICATIONCHAPTER - MOTION IN A PLANE EXERCISE - COMPETITION FILE OBJECTIVE TYPE QUESTIONS (JEE (Main) & Other Boards for Engineering Entrance)14videos
MOTION IN A PLANE
- BOOK - MODERN PUBLICATIONCHAPTER - MOTION IN A PLANE EXERCISE - Revision Exercises (Numerical Problems)26videos
MOTION IN A PLANE
- BOOK - MODERN PUBLICATIONCHAPTER - MECHANICAL PROPERTIES OF FLUIDSEXERCISE - Chapter Practise Test16videos
MECHANICAL PROPERTIES OF FLUIDS
- BOOK - MODERN PUBLICATIONCHAPTER - MOTION IN A STRAIGHT LINE EXERCISE - CHAPTER PRACTICE TEST16videos
MOTION IN A STRAIGHT LINE
Similar Questions
A projectile is thrown from ground at a speed v0 at an angle α to the horizontal. Consider point of projection as origin, horizontal direction as X axis and vertically upward as Y axis. Let t be the time when the velocity vector of the projectile becomes perpendicular to its position vector.
(a) Write a quadratic equation in t.
(b) What is the maximum angle a for which the distance of projectile from the point of projection always keeps on increasing? [Hint: Start from the equation you obtained in part (a)]View SolutionA particle is projected from a point on the level ground and its height is h when at horizontal distances aand2a from its point of projection. Find the velocity of projection.
View Solution
Knowledge Check
- Question 1 - Select One
A particle is projected from the horizontal x-z plane, in vertical x-y plane where x-axis is horizontal and positive y-axis vertically upwards. The graph of y coordinate of the particle v/s time is as shown . The range of the particle is √3. Then the speed of the projected particle is:A√3msB√4034msC2√5msD√28ms - Question 2 - Select One
A particle is projected with speed 30m/s at angle 22.5∘ with horizontal from ground as shown. AB and CD are parallel to y-axis and B is highest point of trajectory of particle. CD/AB is
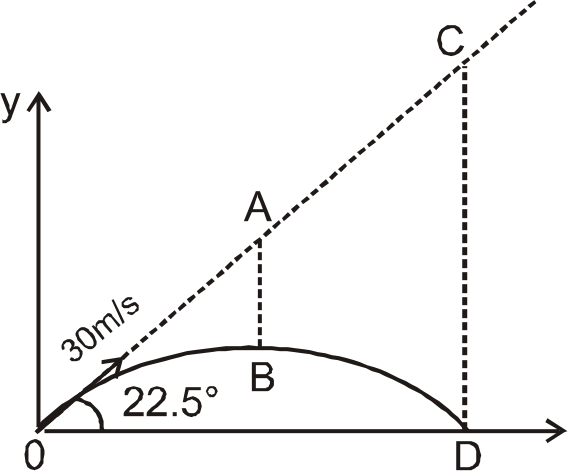A3B3/2C2D4
A particle is projected from a point on the level ground and its height is h when at horizontal distances aand2a from its point of projection. Find the velocity of projection.
View Solution-
A particle is projected from the horizontal x-z plane, in vertical x-y plane where x-axis is horizontal and positive y-axis vertically upwards. The graph of y coordinate of the particle v/s time is as shown . The range of the particle is √3. Then the speed of the projected particle is:View Solution A projectile is projected with velocity u making angle θ with horizontal direction, find : horizontal range.
View SolutionA particle is projected from ground at some angle with horizontal (Assuming point of projection to the origin and the horizontal and vertical directions to be the x and y axis) the particle passes through the points (3, 4) m and (4, 3) m during its motion then the range of the particle would be : (g=10m/s2)
View SolutionA particle is projected from ground at some angle with horizontal (Assuming point of projection to the origin and the horizontal and vertical directions to be the x and y axis) the particle passes through the points (3, 4) m and (4, 3) m during its motion then the range of the particle would be : (g=10m/s2)
View SolutionA particle is projected with speed 30m/s at angle 22.5∘ with horizontal from ground as shown. AB and CD are parallel to y-axis and B is highest point of trajectory of particle. CD/AB is
View Solution