A particle is projected in aer an angle to a surface which itself is inclined at an angle to the horixonta (Fig. 2 (EP). 26)
(a) Find an ecxpression for range on the plane surface (distanc eon the plance from the point of projection at which particle will hit the surface). (b) Time of flight. 9c ) at which range will be maximum.
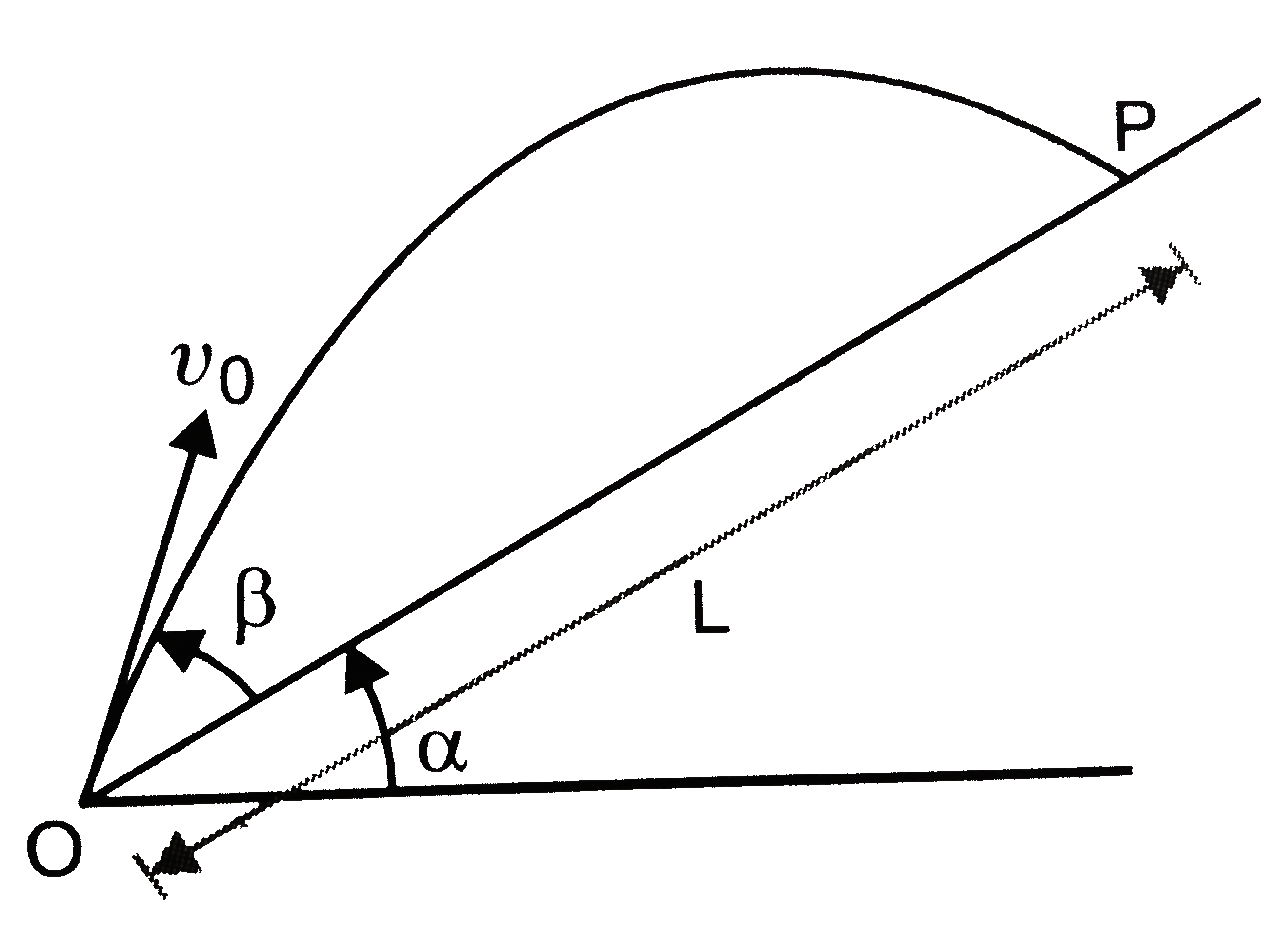
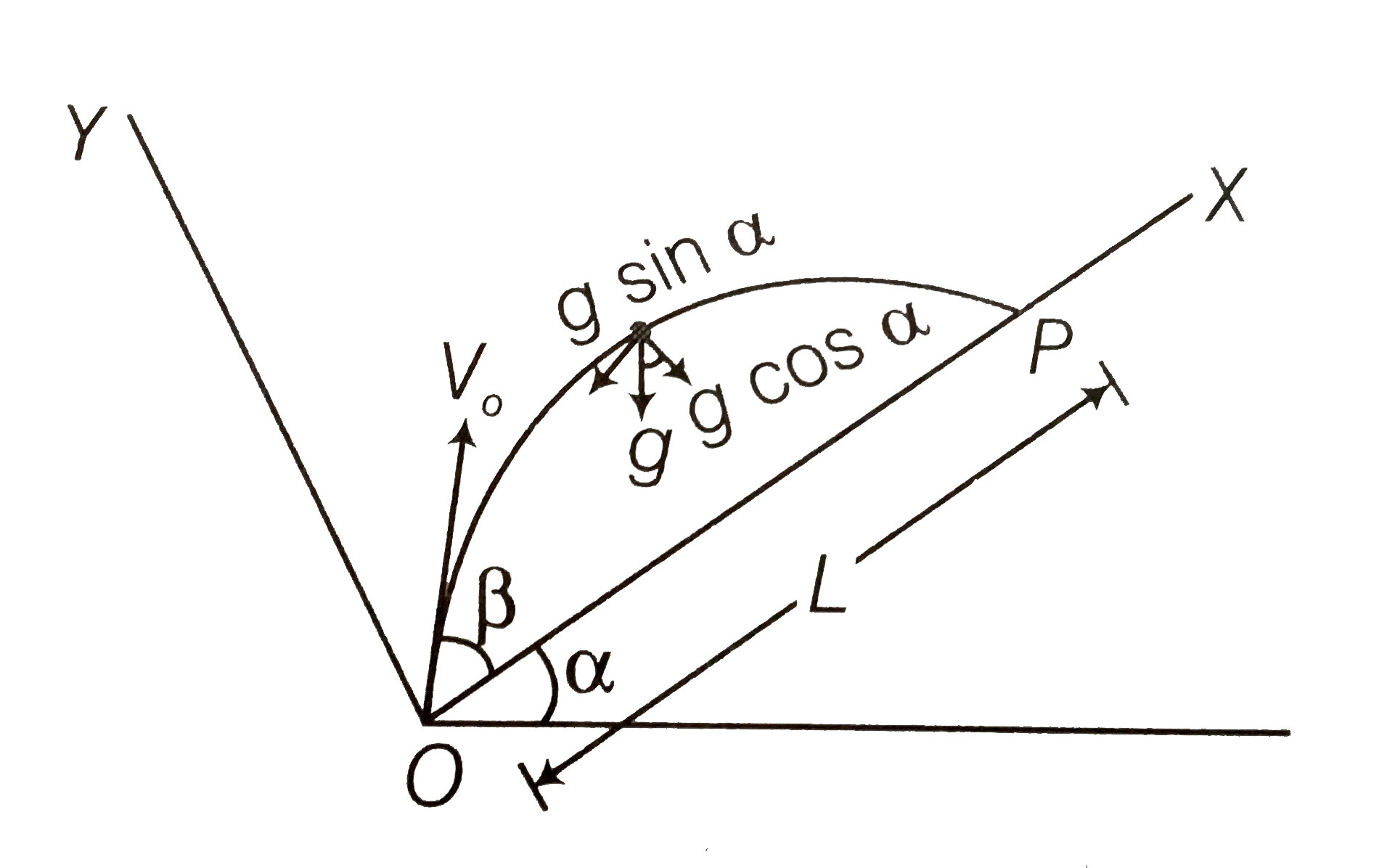
Consider the adjacent diagram.
Mutually perpendicular x and y-axes are shown in the diagram.
Particle is projected from the point O.
Let time taken in reaching from point O to point P is T.
(b) Considering motion along vertical upward direction perpendicular to OX.
For the journey O to P.
Applying equation,
As T = 0, corresponding to point O
Hence,
(a) Considering motion along OX,
(c) For range (L) to be maximum.
Let,
For z to be maximum,
=maximum = 1
Topper's Solved these Questions
Similar Questions
A particle is projected horizontally with a speed u from the top of plane inclined at an angle θ with the horizontal. How far from the point of projection will the particle strike the plane ?

View SolutionA particle is projected horizontal with a speed u from the top of plane inclined at an angle θ with the horizontal. How far from the point of projection will the particle strike the plane?
View Solution
Knowledge Check
The ratio of the greatest range up an inclined plane through the point of projection and the distance through which a particle falls freely during the corresponding time of flight is
A2B12C1D3A particle is projected at an angle α with the horizontal from the foot of an inclined plane making an angle β with horizontal . Which of the following expressions holds good if the particle strikes the inclined plane normally?
Acotβ=−tan(α−β)Bcotβ=2tan(α−β)Ccotα=tan(α−β)Dcotα=2tan(α−β)A particle I projected at an angle of elevation α after time t it makes an angle βwith horizontal. The velocity of projection is
A2gtcosβsin(α−β)Bgtcosβsin(α−β)Cgt2sin(α−β)D4gtsin(α−β)
A particle is projected from the bottom of an inclined plane of inclination 30∘. At what angle α(from the horizontal) should the particle be projected to get the maximum range on the inclined plane.
View SolutionA particles is projected horizontally with a speed v from the top of a plane inclined at an angle tehta to the horizontal as shown in the figure.
(a) Hwo far from the point of projection will the particle strike the plane ?
(b) Find the time taken by the particel to hit the plane.
(c) What is the velocity of particle just before it hits the plane ?
View SolutionA particle is projected up an inclines plane. Plane is inclined at an angle θ with horizontal and particle is projected at an angle α with horizontal. If particle strikes the plane horizontally prove that tanα=2tanθ
View SolutionA particle is projected at an angle with horizontal. If T is the time of flight and R is a horizontal range, then θ is
View SolutionA particle is projected horizontally with a speed V=5m/s from the top of a plane inclined at an angle θ=37∘ to the horizontal (g=10m/s2)
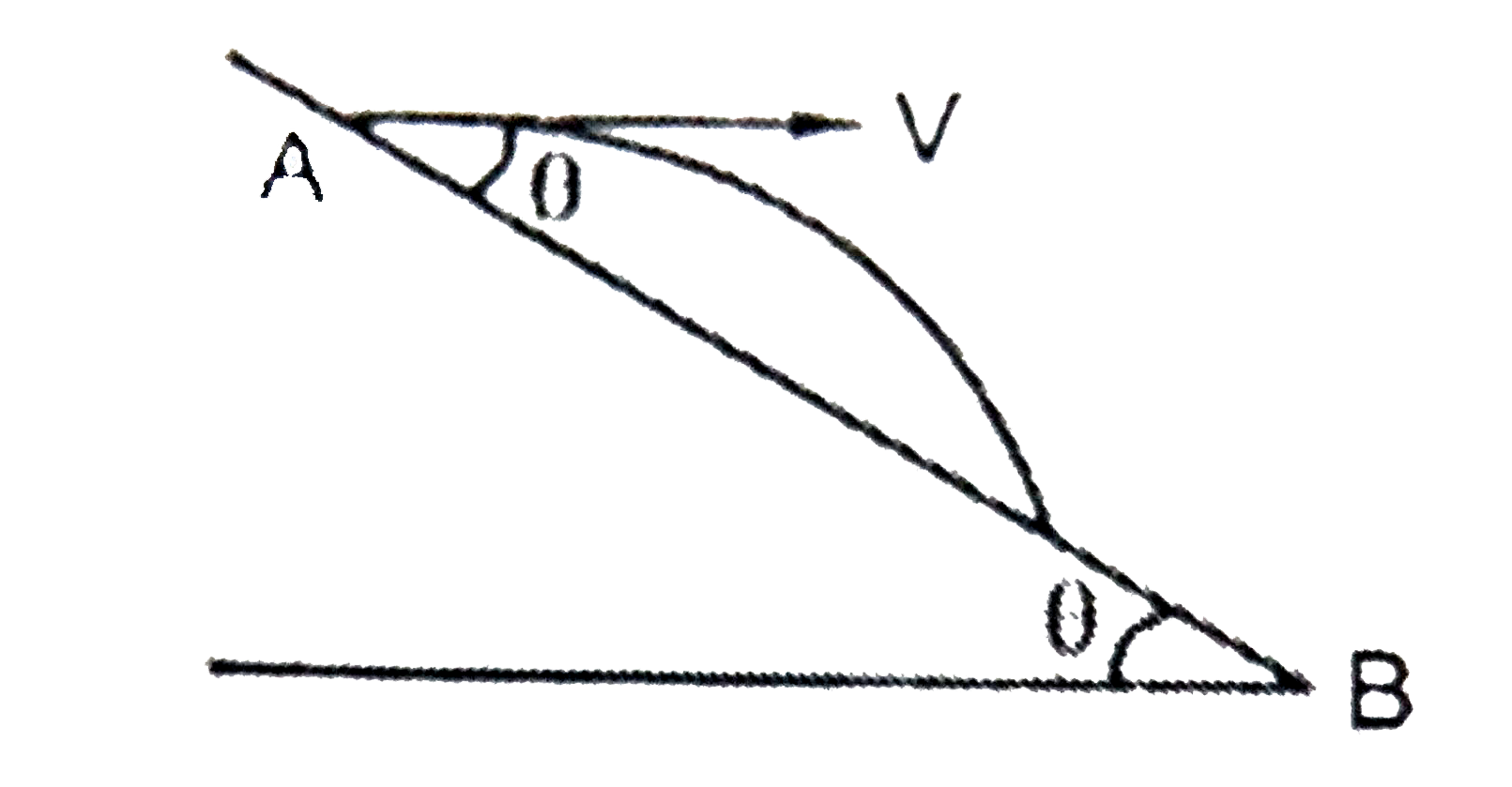
Find the time taken by the particle to hit the plane.
View Solution
