A particle is thrown from the origin,at an angle θ(0<θ<90∘) such that it just crosses a wall of height 9m. Wall is at x=12m. Speed of projection is n√3ms and particle strikes the ground at x=48m. Value of n is (g=10m/s2)
The correct Answer is:4
Step by step video, text & image solution for A particle is thrown from the origin,at an angle theta(0lttheta lt 90^(@)) such that it just crosses a wall of height 9m. Wall is at x=12m. Speed of projection is nsqrt(3)m/s and particle strikes the ground at x=48m. Value of n is (g=10m//s^(2)) by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Topper's Solved these Questions
Similar Questions
A body projected just crosses a wall of 4m height,at 6m distance from the point of projection and it hits the ground at 12m away from the wall. The angle of projection is nearly
View SolutionA particle is projected with a velocity 10 m//s at an angle 37∘ to the horizontal. Find the location at which the particle is at a height 1 m from point of projection.
View Solution
Knowledge Check
- Question 1 - Select One or More
A particle is projected at an angle θ from ground with speed u (g = 10 m/s2 )
Aif u = 10 m/s and θ=30∘, then time of flight will be 1 secBIf u=10√3m/sandθ=60∘, then time of flight will be 3 sec.Cif u=10√3m/sandθ=60∘, then after 2 sec velocity becomes perpendicular to initial velocity.Dif u = 10 m/s and θ=30∘, then velocity never becomes perpendicular to initial velocity during its flight. - Question 2 - Select One
A particle is projected horizontally with a speed V=5m/s from the top of a plane inclined at an angle θ=37∘ to the horizontal (g=10m/s2)
How far from the point of projection will the particle strike the plane?A75mB6516mC7516mD859m - Question 3 - Select One
A particle projected at some angle with velocity 50 m/s crosses a 20 m high wall after 4 s from the time of projection. The angle of porjection of the particle is
A30∘B45∘C60∘D53∘
A body projected from a point O at an angle θ, just crosses a wall y m high at a distance. x m from the point of projection and strikes the ground at Q beyond the wall as shown, then find height of the wall
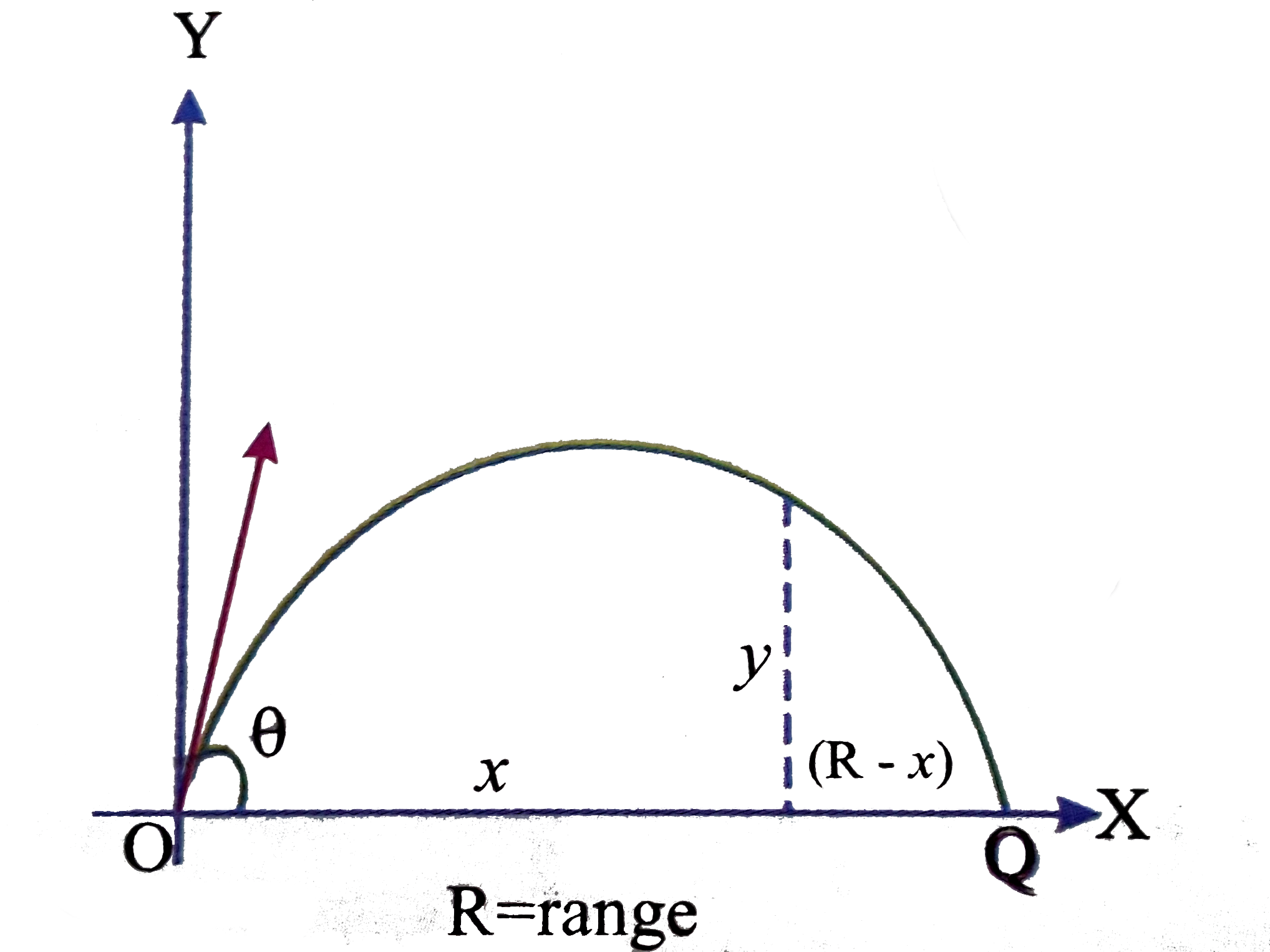 View Solution
View SolutionA ball is thrown from a point at a distance 40m from a wall of height 15m. It just clears the wall and then attains maximum height. Find the maximum height the angle of projection is 45∘.
View SolutionA particle is projected from the earth's surface with a velocity of 50 m s−1 at an angle θ with the horizontal. After 2s it just clears a wall 5m high. What is the value of 55sinθ?(g=10ms−2)
View SolutionA particle is thrown vertically up from the top of a building of height 20m with initial velocity u. A second particle is released from the same point 1 second later. If both particles reach the ground at the same instant ,3u=m/s. [Takeg=10m/s2]
View SolutionA ball projected from ground at an angle of 45∘ just clears a wall infront. If point of projection is 4m from the foot of wall and ball strikes the ground at a distance of 6m on the other side of the wall, the height of the wall is
View Solution

