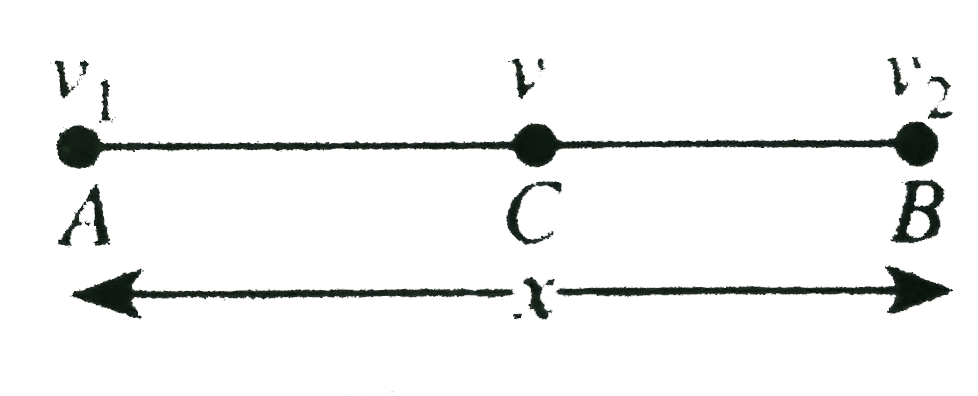
A particle moving with uniform acceleration from $A$ to $B$ along a straight line has velcities $v_{1}$ and $v_{2}$ at $A$ and $B$ respectively. If $C$ is the mid-point between $A$ and $B$ then determine the velocity of the particle at $C$ .
.

Video Solution

Text Solution

Verified by Experts

Let $v$ be the velocity of the particle at $c$ . Assume the acceleration of the particle to be $A$ and distance between $A$ and $B$ be $x$ . To find the velocity $c$ , consider the motion from $A$ and $C$ :
Applying $v^{2} - u^{2} = 2$ as, we get $v^{2} - v_{1}^{2} = 2 a \frac{x}{2}$
$\Rightarrow v^{2} - v_{1}^{2} = a x$ ........ (i)
Appying the same equation from $C$ to $B$ . we get $< b r >$ V_(2)^(2)=2a(x)/(2) rArr v_(2)^(2)-v^(2)=ax $... ... (i i) < b r > F r o m (i) and (i i), w e \geq t$ v=sqrt(v_(1)^(2)+v_(2)^(2))/(2).

Updated on:21/07/2023