A body is moving with constant acceleration, a=5ms2 from A to B in straight line AB. The velocity of particle at A and B are 5 m/s and 15 m/s respectively. The velocity at mid-point of AB is
√125ms
√250ms
√550ms
√600ms
The correct Answer is:A
To find the velocity at the midpoint of the straight line AB, we can follow these steps:
Step 1: Identify the given values
- Initial velocity at point A, VA=5m/s
- Final velocity at point B, VB=15m/s
- Constant acceleration, a=5m/s2
Step 2: Use the third equation of motion to find the distance S from A to B
The third equation of motion is given by:
V2=U2+2aS
Where:
- V is the final velocity (at B)
- U is the initial velocity (at A)
- a is the acceleration
- S is the distance
Substituting the known values:
152=52+2⋅5⋅S
Calculating the squares:
225=25+10S
Rearranging the equation:
225−25=10S
200=10S
Dividing both sides by 10:
S=20m
Step 3: Find the midpoint distance
Since the total distance S from A to B is 20 m, the distance to the midpoint O is:
Distance to midpoint=S2=202=10m
Step 4: Use the third equation of motion again to find the velocity at the midpoint VO
We will use the same equation, but now we will calculate the velocity at the midpoint:
V2O=V2A+2a⋅Distance to midpoint
Substituting the known values:
V2O=52+2⋅5⋅10
Calculating the squares:
V2O=25+100
V2O=125
Taking the square root to find VO:
VO=√125m/s
VO=11.18m/s(approximately)
Final Answer
The velocity at the midpoint of AB is approximately 11.18m/s.
---
- Initial velocity at point A, VA=5m/s
- Final velocity at point B, VB=15m/s
- Constant acceleration, a=5m/s2
The third equation of motion is given by:
V2=U2+2aS
Where:
- V is the final velocity (at B)
- U is the initial velocity (at A)
- a is the acceleration
- S is the distance
152=52+2⋅5⋅S
Calculating the squares:
225=25+10S
Rearranging the equation:
225−25=10S
200=10S
Dividing both sides by 10:
S=20m
Since the total distance S from A to B is 20 m, the distance to the midpoint O is:
Distance to midpoint=S2=202=10m
We will use the same equation, but now we will calculate the velocity at the midpoint:
V2O=V2A+2a⋅Distance to midpoint
Substituting the known values:
V2O=52+2⋅5⋅10
Calculating the squares:
V2O=25+100
V2O=125
Taking the square root to find VO:
VO=√125m/s
VO=11.18m/s(approximately)
The velocity at the midpoint of AB is approximately 11.18m/s.
Topper's Solved these Questions
Similar Questions
A point moving with constant acceleration from A to B in the straight line AB has velocities u and v at A and B respectively. Find its velocity at C, the mid point of AB. Also show that if the time from A to C is twice that from C to B, then v=7u.
View SolutionA particle moving with uniform acceleration from A to B along a straight line has velcities v1 and v2 at A and B respectively. If C is the mid-point between A and B then determine the velocity of the particle at C .
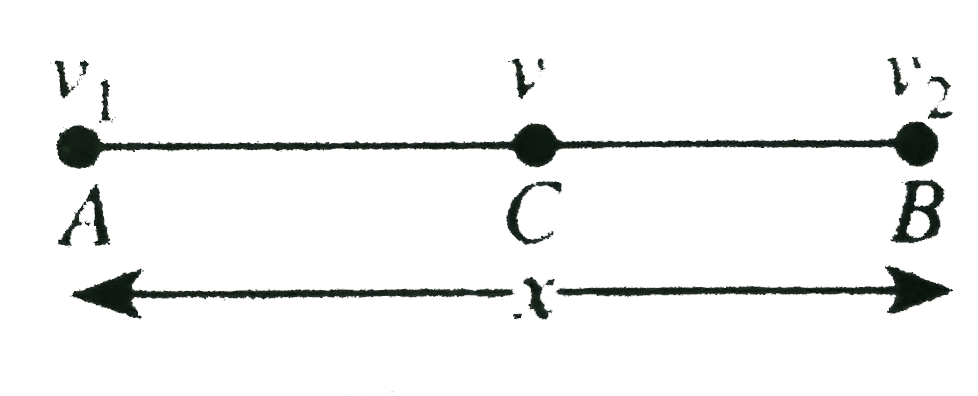 .
.
View SolutionThe velocity of a particle moving with constant acceleration at an instant t0 is 10m/s After 5 seconds of that instant the velocity of the particle is 20m/s. The velocity at 3 second before t0 is:-
View SolutionA particle goes from A to B in 10s with uniform acceleration. Its velocity at A and B are 5 m/s and 25 m/s. Its acceleration (in m/s2) is
View SolutionA body travelling with uniform acceleration crosses two point A and B with velocities 20ms−1 and 30ms−1 respectively. The speed of the body at the mid-point of A and B is.
View SolutionThe velocity of a projectile at the initial point A is (2ˆi+3ˆj)m/s. Its velocity (in m/s) at point B is

View SolutionA cyclist is moving with a constant acceleration of 1.2 m/s2 on a straight track. A racer is moving on a circular path of radius 150 m at constant speed of 15 m/s. Find the magnitude of velocity of racer which is measured by the cyclist has reached a speed of 20 m/s for the position represented in the figure -

View SolutionThe acceleration-time graph of a particle moving in a straight line is as shown in figure. The velocity of the particle at time t=0 is 2m/s. The velocity after 2 seconds will be
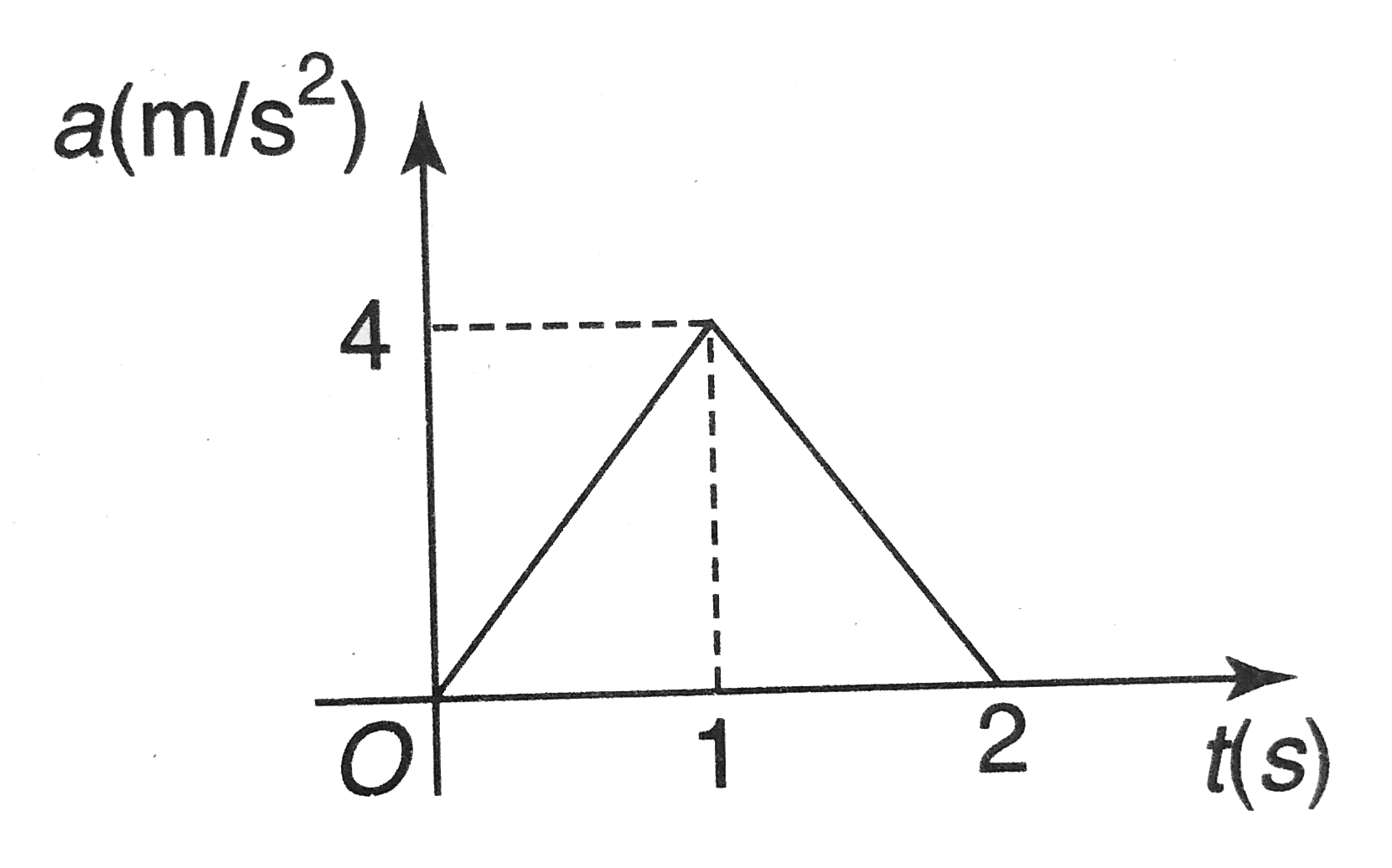
View SolutionThe acceleration-time graph of a particle moving in a straight line is as shown in figure. The velocity of the particle at time t=0 is 2m/s. The velocity after 2 seconds will be

View SolutionA car moving with constant acceleration crosses two points A and B with velocities 10 m/s and 20 m/s in its path. Find the velocity of the car midway between A and B. (in m/s).
View Solution
