A particle of charge q and mass m moving with a velocity v along the x-axis enters the region x>0 with uniform magnetic field B along the ˆk direction. The particle will penetrate in this region in the x-direction upto a distance d equal to
Zero
mvqB
2mvqB
Infinity
The correct Answer is:B
To solve the problem of a charged particle moving in a magnetic field, we can follow these steps:
Step 1: Understand the Motion of the Particle
The particle of charge q and mass m is moving with a velocity v along the x-axis. When it enters the region where x>0, it encounters a uniform magnetic field →B directed along the ^k direction (which is the z-direction). The magnetic field will exert a force on the charged particle, causing it to move in a circular path.
Hint: Remember that the magnetic force acts perpendicular to the velocity of the charged particle, causing circular motion.
Step 2: Identify the Force Acting on the Particle
The magnetic force →F acting on the particle can be given by the Lorentz force equation:
→F=q→v×→B
Since the velocity →v is along the x-axis and the magnetic field →B is along the z-axis, the force will act in the y-direction.
Hint: Use the right-hand rule to determine the direction of the magnetic force.
Step 3: Determine the Radius of the Circular Path
The particle will move in a circular path due to the magnetic force. The radius R of this circular path can be derived from the balance of the magnetic force and the centripetal force required for circular motion. The centripetal force is given by:
Fc=mv2R
Setting the magnetic force equal to the centripetal force:
qvB=mv2R
From this equation, we can solve for the radius R:
R=mvqB
Hint: Make sure to isolate R correctly by rearranging the equation.
Step 4: Relate the Distance d to the Radius
In this scenario, the distance d that the particle penetrates into the region while moving in the x-direction is equal to the radius R of the circular path.
Thus, we can conclude:
d=R=mvqB
Hint: Remember that the distance traveled in the x-direction corresponds to the radius of the circular path due to the magnetic force.
Final Answer
The distance d that the particle will penetrate in the x-direction is given by:
d=mvqB
The particle of charge q and mass m is moving with a velocity v along the x-axis. When it enters the region where x>0, it encounters a uniform magnetic field →B directed along the ^k direction (which is the z-direction). The magnetic field will exert a force on the charged particle, causing it to move in a circular path.
The magnetic force →F acting on the particle can be given by the Lorentz force equation:
→F=q→v×→B
Since the velocity →v is along the x-axis and the magnetic field →B is along the z-axis, the force will act in the y-direction.
The particle will move in a circular path due to the magnetic force. The radius R of this circular path can be derived from the balance of the magnetic force and the centripetal force required for circular motion. The centripetal force is given by:
Fc=mv2R
Setting the magnetic force equal to the centripetal force:
qvB=mv2R
From this equation, we can solve for the radius R:
R=mvqB
In this scenario, the distance d that the particle penetrates into the region while moving in the x-direction is equal to the radius R of the circular path.
d=R=mvqB
The distance d that the particle will penetrate in the x-direction is given by:
d=mvqB
Topper's Solved these Questions
- MAGNETIC FIELD AND MAGNETIC FORCESBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - MAGNETIC FIELD AND MAGNETIC FORCESEXERCISE - Comprehension type
8 Videos
- MAGNETIC FIELD AND MAGNETIC FORCESBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - MAGNETIC FIELD AND MAGNETIC FORCESEXERCISE - Subjective
11 Videos
- MAGNETIC FIELD AND MAGNETIC FORCESBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - MAGNETIC FIELD AND MAGNETIC FORCESEXERCISE - Archives Assertion-reasion
1 Videos
- INDUCTANCEBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - INDUCTANCEEXERCISE - Concept Based
8 Videos
- MISCELLANEOUS VOLUME 3BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - MISCELLANEOUS VOLUME 3EXERCISE - True and False
3 Videos
Similar Questions
A charge particle of charge q and mass m is moving with velocity v as shown in fig In a uniform magnetic field B along -ve z-direction.Select the correct alternative (s).

View SolutionA positively charged particle moving due east enters a region of uniform magnetic field directed vertically upwards. The partical will be
View SolutionA particle of mass m and charge q moves with a constant velocity v along the positive x direction. It enters a region containing a uniform magnetic field B directed along the negative z direction, extending from x=a to x=b. The minimum value of v required so that the particle can just enter the region x>b is
View SolutionA particle of mass m and charge q moves with a constant velocity v along the positive x− direction. It enters a region containing a uniform magnetic field B directed along the negative z direction, extending from x=a to x=b. The minimum value of v required so that the particle can just enter the region x>b is

View SolutionA charged particle (charge q, mass m) has velocity v0 at origin in +x direction. In space there is a uniform magnetic field B in -z direction. Find the y coordinate of particle when is crosses y axis.
View SolutionA moving charged particle q travelling along the positive X-axis enters a uniform magnetic field B. When will the force acting on q be maximum?
View SolutionA charge q moves with a velocity 2m/s along x- axis in a unifrom magnetic field B=(ˆi+2ˆj+3ˆk) tesla.
View SolutionA proton is moving along the negative direction of X-axis in a magnetic field directed along the positive direction of Y-axis. The proton will be deflected along the negative direction of
View SolutionA particle with mass m and charge q moving with a velocity v, enters a region of uniform magnetic field B, shown, in the figure above. The particle strikes the wall at a distance d from the entance slit. If the paticle's velocity stays the same but its charge to mass ratio is doubled, distance frm the entrance slit where the particle strike the wall is dn, then find n
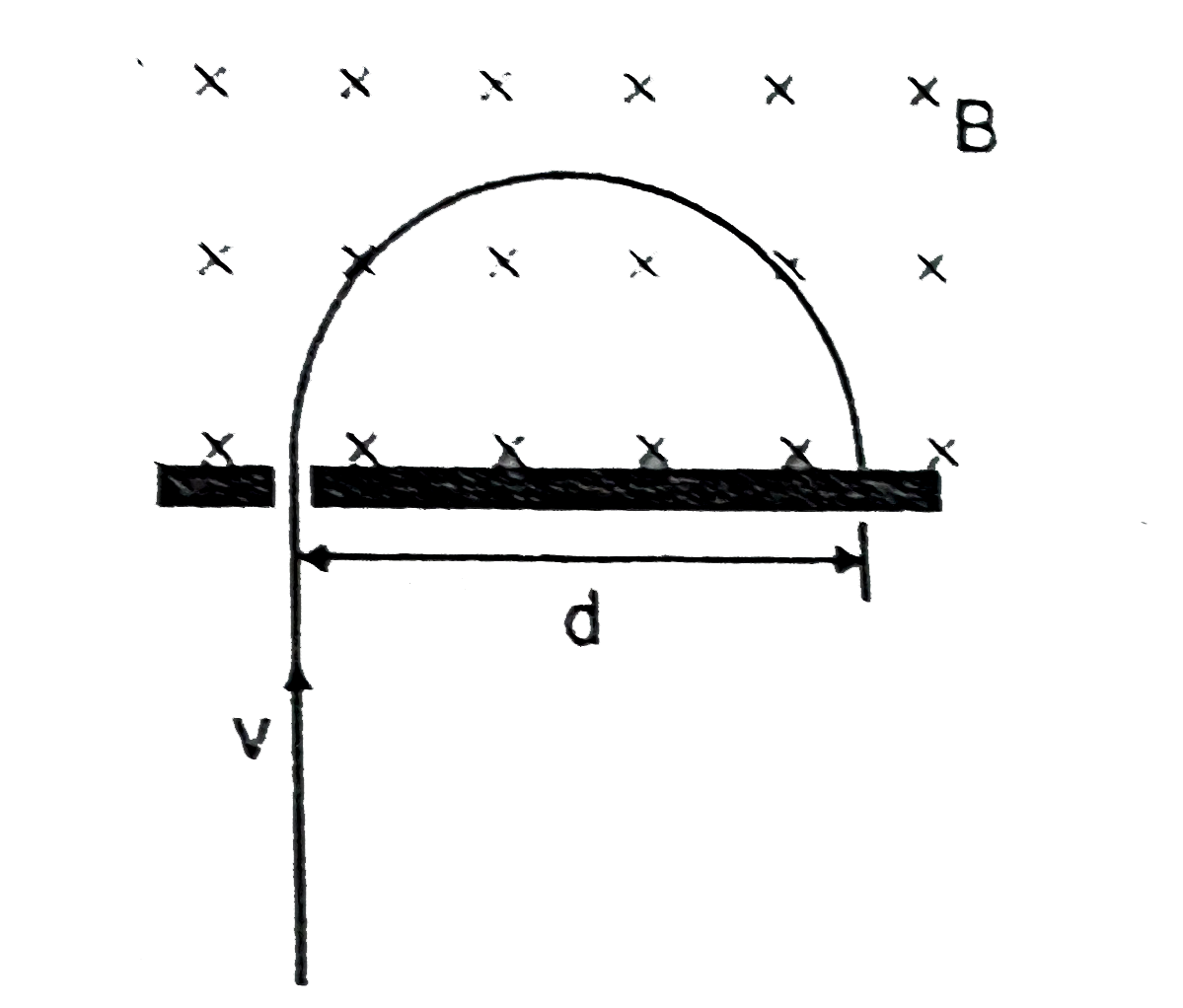
View SolutionA particle with mass m and charge q moving with a velocity v, enters a region of uniform magnetic field B, shown, in the figure above. The particle strikes the wall at a distance d from the entance slit. If the paticle's velocity stays the same but its charge to mass ratio is doubled, distance frm the entrance slit where the particle strike the wall is dn, then find n

View Solution
