A particle of mass m and charge q moves with a constant velocity v along the positive x direction. It enters a region containing a uniform magnetic field B directed along the negative z direction, extending from x=a to x=b. The minimum value of v required so that the particle can just enter the region x>b is
qpB/m
q(b−a)B/m
qaB/m
q(b+a)B/2m
The correct Answer is:B
To solve the problem, we need to determine the minimum velocity v required for a charged particle to just enter the region beyond x=b when it moves through a magnetic field. Here’s a step-by-step breakdown of the solution:
Step 1: Understand the motion of the particle
The particle of mass m and charge q is moving along the positive x-direction with a constant velocity v. When it enters the magnetic field region, which is directed along the negative z-direction, it will experience a magnetic force.
Hint: Recall that the magnetic force on a charged particle moving in a magnetic field is given by the Lorentz force equation:
F=q(v×B)
Step 2: Determine the direction of the magnetic force
Using the right-hand rule, if the velocity v is in the positive x-direction and the magnetic field B is in the negative z-direction, the magnetic force F will be directed in the positive y-direction.
Hint: Visualize the right-hand rule: point your thumb in the direction of v and your fingers in the direction of B to find the direction of F.
Step 3: Analyze the particle's trajectory
The particle will undergo circular motion due to the magnetic force acting as the centripetal force. The radius r of this circular motion can be expressed as:
r=mvqB
Hint: Remember that the centripetal force required for circular motion is provided by the magnetic force.
Step 4: Relate the radius to the magnetic field region
For the particle to just enter the region beyond x=b, the radius of the circular path must be equal to the width of the magnetic field region, which is b−a. Therefore, we set:
mvqB=b−a
Hint: This equation relates the radius of the circular path to the dimensions of the magnetic field region.
Step 5: Solve for the velocity v
Rearranging the equation gives:
v=qB(b−a)m
This equation gives us the minimum velocity required for the particle to just enter the region x>b.
Hint: Ensure you understand how each variable in the equation affects the velocity.
Final Answer
The minimum value of v required for the particle to just enter the region x>b is:
v=qB(b−a)m
The particle of mass m and charge q is moving along the positive x-direction with a constant velocity v. When it enters the magnetic field region, which is directed along the negative z-direction, it will experience a magnetic force.
F=q(v×B)
Using the right-hand rule, if the velocity v is in the positive x-direction and the magnetic field B is in the negative z-direction, the magnetic force F will be directed in the positive y-direction.
The particle will undergo circular motion due to the magnetic force acting as the centripetal force. The radius r of this circular motion can be expressed as:
r=mvqB
For the particle to just enter the region beyond x=b, the radius of the circular path must be equal to the width of the magnetic field region, which is b−a. Therefore, we set:
mvqB=b−a
Rearranging the equation gives:
v=qB(b−a)m
The minimum value of v required for the particle to just enter the region x>b is:
v=qB(b−a)m
Topper's Solved these Questions
- MAGNETIC FIELD AND FORCESBOOK - DC PANDEY ENGLISHCHAPTER - MAGNETIC FIELD AND FORCESEXERCISE - Assertion and reason
20 Videos
- MAGNETIC FIELD AND FORCESBOOK - DC PANDEY ENGLISHCHAPTER - MAGNETIC FIELD AND FORCESEXERCISE - Match the following
4 Videos
- MAGNETIC FIELD AND FORCESBOOK - DC PANDEY ENGLISHCHAPTER - MAGNETIC FIELD AND FORCESEXERCISE - Check point
54 Videos
- INTERFERENCE AND DIFFRACTION OF LIGHTBOOK - DC PANDEY ENGLISHCHAPTER - INTERFERENCE AND DIFFRACTION OF LIGHTEXERCISE - Level 2 Subjective
5 Videos
- MAGNETICSBOOK - DC PANDEY ENGLISHCHAPTER - MAGNETICSEXERCISE - MCQ_TYPE
1 Videos
Similar Questions
A particle of mass m and charge q moves with a constant velocity v along the positive x− direction. It enters a region containing a uniform magnetic field B directed along the negative z direction, extending from x=a to x=b. The minimum value of v required so that the particle can just enter the region x>b is

View SolutionA particle of mass m and charge Q moving with a velocity v enters a region on uniform field of induction B Then its path in the region is s
View SolutionA positively charged particle moving due east enters a region of uniform magnetic field directed vertically upwards. The partical will be
View SolutionA particle of charge q and mass m moving with a velocity v along the x-axis enters the region x>0 with uniform magnetic field B along the ˆk direction. The particle will penetrate in this region in the x-direction upto a distance d equal to
View SolutionA charged particle (charge q, mass m) has velocity v0 at origin in +x direction. In space there is a uniform magnetic field B in -z direction. Find the y coordinate of particle when is crosses y axis.
View SolutionA particle of mass m carrying charge q is accelerated by a potential difference V. It enters perpendicularly in a region of uniform magnetic field B and executes circular arc of radius R, then qm equals
View SolutionA particle of mass m is moving with constant speed v on the line y=b in positive x-direction. Find its angular momentum about origin, when position coordinates of the particle are (a, b).
View SolutionA charged particle ( mass m and charge q) moves along X axis with velocity V0 . When it passes through the origin it enters a region having uniform electric field →E=−Eˆj which extends upto x=d . Equation of path of electron in the region . x>d is :
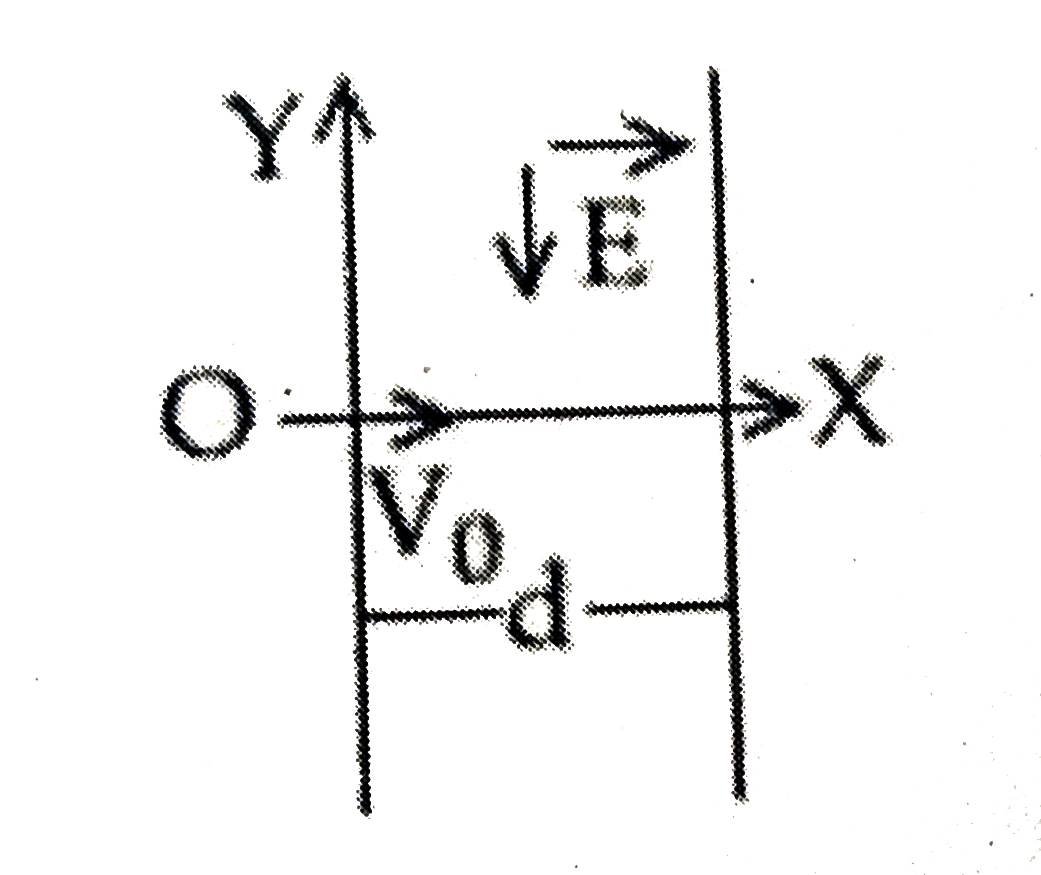
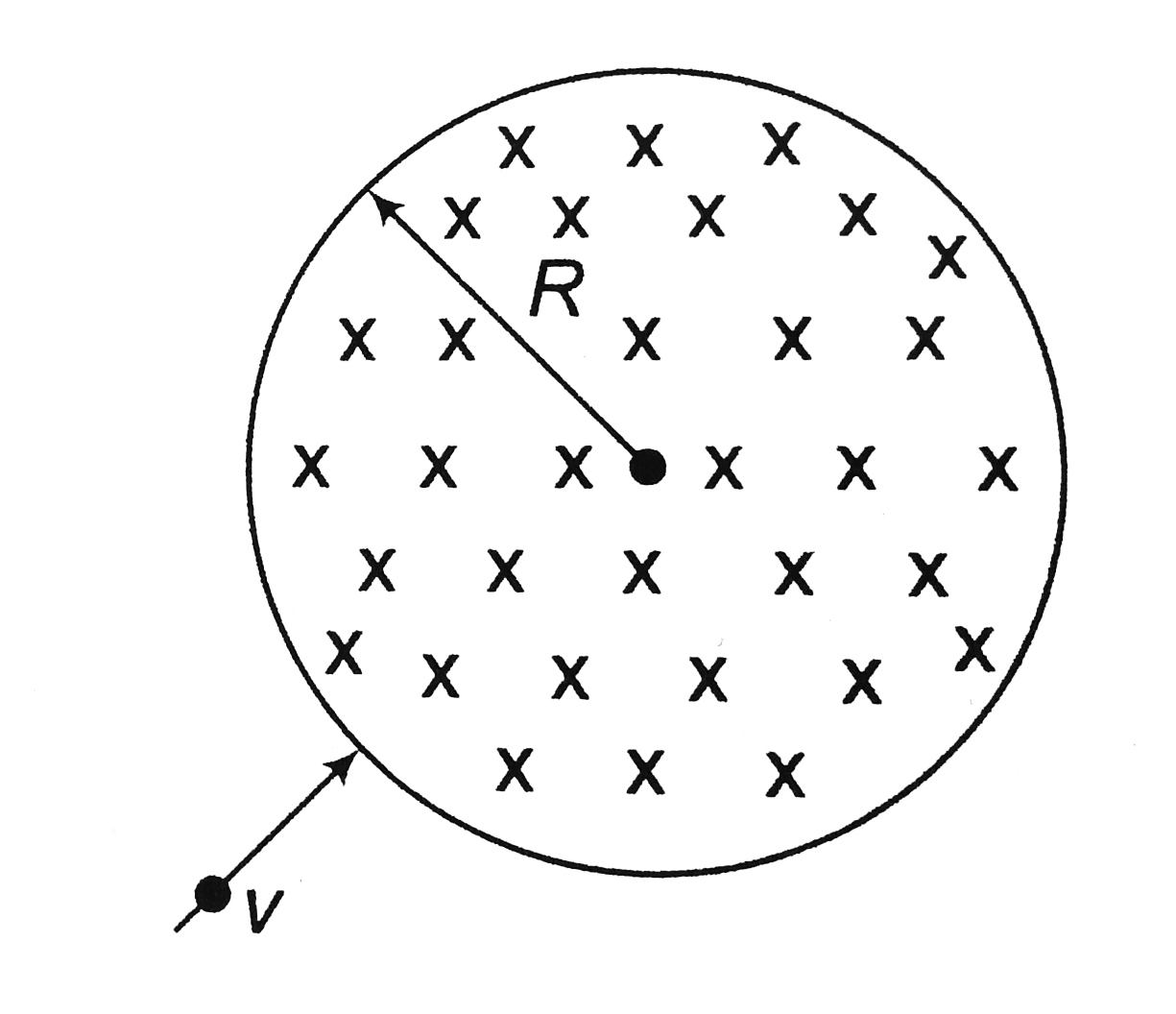
View SolutionA particle of mass m having a charge q enters into a circular region of radius R with velocity v directed towards the centre. The strength of magnetic field is B. Find the deviation in the path of the particle.
View SolutionA moving charged particle q travelling along the positive X-axis enters a uniform magnetic field B. When will the force acting on q be maximum?
View Solution
