A particle of mass 1 kg is projected upwards with velocity 60ms−1. Another particle of mass 2kg is just dropped from a certain height. After 2s, match the following.
[Take, g =10ms−2]
column1column2Accelertion of CMP ZeroB Velocity of CMQ 10 SI unitC Displacement of CMR 20 SI unit-S None
The correct Answer is:A → q, B→p, C → r
To solve the problem step by step, we will analyze the motion of both particles and calculate the required quantities: acceleration, velocity, and displacement of the center of mass (CM).
Step 1: Calculate the Acceleration of the Center of Mass (CM)
The acceleration of the center of mass (CM) for two particles can be calculated using the formula:
aCM=m1a1+m2a2m1+m2
Where:
- m1=1kg (mass of the first particle)
- m2=2kg (mass of the second particle)
- a1=−g=−10m/s2 (acceleration of the first particle, upwards)
- a2=g=10m/s2 (acceleration of the second particle, downwards)
Substituting the values:
aCM=1⋅(−10)+2⋅101+2=−10+203=103m/s2
Since both accelerations are directed downwards, we take g as positive for the downward direction. Hence, the acceleration of the center of mass is:
aCM=10m/s2
Step 2: Calculate the Velocity of the Center of Mass (CM)
To find the velocity of the CM after 2 seconds, we first calculate the velocities of both particles.
For Particle 1 (1 kg):
Using the equation of motion:
v1=u1+a1t
Where:
- u1=60m/s (initial velocity)
- a1=−10m/s2 (acceleration)
- t=2s
Substituting the values:
v1=60+(−10)⋅2=60−20=40m/s
For Particle 2 (2 kg):
Using the equation of motion:
v2=u2+a2t
Where:
- u2=0m/s (initial velocity)
- a2=10m/s2 (acceleration)
- t=2s
Substituting the values:
v2=0+10⋅2=20m/s
Now, we calculate the velocity of the center of mass:
vCM=m1v1+m2v2m1+m2
Substituting the values:
vCM=1⋅40+2⋅(−20)1+2=40−403=0m/s
Step 3: Calculate the Displacement of the Center of Mass (CM)
For Particle 1 (1 kg):
Using the equation of motion:
s1=u1t+12a1t2
Substituting the values:
s1=60⋅2+12⋅(−10)⋅(2)2=120−20=100m
For Particle 2 (2 kg):
Using the equation of motion:
s2=u2t+12a2t2
Substituting the values:
s2=0⋅2+12⋅10⋅(2)2=0+20=20m
Now, we calculate the displacement of the center of mass:
sCM=m1s1+m2s2m1+m2
Substituting the values:
sCM=1⋅100+2⋅(−20)1+2=100−403=603=20m
Final Results
- Acceleration of CM: 10m/s2
- Velocity of CM: 0m/s
- Displacement of CM: 20m
Matching the Results
- Acceleration of CM (A) matches with (Q) 10 SI unit.
- Velocity of CM (B) matches with (P) 0.
- Displacement of CM (C) matches with (R) 20 SI unit.
- m1=1kg (mass of the first particle)
- m2=2kg (mass of the second particle)
- a1=−g=−10m/s2 (acceleration of the first particle, upwards)
- a2=g=10m/s2 (acceleration of the second particle, downwards)
Using the equation of motion:
v1=u1+a1t
Where:
- u1=60m/s (initial velocity)
- a1=−10m/s2 (acceleration)
- t=2s
v1=60+(−10)⋅2=60−20=40m/s
Using the equation of motion:
v2=u2+a2t
Where:
- u2=0m/s (initial velocity)
- a2=10m/s2 (acceleration)
- t=2s
v2=0+10⋅2=20m/s
vCM=m1v1+m2v2m1+m2
Substituting the values:
vCM=1⋅40+2⋅(−20)1+2=40−403=0m/s
Using the equation of motion:
s1=u1t+12a1t2
Substituting the values:
s1=60⋅2+12⋅(−10)⋅(2)2=120−20=100m
Using the equation of motion:
s2=u2t+12a2t2
Substituting the values:
s2=0⋅2+12⋅10⋅(2)2=0+20=20m
sCM=m1s1+m2s2m1+m2
Substituting the values:
sCM=1⋅100+2⋅(−20)1+2=100−403=603=20m
- Velocity of CM: 0m/s
- Displacement of CM: 20m
- Velocity of CM (B) matches with (P) 0.
- Displacement of CM (C) matches with (R) 20 SI unit.
Topper's Solved these Questions
- BOOK - DC PANDEY ENGLISHCHAPTER - CENTRE OF MASSEXERCISE - Medical entrances gallery27videos
CENTRE OF MASS
- BOOK - DC PANDEY ENGLISHCHAPTER - CENTRE OF MASSEXERCISE - Assertion and reason21videos
CENTRE OF MASS
- BOOK - DC PANDEY ENGLISHCHAPTER - CALORIMETRY AND HEAT TRANSFEREXERCISE - Medical entrance s gallery38videos
CALORIMETRY AND HEAT TRANSFER
- BOOK - DC PANDEY ENGLISHCHAPTER - CENTRE OF MASS, IMPULSE AND MOMENTUMEXERCISE - Comprehension type questions15videos
CENTRE OF MASS, IMPULSE AND MOMENTUM
Similar Questions
A particle of mass 1 kg is projected upwards with velocity 60 m/s. Another particle of mass 2 kg is just deopper from a certain height. After 2 kg is just of the particles have colliided with ground, match the following [ Take g= 10 m/s2]
View SolutionIn the diagram shown in figure, match the following columns (take g=10ms−2)
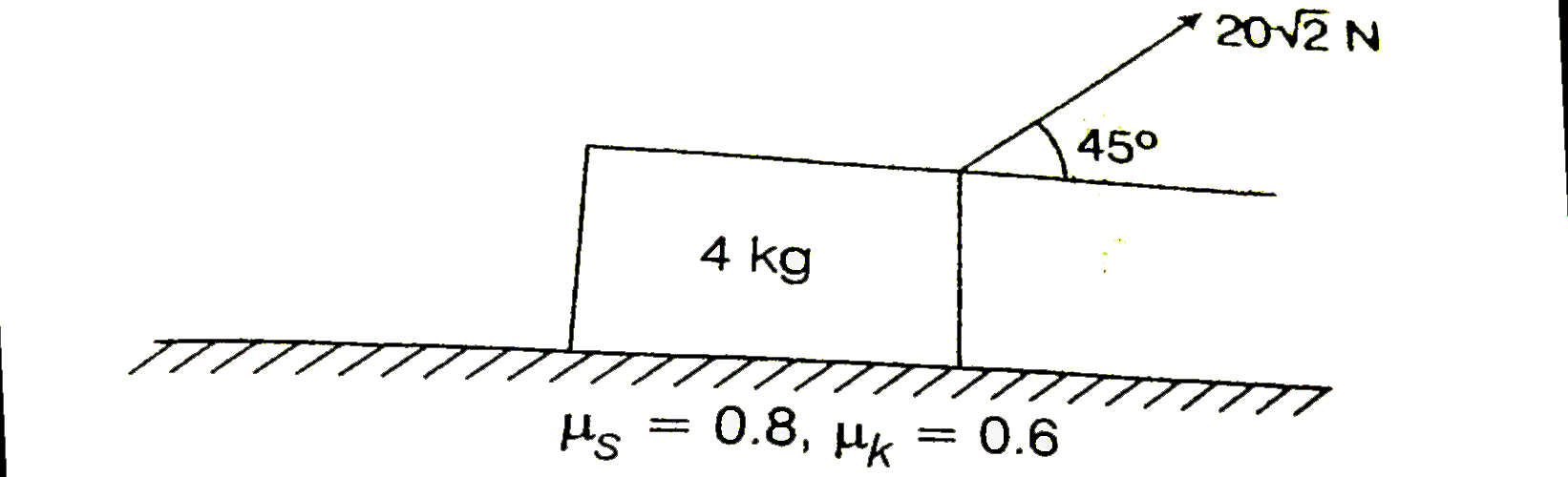
Column IColumn II(A) Normal reaction(p) 12 SI unit(B) Force of friction(q) 20 SI unit(C) Acceleration of block(r) zero(s) 2 SI unitView SolutionThe particle of mass 1 kg is projected with velocity 20√2m/s at 45∘ with ground . When , the particle is at highest point (g=10m/s2),
 View Solution
View SolutionA body of mass 1kg is thrown upwards with a velocity 20ms−1. It momentarily comes to rest after attaining a height of 18m. How much energy is lost due to air friction? (g=10ms−2)
View SolutionA balloon rises up with constant net acceleration of 10m/s2. After 2 s a particle drops from the balloon. After further 2 s match the following : (Takeg=10m/s2)
Column IColumn II(A)Height of perticle from ground(p)Zero(B)Speed of particle(q)10SIunits(C)Displacement of Particle(r)40SIunits(D)Acceleration of particle(s)20SIunitsView SolutionA particle of mass 2 kg chrge 1 mC is projected vertially with velocity k10ms−1. There is as uniform horizontal electric field of 104N/C, then
View SolutionA particle of mass 2 kg charge 1 mC is projected vertially with velocity k10ms−1. There is as uniform horizontal electric field of 104N/C, then
View SolutionA particle of mass 2 kg chrge 1 mC is projected vertially with velocity k10ms−1. There is as uniform horizontal electric field of 104N/C, then
View SolutionA bullet of mass 20g is fired horizontly with a velocity of 150ms−1 from a pistol of maass 2kg. What is the recoil velocity of the pistol?
View SolutionA particle of mass 2 kg is projected vertically upward with a velocity of 20 m/s. It attains maximum height of 17 m. The loss in mechanical energy due to air drag is (g=10ms−2)
View Solution

