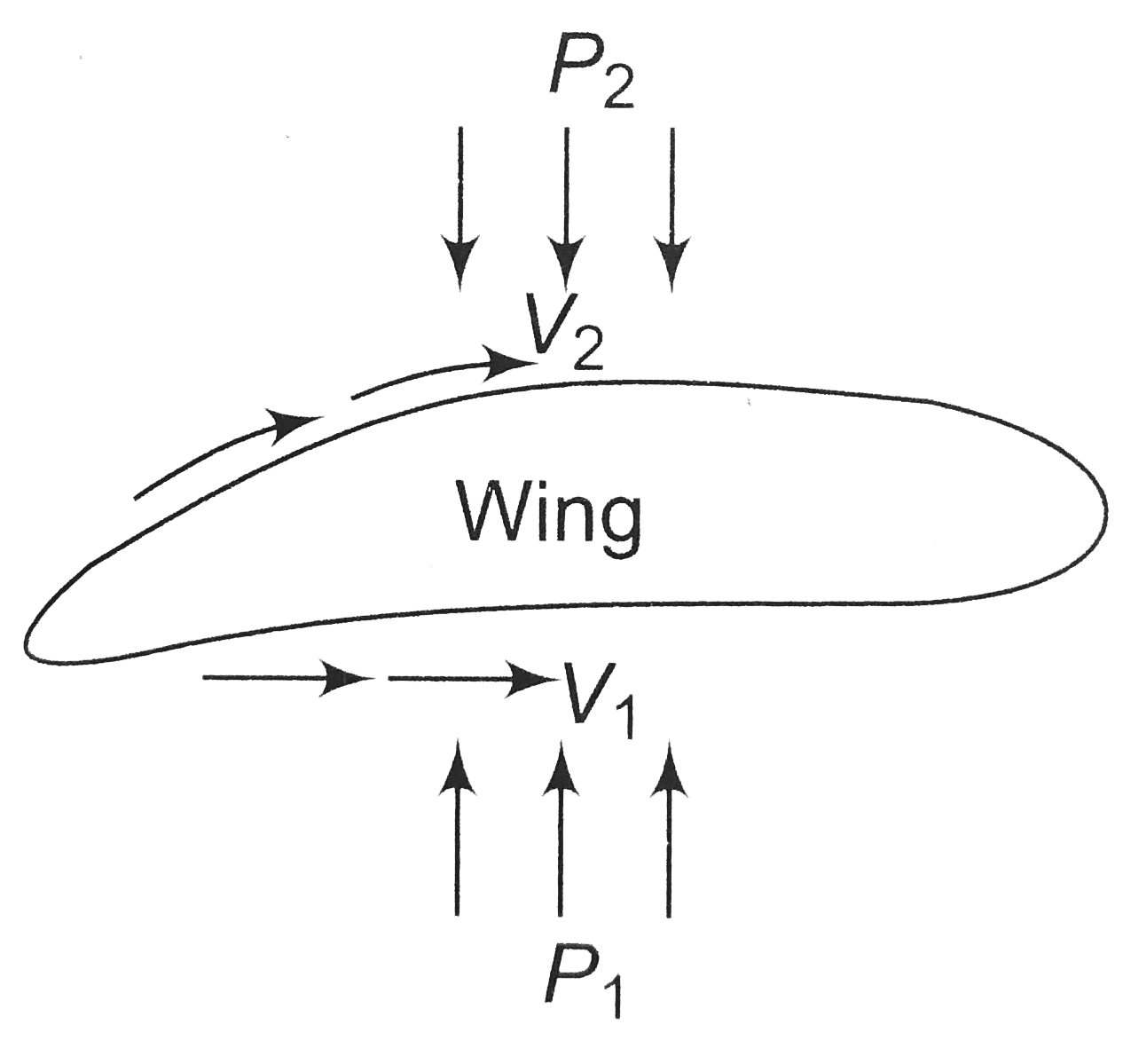
A plane is in level flight at constant speed and each of its wings has an area of 25m2. If the speed of the air is 180km/h over the upper wing surface, determine the plane's mass . (Take air density to be 1kg/m3). g=9.8m/s2.
To determine the mass of the plane in level flight, we can follow these steps:
1. Convert Speeds from km/h to m/s:
- The speed of air over the lower wing (V1) is given as 180 km/h.
- Convert V1 to m/s:
V1=180×10003600=50 m/s
- The speed of air over the upper wing (V2) is given as 234 km/h.
- Convert V2 to m/s:
V2=234×10003600=65 m/s
2. Calculate the Pressure Difference:
- Use Bernoulli's equation to find the pressure difference (P1 - P2) between the lower and upper wings:
P1−P2=12ρ(V22−V12)
- Given that air density (ρ) is 1 kg/m³, substitute the values:
P1−P2=12×1×(652−502)
- Calculate 652 and 502:
652=4225,502=2500
- Thus, the pressure difference becomes:
P1−P2=12×1×(4225−2500)=12×1×1725=862.5 Pa
3. Calculate the Total Lift Force:
- The total lift force (F) acting on the wings can be calculated using the area (A) of the wings:
F=(P1−P2)×A
- The area of each wing is given as 25 m², so for both wings:
A=2×25=50 m2
- Therefore, the lift force is:
F=862.5×50=43125 N
4. Relate Lift Force to Weight:
- In level flight, the lift force equals the weight of the plane:
F=m⋅g
- Where g=9.8 m/s2. Thus, we can find the mass (m) of the plane:
m=Fg=431259.8≈4400.51 kg
5. Final Result:
- Rounding to the nearest whole number, the mass of the plane is approximately:
m≈4400 kg
- The speed of air over the lower wing (V1) is given as 180 km/h.
- Convert V1 to m/s:
V1=180×10003600=50 m/s
- The speed of air over the upper wing (V2) is given as 234 km/h.
- Convert V2 to m/s:
V2=234×10003600=65 m/s
- Use Bernoulli's equation to find the pressure difference (P1 - P2) between the lower and upper wings:
P1−P2=12ρ(V22−V12)
- Given that air density (ρ) is 1 kg/m³, substitute the values:
P1−P2=12×1×(652−502)
- Calculate 652 and 502:
652=4225,502=2500
- Thus, the pressure difference becomes:
P1−P2=12×1×(4225−2500)=12×1×1725=862.5 Pa
- The total lift force (F) acting on the wings can be calculated using the area (A) of the wings:
F=(P1−P2)×A
- The area of each wing is given as 25 m², so for both wings:
A=2×25=50 m2
- Therefore, the lift force is:
F=862.5×50=43125 N
- In level flight, the lift force equals the weight of the plane:
F=m⋅g
- Where g=9.8 m/s2. Thus, we can find the mass (m) of the plane:
m=Fg=431259.8≈4400.51 kg
- Rounding to the nearest whole number, the mass of the plane is approximately:
m≈4400 kg
Topper's Solved these Questions
- MECHANICAL PROPERTIES OF FLUIDSBOOK - NCERT ENGLISHCHAPTER - MECHANICAL PROPERTIES OF FLUIDSEXERCISE - EXERCISE
31 Videos
- LAWS OF MOTION BOOK - NCERT ENGLISHCHAPTER - LAWS OF MOTION EXERCISE - EXERCISE
40 Videos
- MECHANICAL PROPERTIES OF SOLIDSBOOK - NCERT ENGLISHCHAPTER - MECHANICAL PROPERTIES OF SOLIDSEXERCISE - EXERCISE
21 Videos
Similar Questions
Knowledge Check
A plane is in level flight at constant speed and each of its two wings has an area of 25m2 . If the speed of the air on the upper and lower surfaces of the wing are 270kmh−1 and '234 km h^(-1)' respectively, then the mass of the plane is (Take the density of the air =1kgm−3)
A1550 kgB1750 kgC3500 kgD3200 kg
The wings of a bird and the wings of an insect are
View SolutionThe wings of a bird and the wings of an insect are
View SolutionAir stream flows horizontally past an aeroplane wing of surface area 4m2. The speed of air over the top surface is 60ms−1 and under the bottom surface is 40ms−1 The force of lift on the wing is (density of air =1kgm−3)
View SolutionThe speed of flow past the lower surface of a wing of an aeroplane is 50ms−1. What speed of flow over the upper surface will give a dynamic lift of 1000pa? Density of air =1.3kgm−3
View SolutionA cylinder rolls on a horizontal plane surface. If the speed of the centre is 25 m/s, what is the speed of the highest point?
View SolutionA fully loaded Boeing aircraft has a mass of 3.3×105kg. Its total wing area is 500m2 . It is in level flight with a speed of 960km/h.
(a) Estimate the pressure difference between the lower and upper surfaces of the wings
(b) Estimate the fractional increases in the speed of the air on the upper surfaces of the wing relative to the lower surface. The density of air is 1.2kg/m3.
View SolutionThe speed of the wind passing over the wings of a small aeroplane is 70 m/s and below the wing is 60 m/s. If the mass of the plane is 1000 kg and the area of wing is 14m2, then what will be ther net vertical force on the aeroplane ?
? (Density of air =1.2kg/m3andg=10m/s2)
View Solution
