The speed of the wind passing over the wings of a small aeroplane is 70 m/s and below the wing is 60 m/s. If the mass of the plane is 1000 kg and the area of wing is 14m2, then what will be ther net vertical force on the aeroplane ?
? (Density of air =1.2kg/m3andg=10m/s2)
2500 N
5000 N
1250 N
7500 N
The correct Answer is:B
To find the net vertical force on the aeroplane, we will follow these steps:
Step 1: Calculate the pressure difference using Bernoulli's principle.
According to Bernoulli's principle, the pressure difference between the upper and lower surfaces of the wing can be calculated using the formula:
P2−P1=12ρ(V21−V22)
Where:
- ρ = density of air = 1.2 kg/m³
- V1 = speed of air above the wing = 70 m/s
- V2 = speed of air below the wing = 60 m/s
Substituting the values:
P2−P1=12×1.2×(702−602)
Calculating 702 and 602:
702=4900and602=3600
Thus,
P2−P1=12×1.2×(4900−3600)=12×1.2×1300
Calculating further:
P2−P1=0.6×1300=780 Pascals
Step 2: Calculate the upward force due to pressure difference.
The upward force (thrust force) can be calculated using the pressure difference and the area of the wing:
F=(P2−P1)×A
Where:
- A = area of the wing = 14 m²
Substituting the values:
F=780×14=10920 Newtons
Step 3: Calculate the weight of the aeroplane.
The weight of the aeroplane can be calculated using the formula:
W=m×g
Where:
- m = mass of the aeroplane = 1000 kg
- g = acceleration due to gravity = 10 m/s²
Substituting the values:
W=1000×10=10000 Newtons
Step 4: Calculate the net vertical force.
The net vertical force Fnet acting on the aeroplane is the difference between the upward force and the weight of the aeroplane:
Fnet=F−W
Substituting the values:
Fnet=10920−10000=920 Newtons
Conclusion
The net vertical force on the aeroplane is 920 Newtons acting upwards.
---
According to Bernoulli's principle, the pressure difference between the upper and lower surfaces of the wing can be calculated using the formula:
- ρ = density of air = 1.2 kg/m³
- V1 = speed of air above the wing = 70 m/s
- V2 = speed of air below the wing = 60 m/s
The upward force (thrust force) can be calculated using the pressure difference and the area of the wing:
- A = area of the wing = 14 m²
The weight of the aeroplane can be calculated using the formula:
- m = mass of the aeroplane = 1000 kg
- g = acceleration due to gravity = 10 m/s²
The net vertical force Fnet acting on the aeroplane is the difference between the upward force and the weight of the aeroplane:
The net vertical force on the aeroplane is 920 Newtons acting upwards.
Topper's Solved these Questions
Similar Questions
The pressure at a point in water is 10 N/m2. The depth below this point where the pressure becomes double is (Given density of water = 103 kg m−3, g = 10 m s−2)
View SolutionA plane is in level flight at constant speed and each of its wings has an area of 25m2. If the speed of the air is 180km/h over the upper wing surface, determine the plane's mass . (Take air density to be 1kg/m3). g=9.8m/s2.
View Solution
Knowledge Check
A drop of water of radius 0.0015 mm is falling in air .If the cofficient of viscosity of air is 2.0×10−5kgm−1s−1 ,the terminal velocity of the drop will be
(The density of water = 103kgm−3 and g = 10ms−2 )A1. 1.0×10−4ms−1B2. 2.0×10−4ms−1C3. 2.5×10−4ms−1D4. 5.0×10−4ms−1The pressure on the top surface of an aeroplane wing is 0.8×105 Pa and the pressure on the bottom surface is 0.75×105 Pa. If the area of each surface is 50m2, the dynamic lift on the wing is
A0.5×104 NB0.25×104 NC5×104 ND25×104 N
Calculate the r.m.s velocity of air molecules at S.T.P. Density of air at S.T.P. is 1.29 kg m−3
View SolutionThe speed of flow past the lower surface of a wing of an aeroplane is 50ms−1. What speed of flow over the upper surface will give a dynamic lift of 1000pa? Density of air =1.3kgm−3
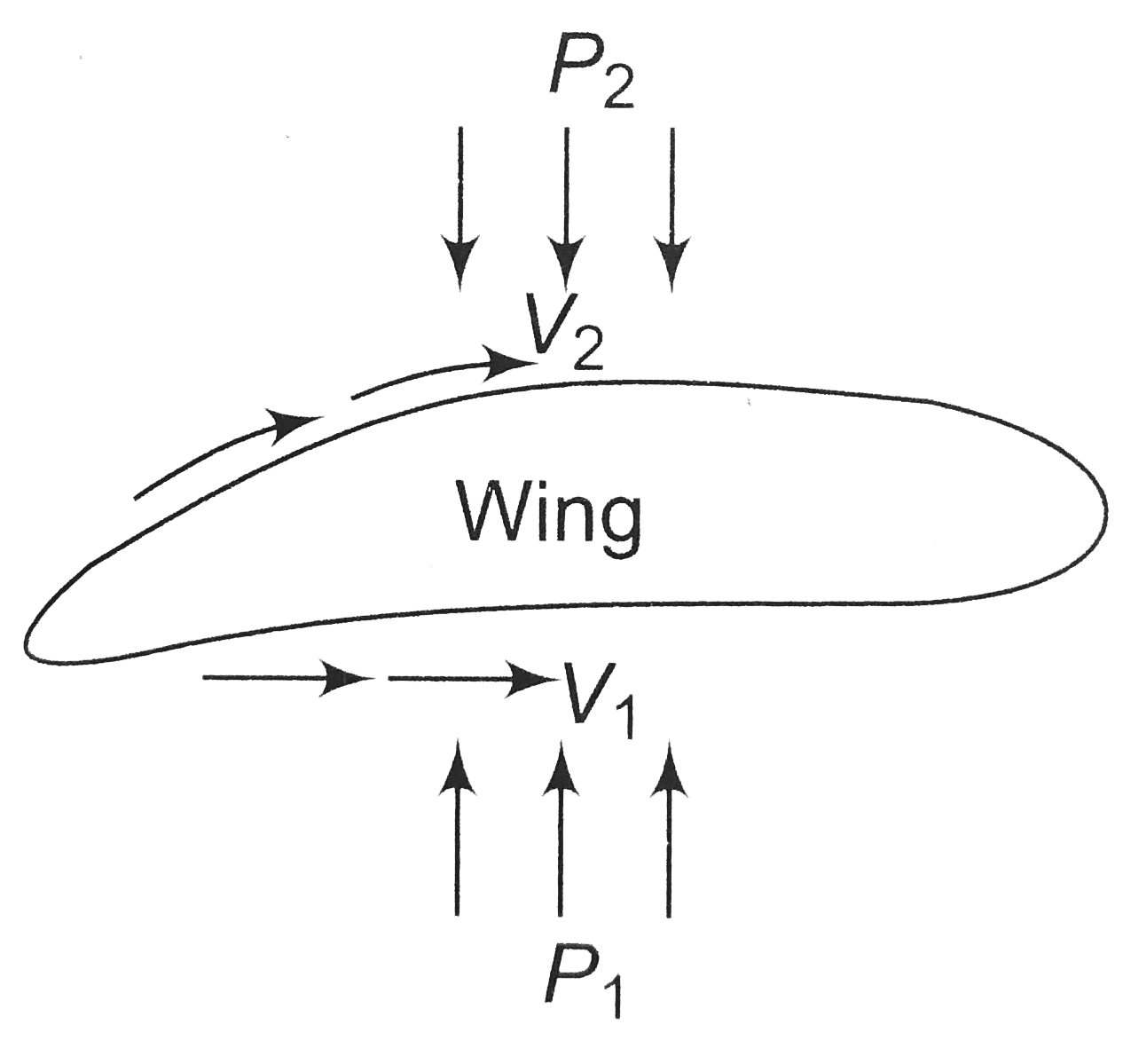
View SolutionIn a test experiment on a model aeroplane in a wind tunnel, the flow speeds on the upper and lower surfaces of the wing are 70ms−1and63ms−1 respectively. What is the lift on the wing if its area is2.5m2? Take the density of air to be1.3kgm−3
View SolutionIn a test experiment on a model aeroplane in a wind tunnel, the flow speeds on the upper and lower surfaces of the wing are 70ms−1 and 83ms−1 respectively. What is the lift on the wing, if its area is 2.5m2? Take the density of air to be 1.3kgm−3
View SolutionAir stream flows horizontally past an aeroplane wing of surface area 4m2. The speed of air over the top surface is 60ms−1 and under the bottom surface is 40ms−1 The force of lift on the wing is (density of air =1kgm−3)
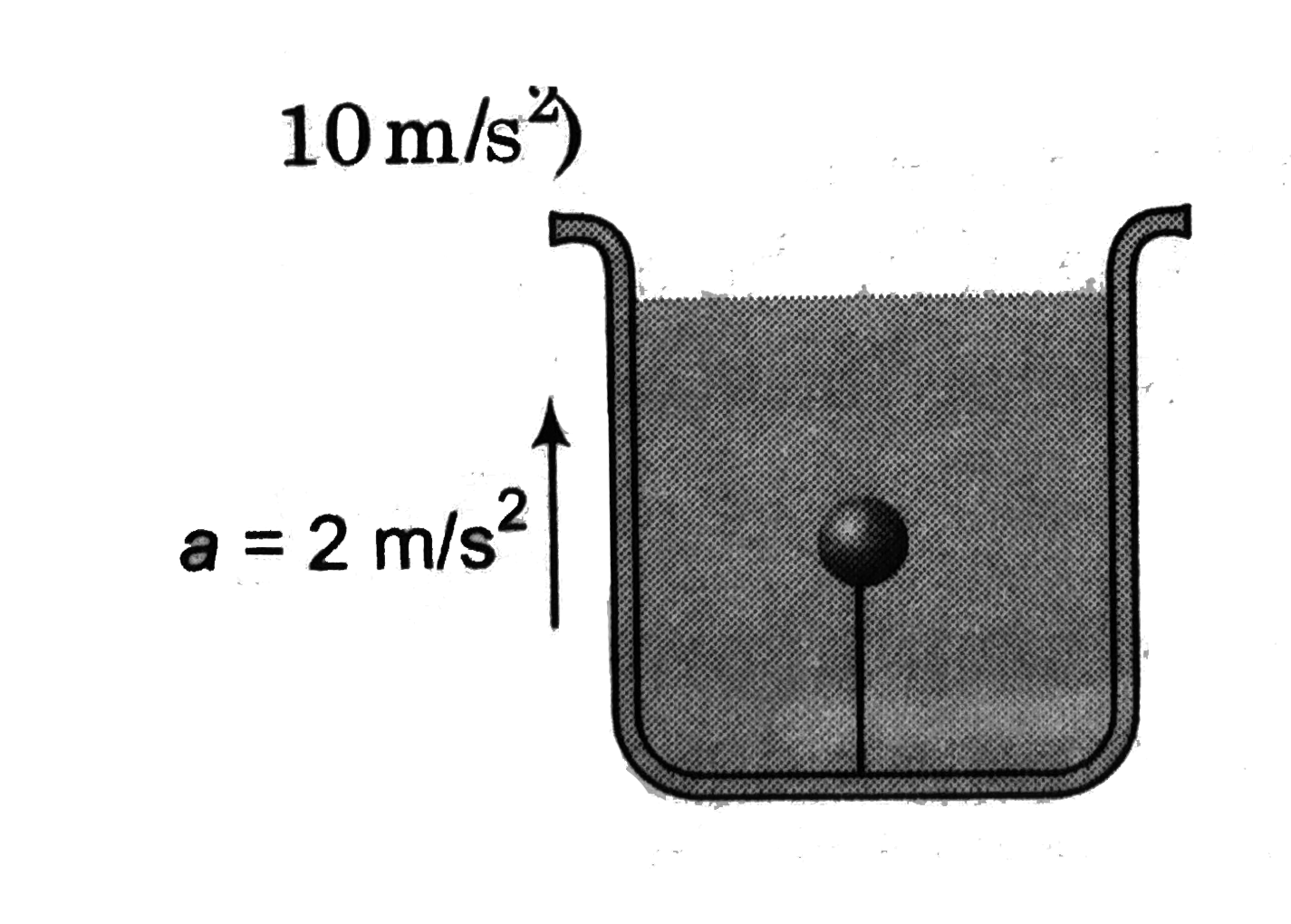
View SolutionA solid sphere of mass m=2kg and density ρ=500kg/m3 is held stationary relative to a tank filled with water. The tank is acceerating upward with acceleration 2m/s2. Calculate
(a) Tension in the thread connected between the sphere and the bottom of the tank.
(b) If the thread snaps, calculate the acceleration of sphere with respect to the tank. (Density of water=1000kg/m3,g=10m/s2)
View Solution
