A point object is above the principal axis in the concave side of a spherical interface separating medium 1 from medium 2. I f the object is in medium 1 and μ1>μ2, determine the position of the image by ray tracing.
To determine the position of the image formed by a point object above the principal axis in the concave side of a spherical interface separating two media, we can use ray tracing. Here’s a step-by-step solution:
Step 1: Draw the Principal Axis and Interface
- Begin by drawing a horizontal line to represent the principal axis.
- Draw a concave spherical interface (a circular arc) on one side of the principal axis. Label the medium on the left as medium 1 (with refractive index μ1) and the medium on the right as medium 2 (with refractive index μ2).
Hint: Remember that the concave side is the side where the center of curvature is located.
Step 2: Place the Object
- Position the point object above the principal axis on the concave side of the interface. Label this point as O.
Hint: The object should be placed at a height above the principal axis to indicate that it is above the axis.
Step 3: Draw the Rays
- Ray 1: Draw a ray from the object O that travels parallel to the principal axis. When this ray hits the interface, it will refract away from the normal because μ1>μ2. Extend this ray in the medium 2.
- Ray 2: Draw another ray from the object O that passes through the pole of the concave interface. This ray will continue in a straight line without bending.
Hint: Use the normal at the point of incidence to determine the direction of refraction for Ray 1.
Step 4: Locate the Image
- Extend the refracted ray (Ray 1) backward in medium 2. The point where this extended ray intersects the line of Ray 2 is the location of the image I.
Hint: The image formed will be virtual since it is located on the same side as the object when viewed from medium 2.
Step 5: Analyze the Image
- Determine the characteristics of the image:
- Since the object is above the principal axis, the image will also be above the principal axis.
- The image will be virtual and upright because it is formed by the divergence of rays.
Hint: Use the properties of the rays to conclude the nature of the image (real/virtual, upright/inverted).
Final Conclusion
The image is formed above the principal axis on the concave side of the spherical interface, indicating that it is virtual and upright.
- Begin by drawing a horizontal line to represent the principal axis.
- Draw a concave spherical interface (a circular arc) on one side of the principal axis. Label the medium on the left as medium 1 (with refractive index μ1) and the medium on the right as medium 2 (with refractive index μ2).
- Position the point object above the principal axis on the concave side of the interface. Label this point as O.
- Ray 1: Draw a ray from the object O that travels parallel to the principal axis. When this ray hits the interface, it will refract away from the normal because μ1>μ2. Extend this ray in the medium 2.
- Ray 2: Draw another ray from the object O that passes through the pole of the concave interface. This ray will continue in a straight line without bending.
- Extend the refracted ray (Ray 1) backward in medium 2. The point where this extended ray intersects the line of Ray 2 is the location of the image I.
- Determine the characteristics of the image:
- Since the object is above the principal axis, the image will also be above the principal axis.
- The image will be virtual and upright because it is formed by the divergence of rays.
The image is formed above the principal axis on the concave side of the spherical interface, indicating that it is virtual and upright.
Topper's Solved these Questions
- GEOMETRICAL OPTICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - GEOMETRICAL OPTICSEXERCISE - Exercise1.6
15 Videos
- GEOMETRICAL OPTICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - GEOMETRICAL OPTICSEXERCISE - Exercise1.7
21 Videos
- GEOMETRICAL OPTICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - GEOMETRICAL OPTICSEXERCISE - Exercise1.4
12 Videos
- ELECTRON,PHONTS,PHOTOELECTRIC EFFECT & X-RAYSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ELECTRON,PHONTS,PHOTOELECTRIC EFFECT & X-RAYSEXERCISE - dpp 3.3
15 Videos
- HEATING EFFECT OF CURRENTBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - HEATING EFFECT OF CURRENTEXERCISE - Thermal Power in Resistance Connected in Circuit
27 Videos
Similar Questions
An object is placed on principal axis of concave mirror at distance of 1.5 f Image will be at
View SolutionShow that for refraction at a concave spherical surface (separating glass-air medium), the distance of the object should be greater than three times the radius of curvature of the refracting surface for the image to be real.
View SolutionRefraction takes place at a convex spherical boundary separating air-glass medium. For the image to be real, the object distance (μg=3/2)
Note Object lying in the glass.
View SolutionFigure shows three transparent media of refractive indices μ1,μ2andμ3. A point object O is placed in the medium μ2. If the entire medium on the right of the spherical surface has refractive index μ1, the image forms at O'. If this entire medium has refractive index μ3 the image form at O''. In the situation shown

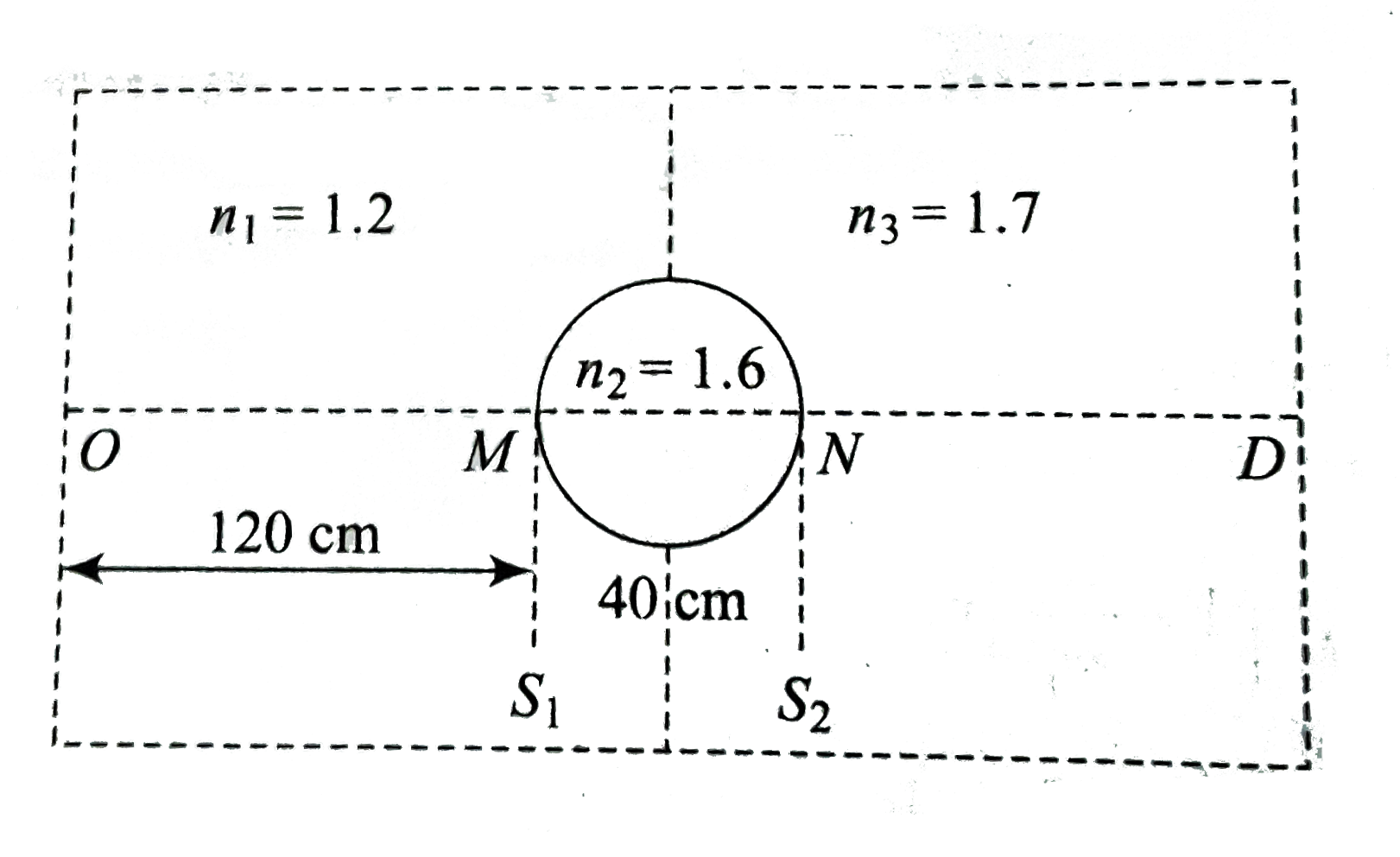
View SolutionA tranparent sphere of radius 20cm and refractive index 1.6 is fixed in a hole of the partition separating the two media: A(refractive index n1=1.2) and B ( refractive index n3=1.7). A luminous point object is placed 120cm from the surface of the sphere iin medium A. it is viewed from D in medium B in a direction normal to the sphere. Find the position of the image formed by the rays, from point N.
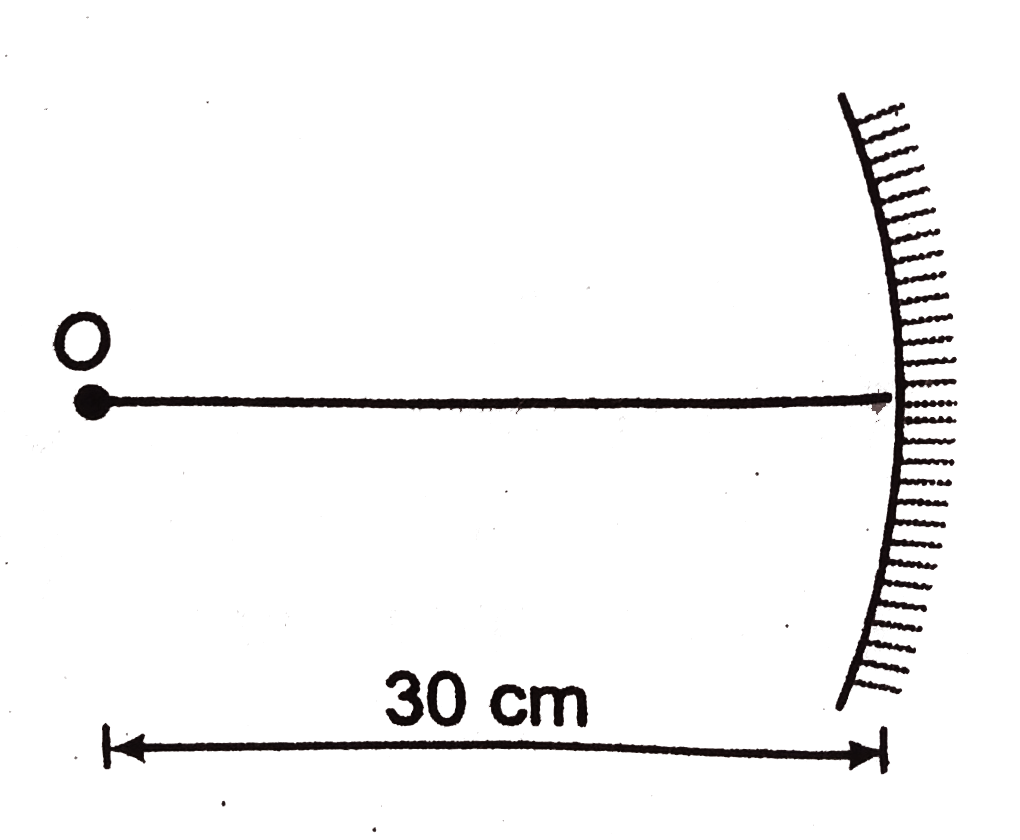
View SolutionA point object is placed on the principal axis of a concave mirror of focal length 20 cm. At this instant object is given a velocity v towards the axis (event-I) or perpendicular to axis (event-2). Then, speed of image
View SolutionA point object is placed on the principal axis of a convex lens (f = 15 cm) at a distance of 30 cm from it. A glass plate (μ=1.50) of thickness 1 cm is placed on the other side of the lens perpendicular to the axis. Locate the image of the point object.
View SolutionAn object is present on the principal axis of a concave mirror at a distance 30cm from it. Focal length of mirror is 20cm.
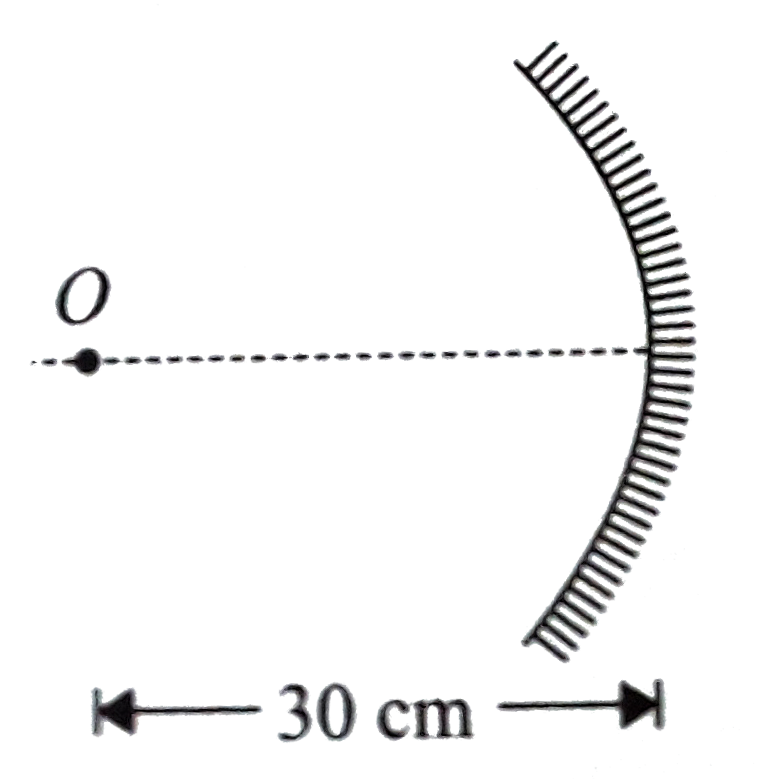
Q. If object starts moving with 2cms−1 perpendicular to principal axis above the principal axis then,
View SolutionObtain an expression for refraction at a single convex spherical surface separating the two media having refractive indices μ1(rarer medium) and μ2(denser medium) i.e., a relation between u,v,μ1,μ2 and R.
View SolutionA point object is placed 60 cm from the pole of a concave mirror of focal length 10 cm on the principal axis.
Find:
a. the position of image.
b. If the object is shifted 1 mm towards the mirror along principal axis, find the shift in image. Explain the result.
View Solution
