A point object is placed at a distance of 12cm from a convex lens of focal length 10cm. On the other side of the lens, a convex mirror is placed at a distance of 10cm from the lens such that the image formed by the combination coincides with the object itself. The focal length of the convex mirror is
20cm
25cm
15cm
30cm
The correct Answer is:B
To solve the problem step by step, we will use the lens formula and the properties of mirrors.
Step 1: Identify the given values
- Distance of the object from the lens (u) = -12 cm (the negative sign indicates that the object is on the same side as the incoming light)
- Focal length of the convex lens (f) = +10 cm (positive for convex lens)
- Distance of the convex mirror from the lens = 10 cm
Step 2: Use the lens formula to find the image distance (v)
The lens formula is given by:
1f=1v−1u
Rearranging the formula gives:
1v=1f+1u
Substituting the values:
1v=110+1−12
Calculating the right-hand side:
1v=110−112
To combine these fractions, find a common denominator (which is 60):
110=660,112=560
Thus,
1v=660−560=160
So,
v=60 cm
Step 3: Determine the distance of the image from the mirror
Since the convex mirror is placed 10 cm away from the lens, the distance of the image from the mirror (denoted as d) can be calculated as:
d=v−10=60−10=50 cm
Step 4: Use the mirror formula to find the focal length of the convex mirror
The mirror formula is given by:
1fm=1vm+1um
For the convex mirror:
- The image distance (v_m) is -50 cm (the negative sign indicates that the image is virtual and on the same side as the object).
- The object distance (u_m) is -10 cm (the object is virtual and on the same side as the incoming light).
Substituting these values into the mirror formula:
1fm=1−50+1−10
Finding a common denominator (which is 50):
1−50+1−10=−150+−550=−650
Thus,
1fm=−650⟹fm=−506=−8.33 cm
Step 5: Conclusion
The focal length of the convex mirror is approximately 8.33 cm (the negative sign indicates that it is a convex mirror).
- Distance of the object from the lens (u) = -12 cm (the negative sign indicates that the object is on the same side as the incoming light)
- Focal length of the convex lens (f) = +10 cm (positive for convex lens)
- Distance of the convex mirror from the lens = 10 cm
The lens formula is given by:
1f=1v−1u
Rearranging the formula gives:
1v=1f+1u
1v=110+1−12
Calculating the right-hand side:
1v=110−112
To combine these fractions, find a common denominator (which is 60):
110=660,112=560
Thus,
1v=660−560=160
So,
v=60 cm
Since the convex mirror is placed 10 cm away from the lens, the distance of the image from the mirror (denoted as d) can be calculated as:
d=v−10=60−10=50 cm
The mirror formula is given by:
1fm=1vm+1um
For the convex mirror:
- The image distance (v_m) is -50 cm (the negative sign indicates that the image is virtual and on the same side as the object).
- The object distance (u_m) is -10 cm (the object is virtual and on the same side as the incoming light).
1fm=1−50+1−10
Finding a common denominator (which is 50):
1−50+1−10=−150+−550=−650
Thus,
1fm=−650⟹fm=−506=−8.33 cm
The focal length of the convex mirror is approximately 8.33 cm (the negative sign indicates that it is a convex mirror).
Topper's Solved these Questions
- REFRACTION OF LIGHTBOOK - DC PANDEY ENGLISHCHAPTER - REFRACTION OF LIGHTEXERCISE - Objective Questions
1 Videos
- REFRACTION OF LIGHTBOOK - DC PANDEY ENGLISHCHAPTER - REFRACTION OF LIGHTEXERCISE - Level 1 Subjective
29 Videos
- REFRACTION OF LIGHTBOOK - DC PANDEY ENGLISHCHAPTER - REFRACTION OF LIGHTEXERCISE - Level 1 Assertion And Reason
13 Videos
- REFLECTION OF LIGHTBOOK - DC PANDEY ENGLISHCHAPTER - REFLECTION OF LIGHTEXERCISE - Subjective
9 Videos
- SEMICONDUCTORSBOOK - DC PANDEY ENGLISHCHAPTER - SEMICONDUCTORSEXERCISE - Subjective
12 Videos
Similar Questions
An object is placed at a distance of 15cm from a convex lenx of focal length 10cm. On the other side of the lens, a convex mirror is placed at its focus such that the image formed by the combination coincides with the object itself. The focal length of the convex mirror is
View SolutionAn object is placed at a distance of 12 cm from a convex lens of focal length 8 cm. Find :
the position of the image
View SolutionAn object is placed at a distance of 12 cm from a convex lens of focal length 8 cm. Find :
nature of the image
View SolutionAn object is placed at a distance of f2 from a convex lens of focal length f. The image will be
View SolutionA point object is placed at distance of 30 cm in front of a convex mirror of Focal length 30 cm. The image will form at
View SolutionAn object is placed at a distance 24 cm in front of a convex lens of focal length 8 cm.
Calculate the distance of the image from the lens.
View SolutionA point object O is placed at a distance of 15 cm from a convex lens L of focal length 10 cm as shown in figure below. On the other side of the lens, a convex mirror M is placed such that its distance from the lens is equal to the focal length of the lens. The final image formed by this combination is observed to coincide with the object 0. Find the focal length of the convex mirror.
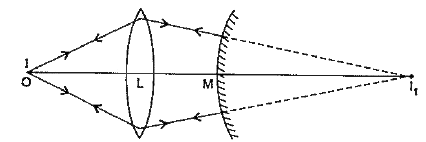
View SolutionAn object is placed at a distance 20 cm from the pole of a convex mirror of focal length 20 cm. The image is produced at
View SolutionAn object is placed at 20 cm from a convex mirror of focal length 10 cm. The image formed by a mirror is
View SolutionIn displacement method distance of object from convex lens of focal length 20 cm in one position 60 cm. Then
View Solution
