A rigid bar of mass M is supported symmetrically by three wires each of length l. Those at each end are of copper and the middle one is of iron. The ratio of their diameters, if each is to have the same tension, is equal to
√YcopperYiron
√YironYcopper
Y2ironY2copper
YironYcopper
The correct Answer is:B
To solve the problem, we need to determine the ratio of the diameters of the copper and iron wires that support a rigid bar symmetrically, given that each wire experiences the same tension.
1. Understanding Young's Modulus:
Young's modulus (Y) is defined as the ratio of stress to strain. Stress is the force per unit area, and strain is the change in length divided by the original length.
2. Expression for Stress:
For a wire, stress can be expressed as:
Stress=FA
where F is the force (tension) and A is the cross-sectional area. The area A of a circular wire can be expressed in terms of its diameter d:
A=πd24
Therefore, the stress in terms of diameter becomes:
Stress=4Fπd2
3. Relating Stress to Strain:
From Young's modulus, we have:
Y=StressStrain=4Fπd2⋅L0ΔL
Rearranging gives:
d2=4FL0πYΔL
4. Considering the Wires:
Since the tension (force) in each wire is the same and the original length L0 is the same for all wires, we can denote:
- For copper: d2c=4FL0πYcΔL
- For iron: d2i=4FL0πYiΔL
5. Finding the Ratio of Diameters:
Taking the ratio of the diameters of copper and iron:
d2cd2i=YiYc
Therefore, taking the square root gives us:
dcdi=√YiYc
6. Conclusion:
This means that the ratio of the diameters of the copper wire to the iron wire is equal to the square root of the ratio of their Young's moduli.
Final Answer:
The ratio of the diameters dcdi is given by:
dcdi=√YiYc
Young's modulus (Y) is defined as the ratio of stress to strain. Stress is the force per unit area, and strain is the change in length divided by the original length.
For a wire, stress can be expressed as:
Stress=FA
where F is the force (tension) and A is the cross-sectional area. The area A of a circular wire can be expressed in terms of its diameter d:
A=πd24
Therefore, the stress in terms of diameter becomes:
Stress=4Fπd2
From Young's modulus, we have:
Y=StressStrain=4Fπd2⋅L0ΔL
Rearranging gives:
d2=4FL0πYΔL
Since the tension (force) in each wire is the same and the original length L0 is the same for all wires, we can denote:
- For copper: d2c=4FL0πYcΔL
- For iron: d2i=4FL0πYiΔL
Taking the ratio of the diameters of copper and iron:
d2cd2i=YiYc
Therefore, taking the square root gives us:
dcdi=√YiYc
This means that the ratio of the diameters of the copper wire to the iron wire is equal to the square root of the ratio of their Young's moduli.
The ratio of the diameters dcdi is given by:
dcdi=√YiYc
Topper's Solved these Questions
- ELASTICITYBOOK - DC PANDEY ENGLISHCHAPTER - ELASTICITYEXERCISE - Match the columns
4 Videos
- ELASTICITYBOOK - DC PANDEY ENGLISHCHAPTER - ELASTICITYEXERCISE - Medical entrances s gallery
21 Videos
- ELASTICITYBOOK - DC PANDEY ENGLISHCHAPTER - ELASTICITYEXERCISE - Check point 12.3
15 Videos
- CURRENT ELECTRICITYBOOK - DC PANDEY ENGLISHCHAPTER - CURRENT ELECTRICITYEXERCISE - All Questions
469 Videos
- ELECTROSTATICSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATICSEXERCISE - Integer
17 Videos
Similar Questions
A rigid bar of mass 15 kg is supported symmetrically by three wires each 2 m long. Those at each end are of copper and middle one is of iron. Determine the ratio of their diameters if each is to have the same tension. Young's modulus of elasticity for copper and steel are 110×109Nm−2 and 190×109Nm−2 respectively.
View SolutionA rigid bar of mass 15 kg is supported symmetrically by three wires, each of length 2 m. The wires at the endpoints are made of copper and the middle one is made of steel. If the tension in each wire is the same, then the diameter of copper wire to the diameter of steel wire is [Given, Ycopper=1.1×1011N m−1andYsteel=1.9×1011N m−2]
View SolutionTwo wires, one of copper and the other of iron, are of the same length and same radius. Which will have more resistance ? Give reason.
View SolutionTwo wires of equal length one of copper and other of manganin have the same resistance. Which wire is thicker?
View SolutionThree identical rods, each of mass m and length l, form an equaliteral triangle. Moment of inertia about one of the sides is

View SolutionA homogeneous block with a mass m hangs on three vertical wires of equal length arranged symmetrically . Find the tensions of the wires if the middle wire is of steel and the other two are of copper . All the wires have the same cross - section . Consider the modulus of elasticity of steel to be double than that of copper .
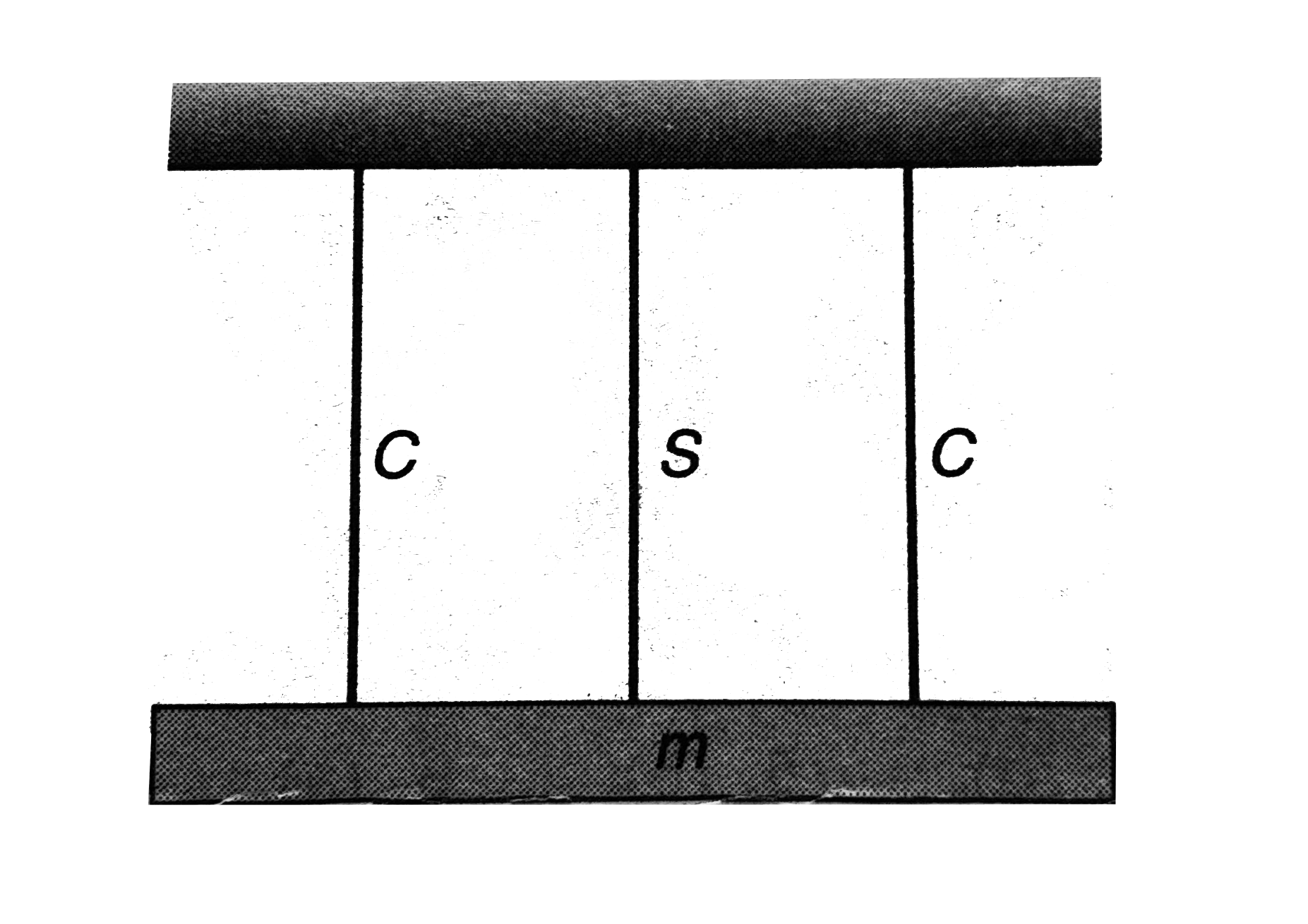
View SolutionA metal wire of length L is suspended vertically from a rigid support. When a bob of mass M is attached to the lower end of wire, the elongation of the wire is l:
View SolutionThree point charges, each with charge q and mass 'm', are connected by three strings of equal length l whose other end is connected to a fixed point P. They form an equilateral triangle of side 'a' in a horizontal plane. The tension in each string will be
View SolutionA heavy plank of mass 100 kg hangs on three vertical wires of equal length arranged symmetrically (see figure). All the wires have the same cross - section.
The middle wire is of steel and the other two are of copper . The modulus of elasticity of steel is assumed to be double that copper. Determine the tensions in the wire. (use g=9.8ms−2 )

View SolutionThree thin rods each of length Land mass M are placed along x, y and z-axes such that one end of each rod is at origin. The moment of inertia of this system about z-axis is
View Solution
