A river is flowing due east with a speed (Fig. 2 (EP) .31 ).
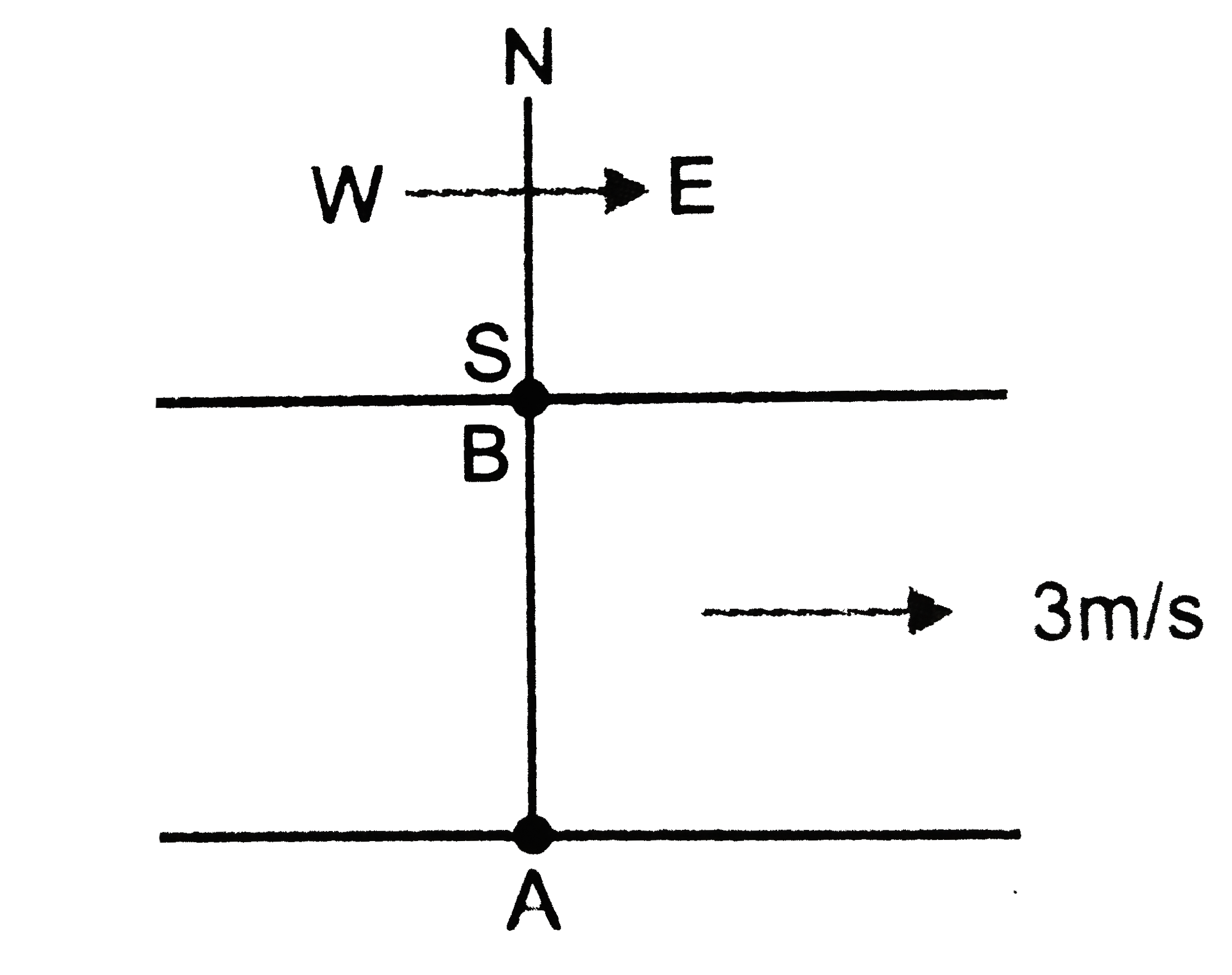 . (a) If swimmer starts swimming due north, what will be his resultant velocity (magnitude and direction) ? (b) If he wants to start from point (A) on South bank and reach opposite point (B) on North bank,
. (a) If swimmer starts swimming due north, what will be his resultant velocity (magnitude and direction) ? (b) If he wants to start from point (A) on South bank and reach opposite point (B) on North bank,
(i) Which direction should he swim? (ii) What will be his resultant speed ? (c ) From two differenrent casses as mentioned in (a) and 9b) above, in which casse will he reach opposite bank in shorter time ?
Given , Speed of the river =3 m/s (east)
Speed of swimmer (east)
(a) When swimmer starts swimming due north then his resultant velocity
Hence,
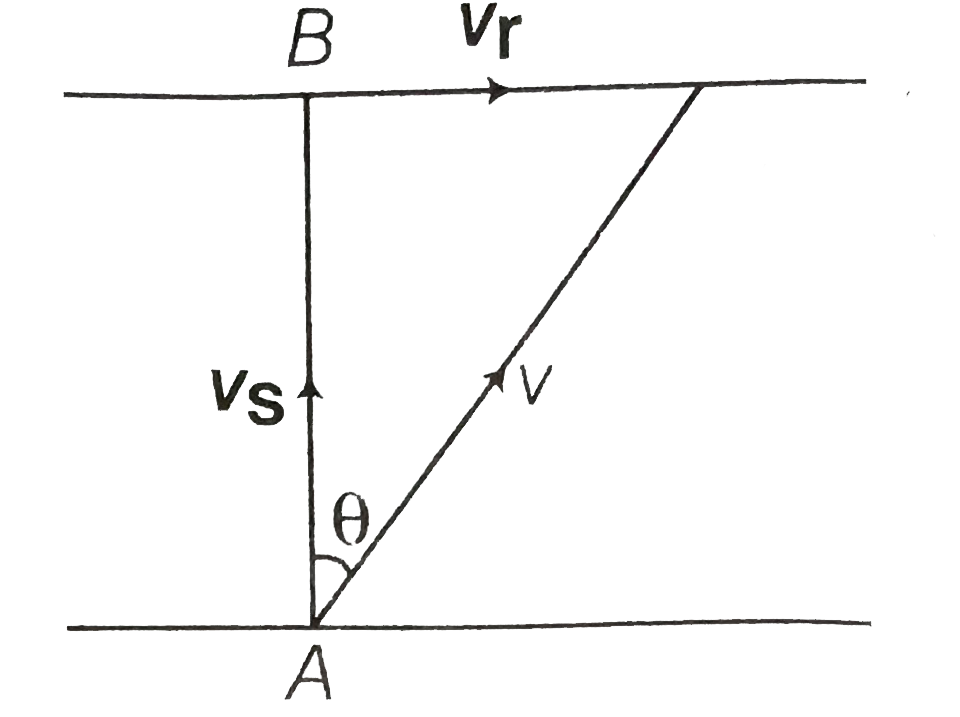
(b) To reach opposite points B, the swimmer should swim at an angle of north.
Resultant speed of the swimmer
of north
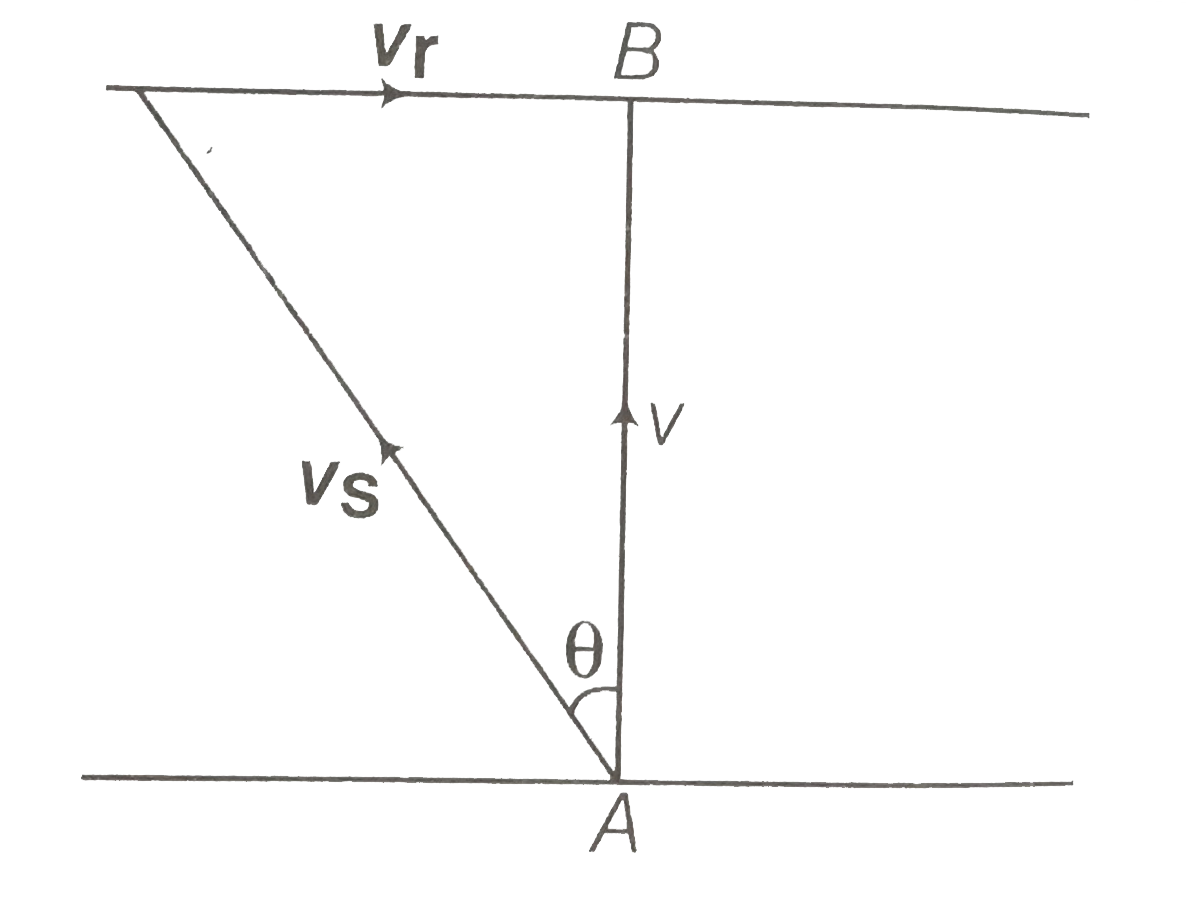
(c) In case (a),
Time taken by the swimmer to cross the river ,
In case (d) ,
Time taken by the swimmer to cross the river
As , therefore
Hence , the swimmer will cross the river in shorter time in case (a).
Topper's Solved these Questions
Similar Questions
A river is flowing due east with a speed 3ms−1 A swimmer can swim in still water at a speed of 4ms−1 If swimmer starts swimming due north what will be his resultant velocity (magnitude and direction )?
View SolutionA river is flowing from west to east with a speed 5m/s. A swimmer can swim in still water at a speed of 10m/s .
If he wants to start from point A on south bank and reach opposite point B on north bank. In what direction direction should he swim ?
View Solution
Knowledge Check
A river is flowing due east with a speed 3ms−1. A swimmer can swim in still water at a speed of 4ms−1. If swimmer starts swimming due north, then the resultant velocity of the swimmer is a) 3 m/s b) 5 m/s c) 7 m/s d) 2 m/s
A3 m s−1B5 m s−1C7 m s−1D2 m s−1
In a lake, stream direction is as shown in the figure. A man starting from the point P on the bank wants to move to the otherside of the bank in shortest time .Then direction in which he should swim
View SolutionA river is flowing from west to east with a speed of 5m/min. A man can swim in still water with a velocity 10m/min. In which direction should the man swim so as to take the shortest possible path to go to the south.
View SolutionVelocity of a boat relative to river current and river current velocity are equal in magnitude. Starting from point A on the southern bank, the boatman wants to reach a point B on the nothern bank in north−east from A. In which direction he has to steer the boat?

View SolutionA man can swim at the rate of 5km/h in still water. A river 1km wide flows at the rate of 3kmh−1. A swimmer wishes to swim across the river directly opposite to the starting point.
(a) Along what direction must the man swim ?
(b) What should be his resultant velocity ?
( c) How much time will he take to cross the river ?
View SolutionA man wants to reach point B on the opposite bank of a river flowing at a speed as shown in figure. What minimum speed relative to water should the man have so that he can reach point B? In which direction should he swim?
View SolutionA river is flowing from west to east at a speed of 5m/s. A man on the south bank of the river capable of swimming at 10m/s in a still water wants to swim, across the river in a shortest time. He should swim in a direction
View SolutionA river is flowing from east to west at a speed of 5mmin. A man on south bank of river, capable of swimming 10mmin ini still water, wants to swim across the river in shortest time. He should swim
View Solution
