Question

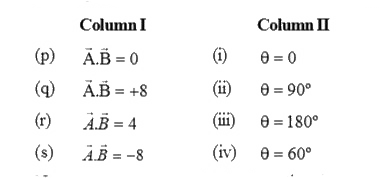
If $\left|\overrightarrow{A}\right|=2\text{and}\left|\overrightarrow{B}\right|=4$, then match the relations in column I with the angle$\theta$ between $\overrightarrow{A}\text{and}\overrightarrow{B}$ in column II.
Column I, Column II
(a) $|\overrightarrow{A}\times \overrightarrow{B}|=0$ , (i) $\theta ={30}^{\circ }$
(b) $|\overrightarrow{A}\times \overrightarrow{B}|=0$ , (ii) $\theta ={45}^{\circ }$
(c ) $\overrightarrow{A}\times \overrightarrow{B}\mid =4$ , (iii) $\theta ={90}^{\circ }$
(d) $|\overrightarrow{A}\times x\overrightarrow{B}|=4\sqrt{2}$ , (iv) $\theta =0^{\circ }$.

Explanation

To solve the problem, we need to analyze the cross product of two vectors $$\overrightarrow{A}$$ and $$\overrightarrow{B}$$ given their magnitudes and find the corresponding angles between them based on the conditions provided in Column I.

1. Understanding the Cross Product:
The magnitude of the cross product of two vectors \( \vec{A} \) and \( \vec{B} \) is given by the formula:
\( |\vec{A} \times \vec{B}| = |\vec{A}| |\vec{B}| \sin \theta \)
where \( \theta \) is the angle between the two vectors.

2. Given Magnitudes:
We know:
\( |\vec{A}| = 2 \quad \text{and} \quad |\vec{B}| = 4 \)

3. Analyzing Each Case:
We will analyze each case from Column I and find the corresponding angle \( \theta \) from Column II.

(a) \( |\vec{A} \times \vec{B}| = 0 \):
- Since the cross product is zero, it implies that \( \sin \theta = 0 \).
- This occurs when \( \theta = 0^\circ \) or \( \theta = 180^\circ \).
- Match: (a) → (iv) \( \theta = 0^\circ \)

(b) \( |\vec{A} \times \vec{B}| = 8 \):
- Using the formula:
\( 8 = 2 \times 4 \times \sin \theta \implies 8 = 8 \sin \theta \implies \sin \theta = 1 \)
- This occurs when \( \theta = 90^\circ \).
- Match: (b) → (iii) \( \theta = 90^\circ \)

(c) \( |\vec{A} \times \vec{B}| = 4 \):
- Using the formula:
\( 4 = 2 \times 4 \times \sin \theta \implies 4 = 8 \sin \theta \implies \sin \theta = \frac{1}{2} \)
- This occurs when \( \theta = 30^\circ \).
- Match: (c) → (i) \( \theta = 30^\circ \)

(d) \( |\vec{A} \times \vec{B}| = 4\sqrt{2} \):
- Using the formula:
\( 4\sqrt{2} = 2 \times 4 \times \sin \theta \implies 4\sqrt{2} = 8 \sin \theta \implies \sin \theta = \frac{\sqrt{2}}{2} \)
- This occurs when \( \theta = 45^\circ \).
- Match: (d) → (ii) \( \theta = 45^\circ \)

Final Matches:
- (a) → (iv) \( \theta = 0^\circ \)
- (b) → (iii) \( \theta = 90^\circ \)
- (c) → (i) \( \theta = 30^\circ \)
- (d) → (ii) \( \theta = 45^\circ \)