A rod of length L is pivoted at one end and is rotated with as uniform angular velocity in a horizontal plane. Let T1andT2 be the tensions at the points L/4 and 3L/4 away from the pivoted ends.
T1>T2
T2>T1
T1=T2
TherelationbetweenT_1 and T_2 depends on whether the rod rotates clockwise or anticlockwise.
The correct Answer is:A
To solve the problem of comparing the tensions T1 and T2 at points L4 and 3L4 respectively on a rotating rod, we can follow these steps:
Step 1: Understand the System
The rod of length L is pivoted at one end and rotates with a uniform angular velocity ω. Each point on the rod experiences a centripetal acceleration due to this rotation.
Step 2: Define the Tensions
Let T1 be the tension at the point L4 from the pivot, and T2 be the tension at the point 3L4 from the pivot.
Step 3: Consider a Small Element
Take a small element dx of the rod at a distance x from the pivot. The mass of this small element can be expressed as dm=MLdx, where M is the total mass of the rod.
Step 4: Apply Newton's Second Law
For the small element dx at distance x, the net force acting on it in the radial direction (towards the pivot) is given by:
T1−T2=dm⋅ω2⋅x
Substituting dm:
T1−T2=(MLdx)⋅ω2⋅x
Step 5: Analyze the Relationship
From the equation T1−T2=Mω2Lxdx, we can see that as x increases, T1 must be greater than T2 because the term Mω2Lxdx is positive. This indicates that tension decreases as we move away from the pivot.
Step 6: Compare Tensions at Specific Points
Now, we need to compare T1 (at L4) and T2 (at 3L4):
- Since L4<3L4, we conclude that T1>T2.
Conclusion
Thus, the tension at L4 (i.e., T1) is greater than the tension at 3L4 (i.e., T2):
T1>T2
---
The rod of length L is pivoted at one end and rotates with a uniform angular velocity ω. Each point on the rod experiences a centripetal acceleration due to this rotation.
Let T1 be the tension at the point L4 from the pivot, and T2 be the tension at the point 3L4 from the pivot.
Take a small element dx of the rod at a distance x from the pivot. The mass of this small element can be expressed as dm=MLdx, where M is the total mass of the rod.
For the small element dx at distance x, the net force acting on it in the radial direction (towards the pivot) is given by:
T1−T2=dm⋅ω2⋅x
Substituting dm:
T1−T2=(MLdx)⋅ω2⋅x
From the equation T1−T2=Mω2Lxdx, we can see that as x increases, T1 must be greater than T2 because the term Mω2Lxdx is positive. This indicates that tension decreases as we move away from the pivot.
Now, we need to compare T1 (at L4) and T2 (at 3L4):
- Since L4<3L4, we conclude that T1>T2.
Thus, the tension at L4 (i.e., T1) is greater than the tension at 3L4 (i.e., T2):
T1>T2
Topper's Solved these Questions
- CIRCULAR MOTIONBOOK - HC VERMA ENGLISHCHAPTER - CIRCULAR MOTIONEXERCISE - Objective -2
6 Videos
- CIRCULAR MOTIONBOOK - HC VERMA ENGLISHCHAPTER - CIRCULAR MOTIONEXERCISE - Exercises
30 Videos
- CIRCULAR MOTIONBOOK - HC VERMA ENGLISHCHAPTER - CIRCULAR MOTIONEXERCISE - worked out Examples
13 Videos
- CENTRE OF MASS, LINEAR MOMENTUM, COLLISIONBOOK - HC VERMA ENGLISHCHAPTER - CENTRE OF MASS, LINEAR MOMENTUM, COLLISIONEXERCISE - Objective -2
11 Videos
- FLUID MECHANICSBOOK - HC VERMA ENGLISHCHAPTER - FLUID MECHANICSEXERCISE - All Questions
90 Videos
Similar Questions
A uniform rod mass m and length l is provided at one end and is rotated with constant angular velocity ω in a horizontal plane. Tension in the rod at a distance (√3l/2) from pivoted end is (Assume gravity free space)
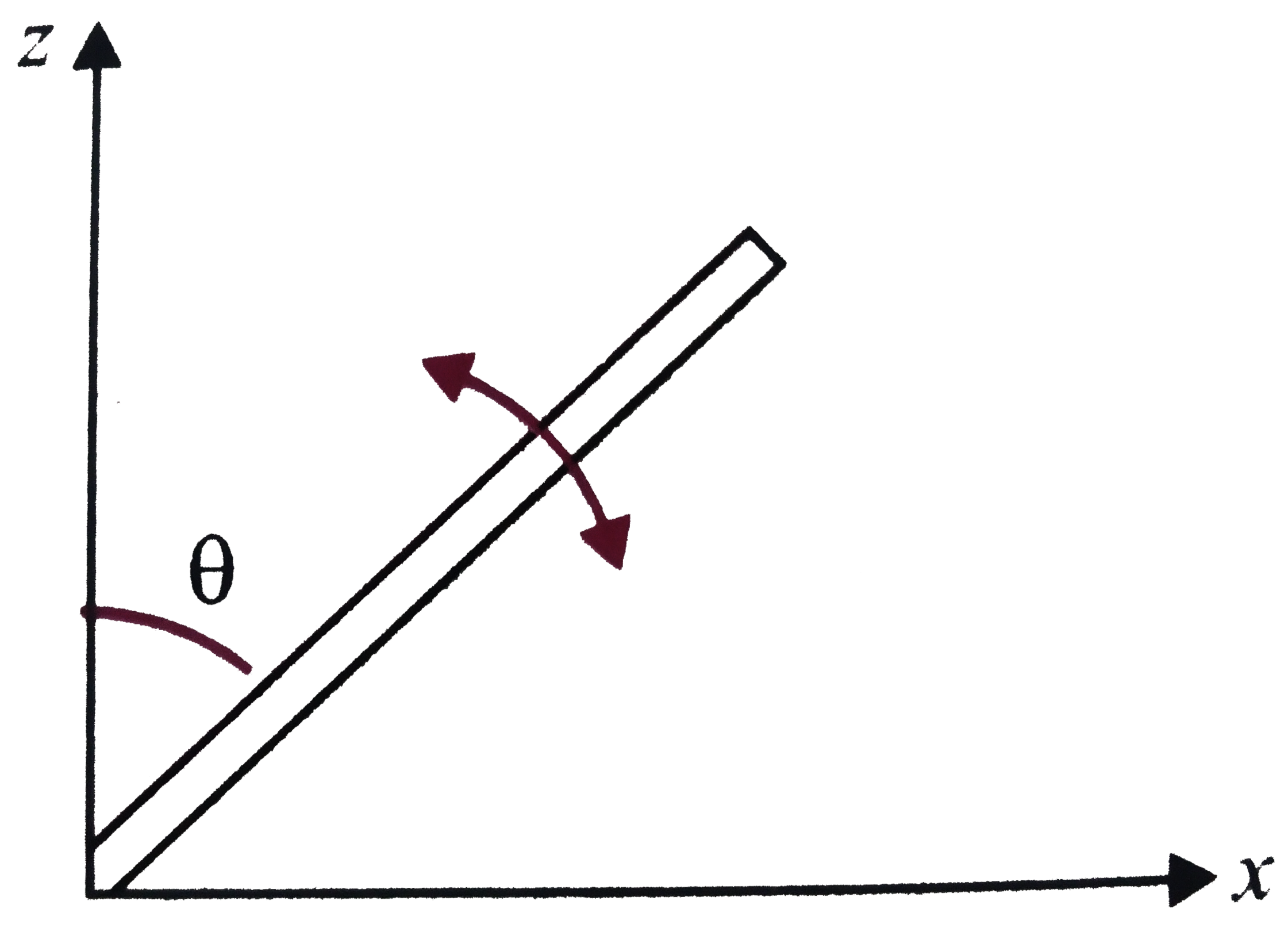
View SolutionA slender uniform rod of mass M and length l is pivoted at one end so that it can rotate in a vertical plane, Fig. There is negligible friction at the pivot. The free end is held vertically above the pivot and then released. The angular acceleration of the rod when it makes an angle θ with the vertical is
View SolutionA thin uniform copper rod of length l and mass m rotates uniformly with an angular velocity ω in a horizontal plane about a vertical axis passing through one of its ends. Determine the tension in the rod as a function of the distance r from the rotation axis. Find the elongation of the rod.
View SolutionA stick of mass m and length l is pivoted at one end and is displaced through an angle θ. The increase in potential energy is
View SolutionA uniform rod of mass m and length l rotates in a horizontal plane with an angular velocity ω about a vertical axis passing through one end. The tension in the rod at a distance x from the axis is
View SolutionA conducting rod OA of length l is rotated about its end O with an angular velocity ω in a uniform magnetic field directed perpendicualr to the rotation. Find the emf induced in the rod, between it's ends.
View SolutionA copper rod AB-of length / pivoted at one end A, rotates at constant angular velocity ω, at right angles to a uniformn magnetic field of induction B. The emf, developed between the mid point of the rod and end B is
View SolutionA stone is tied to one end of a string and rotated in horizontal circle with a uniform angular velocity. The tension in the string is T, if the length of the string is halved and its angular velocity is doubled , the tension in the string will be
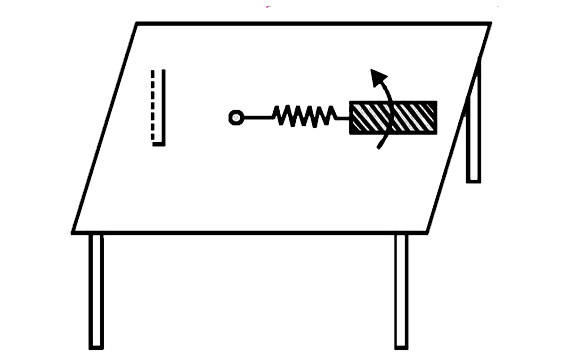
View SolutionA metallic rod of length 'l' is tied to a string of length 2l and made to rotate with angular speed w on a horizontal table with one end of the string fixed. If there is a vertical magnetic field 'B' in the region, the e.m.f. Induced across the ends of the rod is
View SolutionA thin uniform rod of length l is pivoted at its upper end. It is free to swing in a vertical plane. Its time period for oscillation of small amplitude is
View Solution
