A rod of mass $M k g$ and length $L m$ is bent in the from of an equilateral tringle as shown in Gig. The moment of inertia of the triangle about a vertical axis perpendicular to the plane of the triangle and passing through the centre (in units of $k g m^{2}$ ) is.
.

A

$\frac{M L^{2}}{12}$

B

$\frac{M L^{2}}{54}$

C

$\frac{M L^{2}}{162}$

D

$\frac{M L^{2}}{108}$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:B

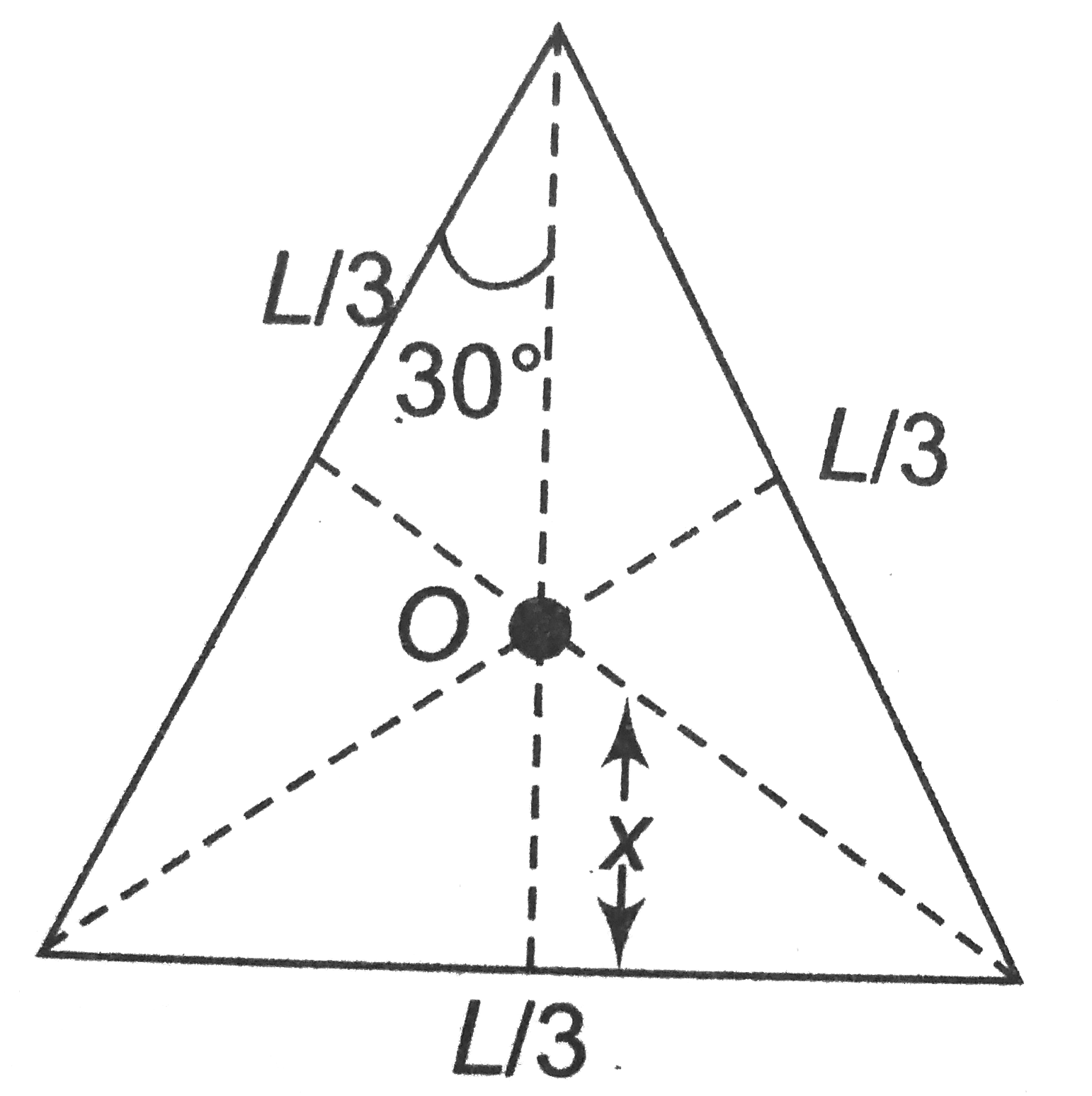
(b) Mass of each side $= \frac{M}{3}$
$x = \frac{1}{3} [\frac{L}{3} {cos 30}^{\circ}] = \frac{L / 3}{2 \sqrt{3}}$
moment of interia of one side about $O$ :
$I = [\frac{1}{12} \frac{M}{3} {(\frac{L}{3})}^{2} + \frac{M}{3} x^{2}] = \frac{M L^{2}}{162}$
moment of interia of whole triangle about
$O : 3 I = \frac{M L^{2}}{54}$ .
.

|

Updated on:21/07/2023

Class 11 PHYSICS RIGID BODY DYNAMICS

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
A rod of mass $M k g$ and length $L m$ is bent in the from of an equilateral tringle as shown in Gig. The moment of inertia of the triangle about a vertical axis perpendicular to the plane of the triangle and passing through the centre (in units of $k g m^{2}$ ) is.
.
A $\frac{M L^{2}}{12}$
B $\frac{M L^{2}}{54}$
C $\frac{M L^{2}}{162}$
D $\frac{M L^{2}}{108}$
Question 2 - Select One
Moment of inertia of a thin rod of mass 'M' and length 'L' about the axis perpendicular to the rod and passing through its centre is ............
A $M L^{\frac{2}{4}}$
B $M L^{\frac{2}{6}}$
C $M L^{\frac{2}{12}}$
D $M L^{\frac{2}{2}}$
Question 3 - Select One
A thin rod of length $L$ of mass $M$ is bent at the middle point $O$ at an angle of $60^{\circ}$ . The moment of inertia of the rod about an axis passing through $O$ and perpendicular to the plane of the rod will be

A $\frac{M L^{2}}{6}$
B $\frac{M L^{2}}{12}$
C $\frac{M L^{2}}{24}$
D $\frac{M L^{2}}{3}$

A2Z-ROTATIONAL DYNAMICS-Moment Of Inertia

Home
Ask Doubt
Profile