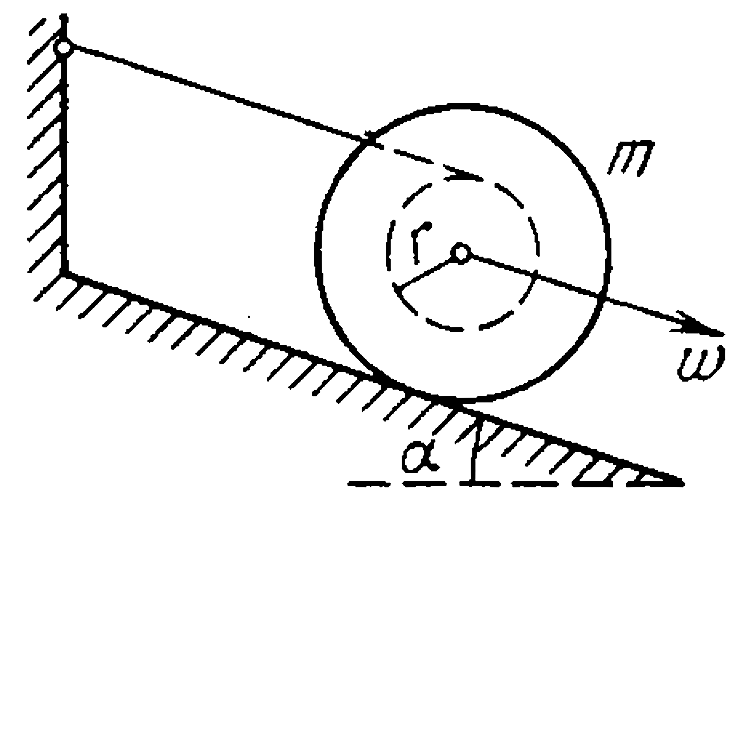
A spool with thread wound on it, of mass m, rests on a rough horizontal surface. Its moment of inertia relative to its own axis is equal to $I = γ m R^{2}$ , where $γ$ is a numerical factor, and R is the outside radius of the spool. The radius of the wound thread layer is equal to r. The spool is pulled without sliding by the thread with a constant force F directed at an angle $α$ to the horizontal (figure). Find:
(a) the projection of the acceleration vector of the spool axis on the x-axis,
(b) the work performed by the force F during the first t seconds after the beginning of motion.

Video Solution

Text Solution

Verified by Experts

The correct Answer is: $⎡ ⎢ ⎢ ⎣ \frac{F (cos θ - \frac{r}{R})}{m (1 + γ)}, \frac{F^{2} t^{2} (cos θ - \frac{r}{R})}{2 m (1 + γ)} ⎤ ⎥ ⎥ ⎦$

Answer

Step by step video, text & image solution for A spool with thread wound on it, of mass m, rests on a rough horizontal surface. Its moment of inertia relative to its own axis is equal to I=gammamR^2, where gamma is a numerical factor, and R is the outside radius of the spool. The radius of the wound thread layer is equal to r. The spool is pulled without sliding by the thread with a constant force F directed at an angle alpha to the horizontal (figure). Find: (a) the projection of the acceleration vector of the spool axis on the x-axis, (b) the work performed by the force F during the first t seconds after the beginning of motion. by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.

Updated on:21/07/2023

Class 11 PHYSICS RIGID BODY DYNAMICS

Topper's Solved these Questions

Knowledge Check

Question 1 - Select One
A solid cylinder of mass M and radius R rolls without slipping on a flat horizontal surface. Its moment of inertia about the line of contact is ?
A $\frac{M R^{2}}{2}$
B $M R^{2}$
C $\frac{3}{2} M R^{2}$
D $2 M R^{2}$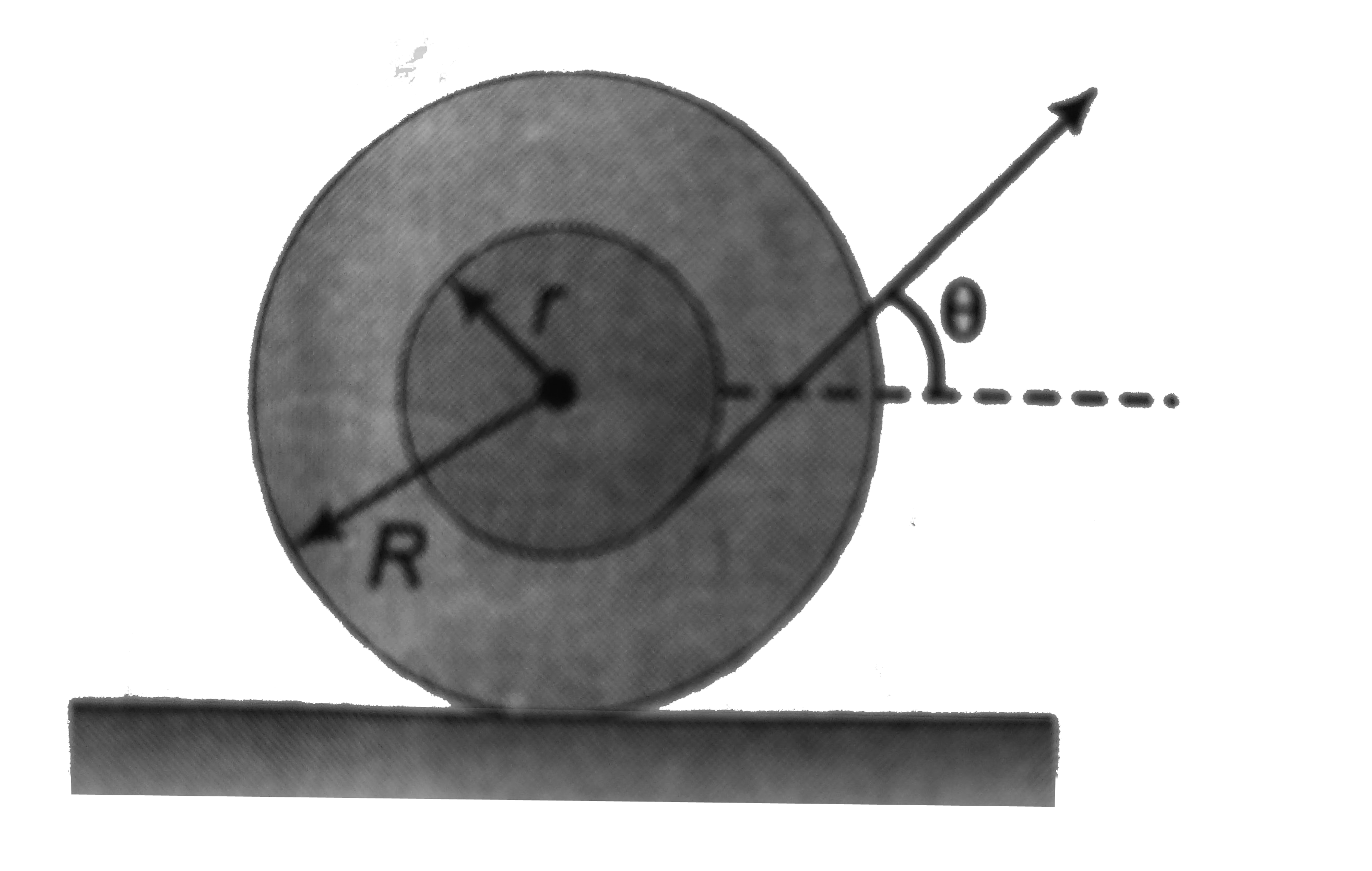
Question 2 - Select One

A spool is pulled at an angle $θ$ with the horizontal on a rough horizontal surface as shown in the figure. If the spool remains at rest, the angle $θ$ is equal to
A ${cos}^{- 1} (\frac{R}{r})$
B ${sin}^{- 1} (\sqrt{1 - \frac{r^{2}}{R^{2}}})$
C $π - {cos}^{- 1} (\frac{r}{R})$
D ${sin}^{- 1} (\frac{r}{R})$
Question 3 - Select One
If the moment of inertia of a rigid body is numerically equal to its mass, radius of gyration is
Aequal to its radius
Bequal to diameter
Cequal to one unit
Dinfinite

PHYSICS GALAXY - ASHISH ARORA-RIGID BODIES AND ROTATIONAL MOTION-Unsolved Numerical

Home
Ask Doubt
Profile

The correct Answer is:⎡⎢ ⎢⎣F(cosθ−rR)m(1+γ),F2t2(cosθ−rR)2m(1+γ)⎤⎥ ⎥⎦

Topper's Solved these Questions

Similar Questions

Knowledge Check

A solid cylinder of mass M and radius R rolls without slipping on a flat horizontal surface. Its moment of inertia about the line of contact is ?

A spool is pulled at an angle θ with the horizontal on a rough horizontal surface as shown in the figure. If the spool remains at rest, the angle θ is equal to

If the moment of inertia of a rigid body is numerically equal to its mass, radius of gyration is

PHYSICS GALAXY - ASHISH ARORA-RIGID BODIES AND ROTATIONAL MOTION-Unsolved Numerical

The correct Answer is: $⎡ ⎢ ⎢ ⎣ \frac{F (cos θ - \frac{r}{R})}{m (1 + γ)}, \frac{F^{2} t^{2} (cos θ - \frac{r}{R})}{2 m (1 + γ)} ⎤ ⎥ ⎥ ⎦$

A spool is pulled at an angle $θ$ with the horizontal on a rough horizontal surface as shown in the figure. If the spool remains at rest, the angle $θ$ is equal to