A spool of inner radius R and outer radius 3R has a moment of inertia =MR2 about an axis passing through it's geometric centre, where M is the mass of the spool. A thread wound on the inner surface of the spool is pulled horizontally with a constant force =Mg. Find the acceleration of the point on which is being pulled assuming that the spool rolls purely on the floor.
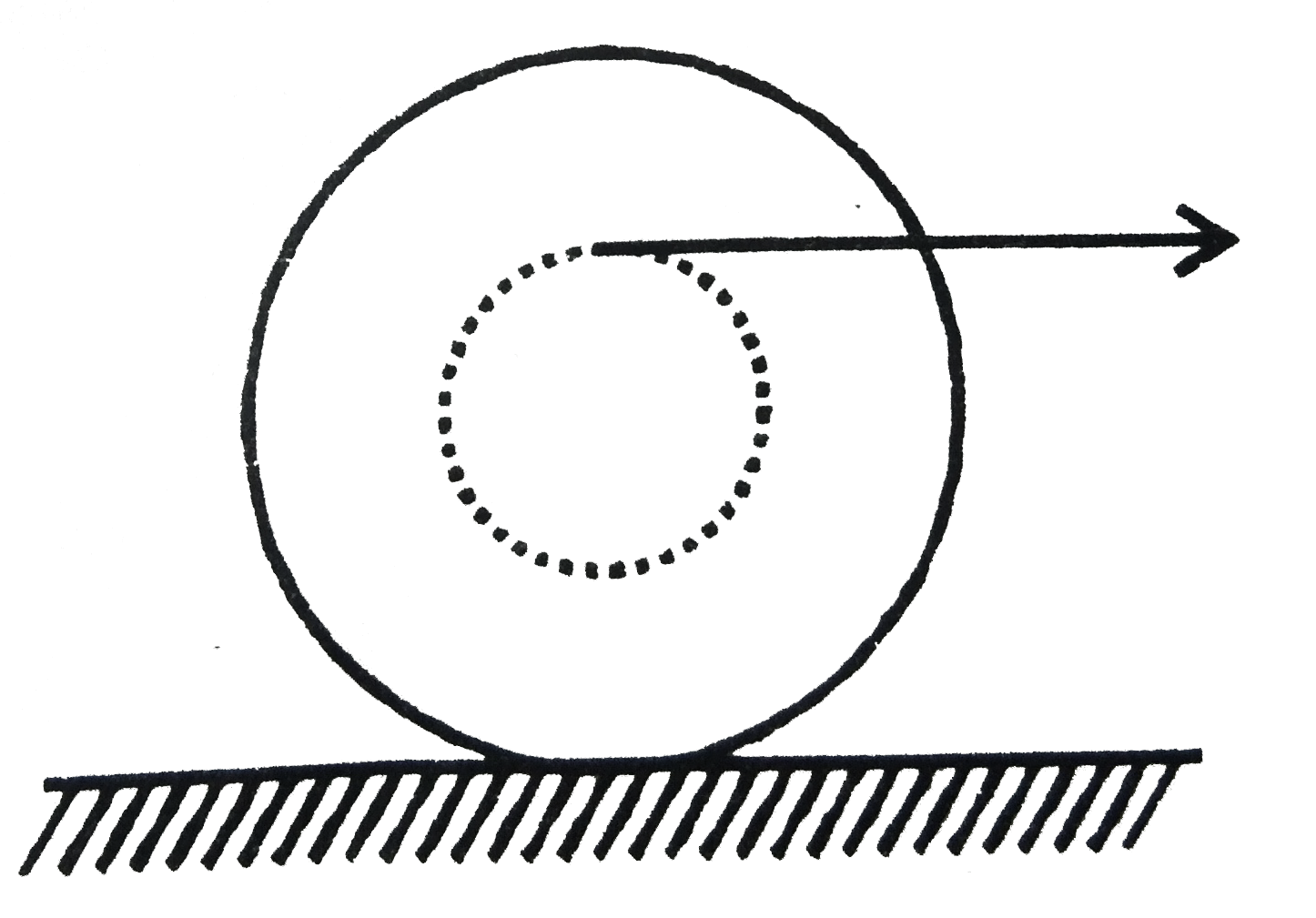 .
.
The correct Answer is:16m/s2
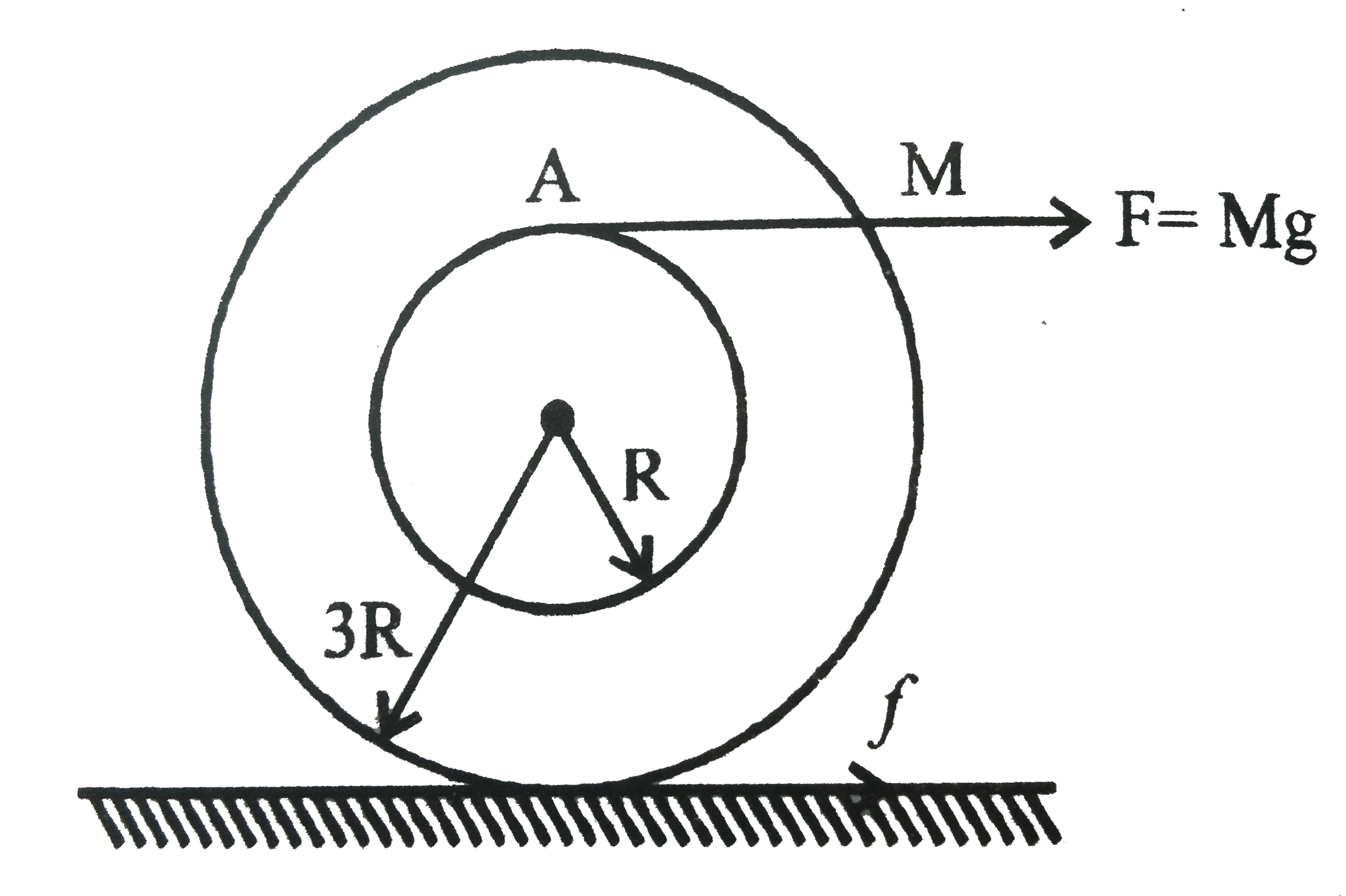
for translation motion F+f=Ma ….(1)
for rotational motion
F×R−f×3R=Iα⇒R(F−3f)=(MR2)α
F−3f=M2(3Rα)⇒F−3f=Ma3….(2)
Solving (1) and (2) we get
a=6g5 & α=2g5R
now aA=a+Rα
aA=6g5+2g5
aA=8g5⇒aA=16m/s2.
Similar Questions
Calculate the moment of inertia of a disc of radius R and mass M, about an axis passing through its centre and perpendicular to the plane.
View SolutionA cotton reel of mass m, radius R and moment of inertia I is kept on a smooth horizontal surface. If the string is pulled horizontally by a force F, find the (i) acceleration of CM, (ii) angular acceleration of the cotton reel.
 View Solution
View Solution
Knowledge Check
- Question 1 - Select One
The moment of inertia of a sphere of radius R about an axis passing through its centre is proportional to-
AR2BR3CR4DR5 - Question 2 - Select One
Moment of inertia of the earth about an axis passing through its centre of mass is (where R and ρ are radius and density of the earth respectively).
A(2/5)πR5ρB(2/3)πR5ρC(8/15)πR5ρD(4/15)πR5ρ - Question 3 - Select One
Moment of inertia of a ring of mass M and radius R about an axis passing through the centre and perpendicular to the plane is
A1/2MR2BMR2C1/4MR2D3/4MR2
A spherical conducting shell of inner radius r1 and outer radius r2 has a charge Q.
(a) A charge q is placed at the centre of the shell. What is the surface charge density on the inner and outer surfaces of the shell ?View Solution
A body of mass m, radius R and moment of inertia I (about an axis passing through the centre of mass and perpendicular to plane of motion) is released from rest over a sufficiently rough ground (to provide accelerated pure rolling) find linear acceleration of the body.View SolutionThe moment of inertia of a cylinder of radius R, length L and mass M about an axis passing through its centre of mass and normal to its length is
View SolutionA solid aluminimum sphere of radius R has moment of inertia I about an axis through its centre. The moment of inertia about a central axis of a solid aluminimum sphere of radius 2R is.
View SolutionA ring of radius R is rolling purely on the outer surface of a pipe of radius 4R At some instant the center of the ring has constant speed =v then the acceleration of the point on the ring which is in contact with the surface of the pipe is
View Solution

