A spring balance is attached to the ceiling of a lift. A man hangs his bag on the spring and the spring reads 49N, when the lift is stationary. If the lift moves downward with an acceleration of 5m/22, the reading of the spring balance will be
(a) 24N
(b) 74N
(c) 15N
(d) 49N
The correct Answer is:A
To solve the problem, we need to determine the reading of the spring balance when the lift is moving downwards with an acceleration of 5m/s2.
1. Identify the Forces:
- When the lift is stationary, the spring balance reads the weight of the bag, which is W=49N.
- The weight of the bag can be expressed as W=mg, where m is the mass of the bag and g is the acceleration due to gravity (g≈9.8m/s2).
2. Calculate the Mass of the Bag:
- We can find the mass m of the bag using the equation:
W=mg⟹m=Wg=49N9.8m/s2≈5kg
3. Apply Newton's Second Law:
- When the lift is accelerating downwards, the net force acting on the bag can be described by Newton's second law:
Fnet=ma
- The forces acting on the bag are the weight W acting downwards and the tension T in the spring acting upwards. The equation can be written as:
W−T=ma
- Here, a is the acceleration of the lift, which is 5m/s2.
4. Substitute the Known Values:
- We know W=49N, m=5kg, and a=5m/s2. Plugging these values into the equation gives:
49N−T=5kg×5m/s2
49N−T=25N
5. Solve for Tension T:
- Rearranging the equation to solve for T:
T=49N−25N=24N
6. Conclusion:
- The reading of the spring balance when the lift is moving downwards with an acceleration of 5m/s2 will be T=24N.
Final Answer:
The reading of the spring balance will be 24 N.
- When the lift is stationary, the spring balance reads the weight of the bag, which is W=49N.
- The weight of the bag can be expressed as W=mg, where m is the mass of the bag and g is the acceleration due to gravity (g≈9.8m/s2).
- We can find the mass m of the bag using the equation:
W=mg⟹m=Wg=49N9.8m/s2≈5kg
- When the lift is accelerating downwards, the net force acting on the bag can be described by Newton's second law:
Fnet=ma
- The forces acting on the bag are the weight W acting downwards and the tension T in the spring acting upwards. The equation can be written as:
W−T=ma
- Here, a is the acceleration of the lift, which is 5m/s2.
- We know W=49N, m=5kg, and a=5m/s2. Plugging these values into the equation gives:
49N−T=5kg×5m/s2
49N−T=25N
- Rearranging the equation to solve for T:
T=49N−25N=24N
- The reading of the spring balance when the lift is moving downwards with an acceleration of 5m/s2 will be T=24N.
The reading of the spring balance will be 24 N.
Topper's Solved these Questions
- BOOK - SUNIL BATRA (41 YEARS IITJEE PHYSICS)CHAPTER - HEAT AND THERMODYNAMICSEXERCISE - JEE Main And Advanced219videos
HEAT AND THERMODYNAMICS
- BOOK - SUNIL BATRA (41 YEARS IITJEE PHYSICS)CHAPTER - MECHANICAL PROPERTIES OF MATTER AND FLUIDSEXERCISE - Subjective Problems1videos
MECHANICAL PROPERTIES OF MATTER AND FLUIDS
Similar Questions
A spring balance is attached to the ceiling of a lift. When the lift is at rest spring balance reads 49 N of a body hang on it. If the lift moves :
(i) Downward
(ii) upward, with an acceleration of 5ms−2.
(iii) with a constant velocity.
What will be the reading of the balance in each case ?View SolutionWhat is the reading of the spring balance in the following device?
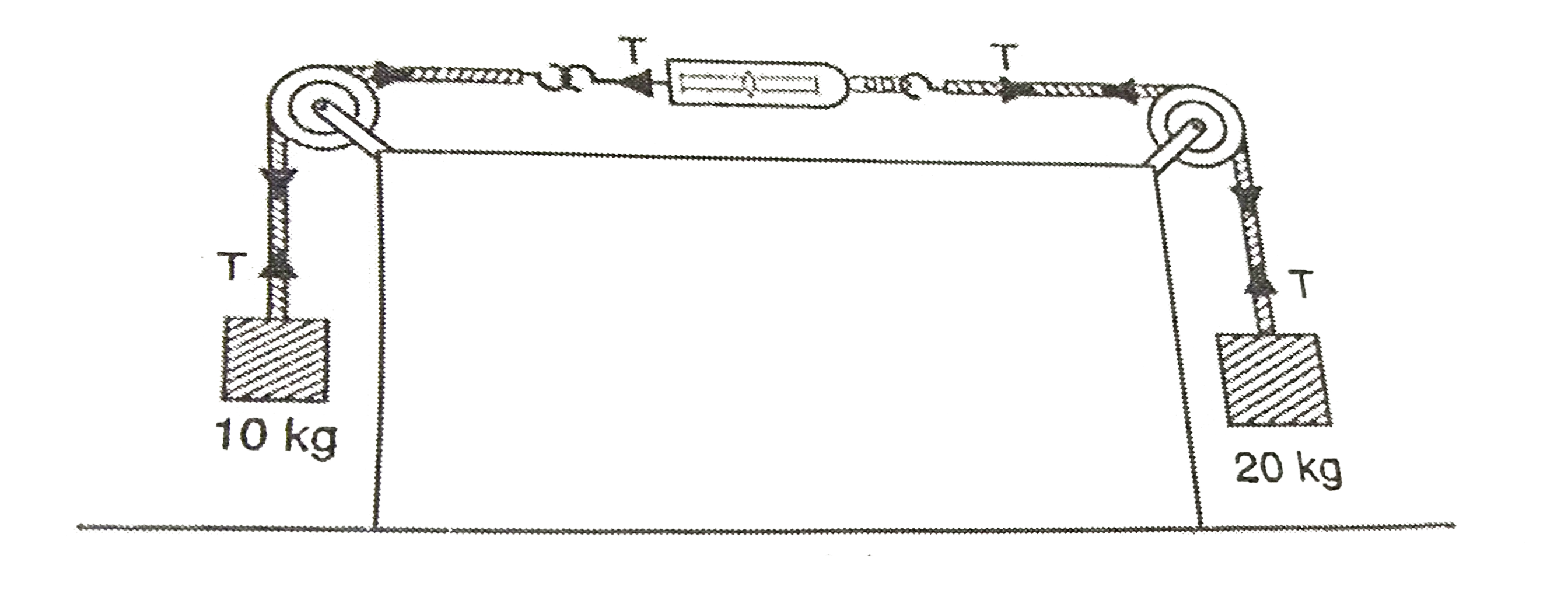 View Solution
View Solution
Knowledge Check
- Question 1 - Select One
A spring balance is attached to the ceiling of a lift. A man hangs his bag on the spring and the spring reads 49 N, when the lift is stationary. If the moves downwards with an acceleration of 5 ms−2, then reading of the spring balance will be
A49 NB24 NC74 ND15 N - Question 2 - Select One
The readings of the spring balance will be :
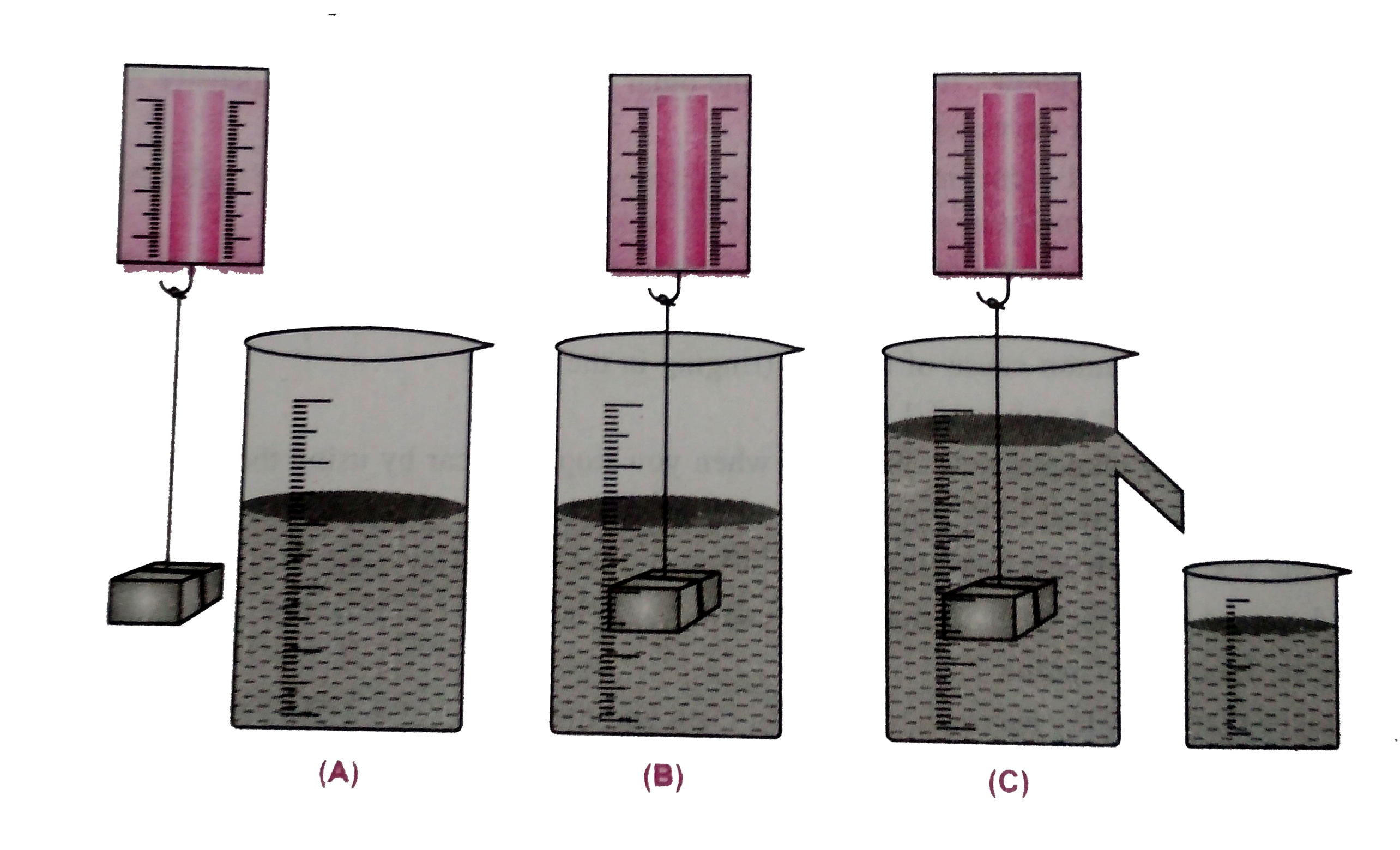 Aequal to each other in all cases A,BandC.Bequal to each other in cases AandC only.Cequal to each other in cases BandC only.Ddifferent in every case.
Aequal to each other in all cases A,BandC.Bequal to each other in cases AandC only.Cequal to each other in cases BandC only.Ddifferent in every case. - Question 3 - Select One
In the given figure, what is the reading of the spring balance?
 A10 NB20 NC5 NDZero
A10 NB20 NC5 NDZero
What is the reading of the spring balance in the following device?
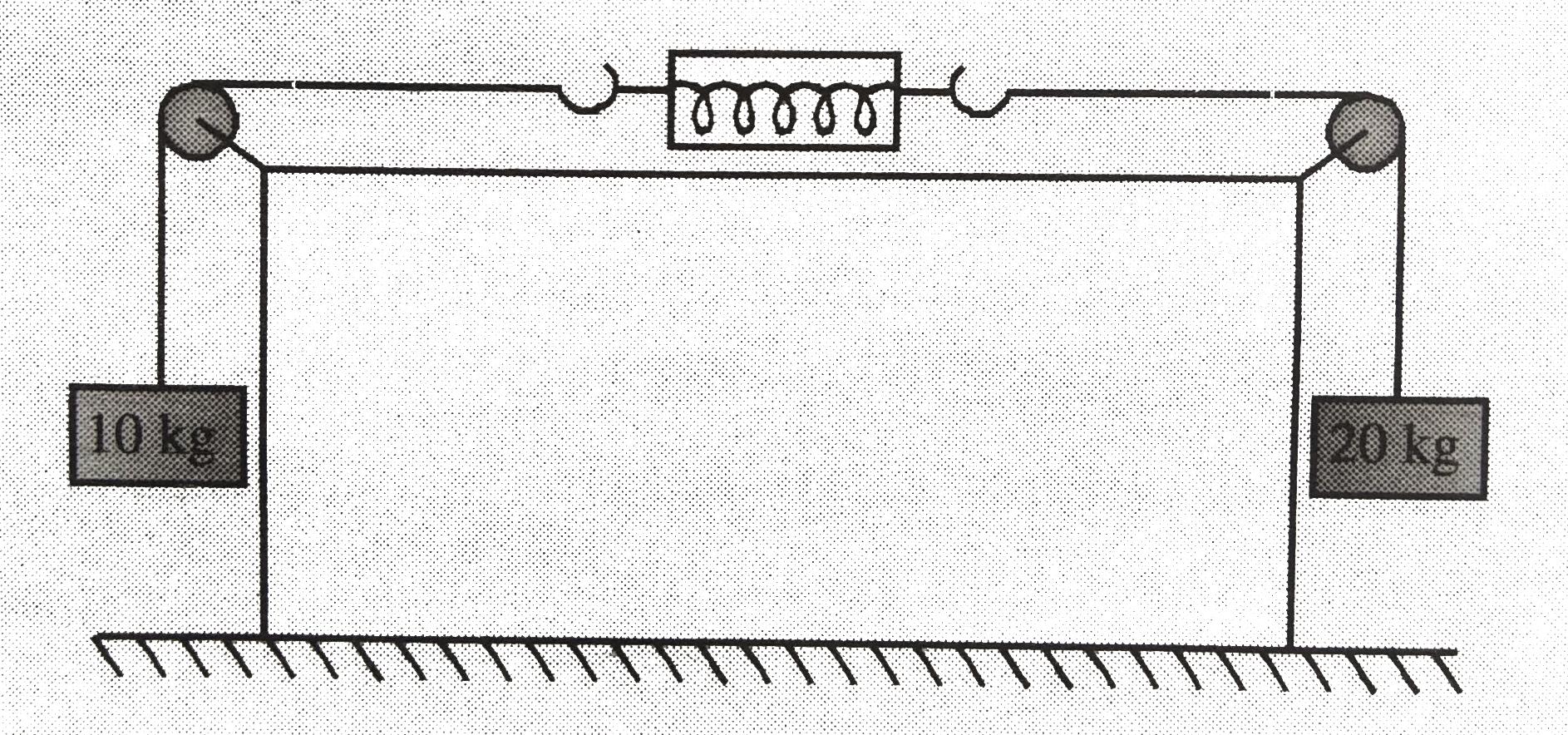 View Solution
View SolutionIn the above problem, if the lift moves up with a constant velocity of 2m/sec , the reading on the balance will be
View SolutionMass of a person sitting in a lift is 50 kg . If lift is coming down with a constant acceleration of 10 m/sec2 . Then the reading of spring balance will be (g=10m/sec2)
View SolutionFor the arrangement shown in the figure, the reading of spring balance is
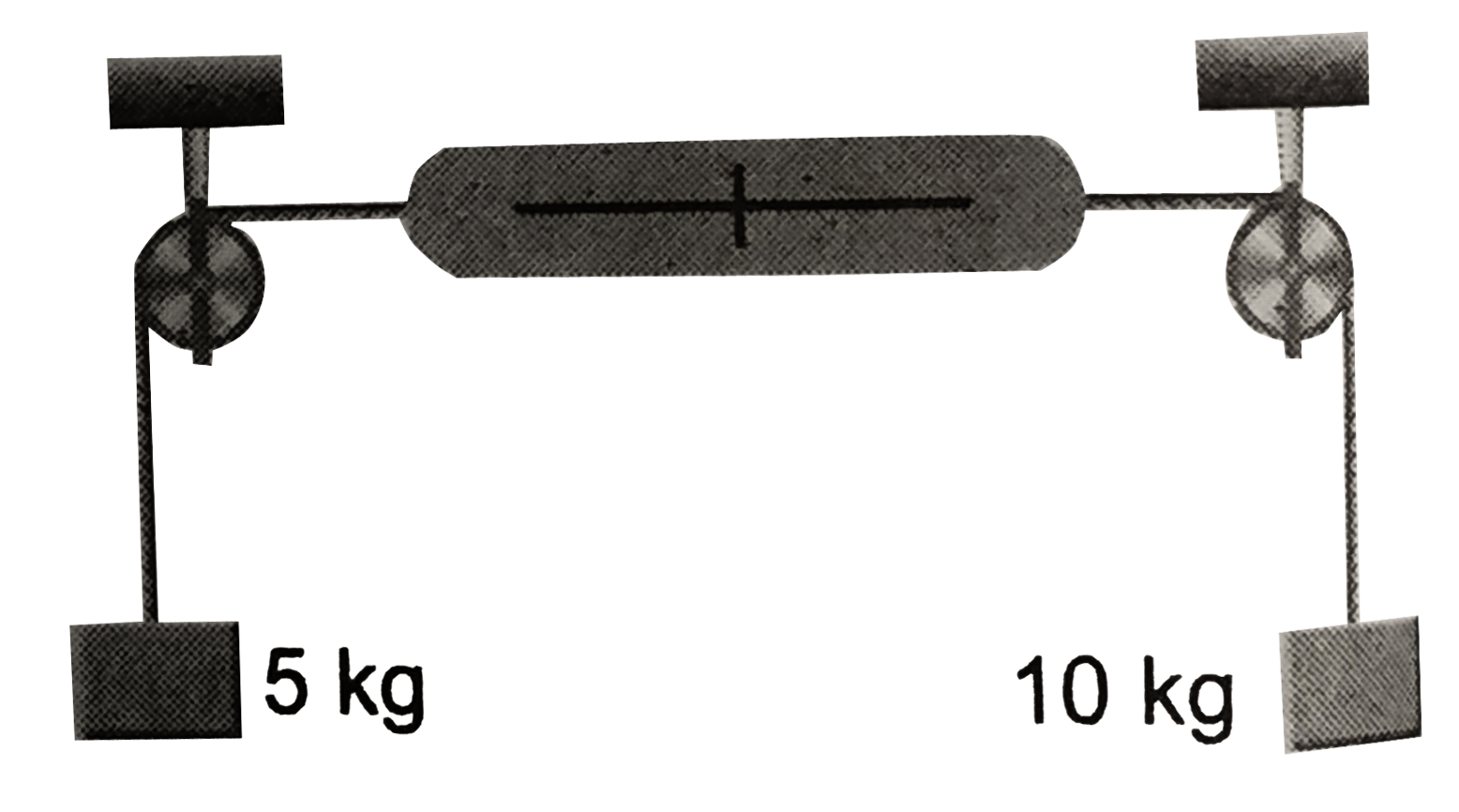 View Solution
View SolutionA man of mass 60 kg is standing on a spring balance inside a lift. If the lift falls downwards, then the reading of the spring balance will be:-
View Solution

