A squre hole of side length l is made at a depth of h and a circular hole is made at a depht of 4 h from the surface of water in a water tank kept on a horizontal surface (where l ltlt h). Find the radius r of the circular hole if equal amount of water comes out of the vessel through the holes per second.
Refer to 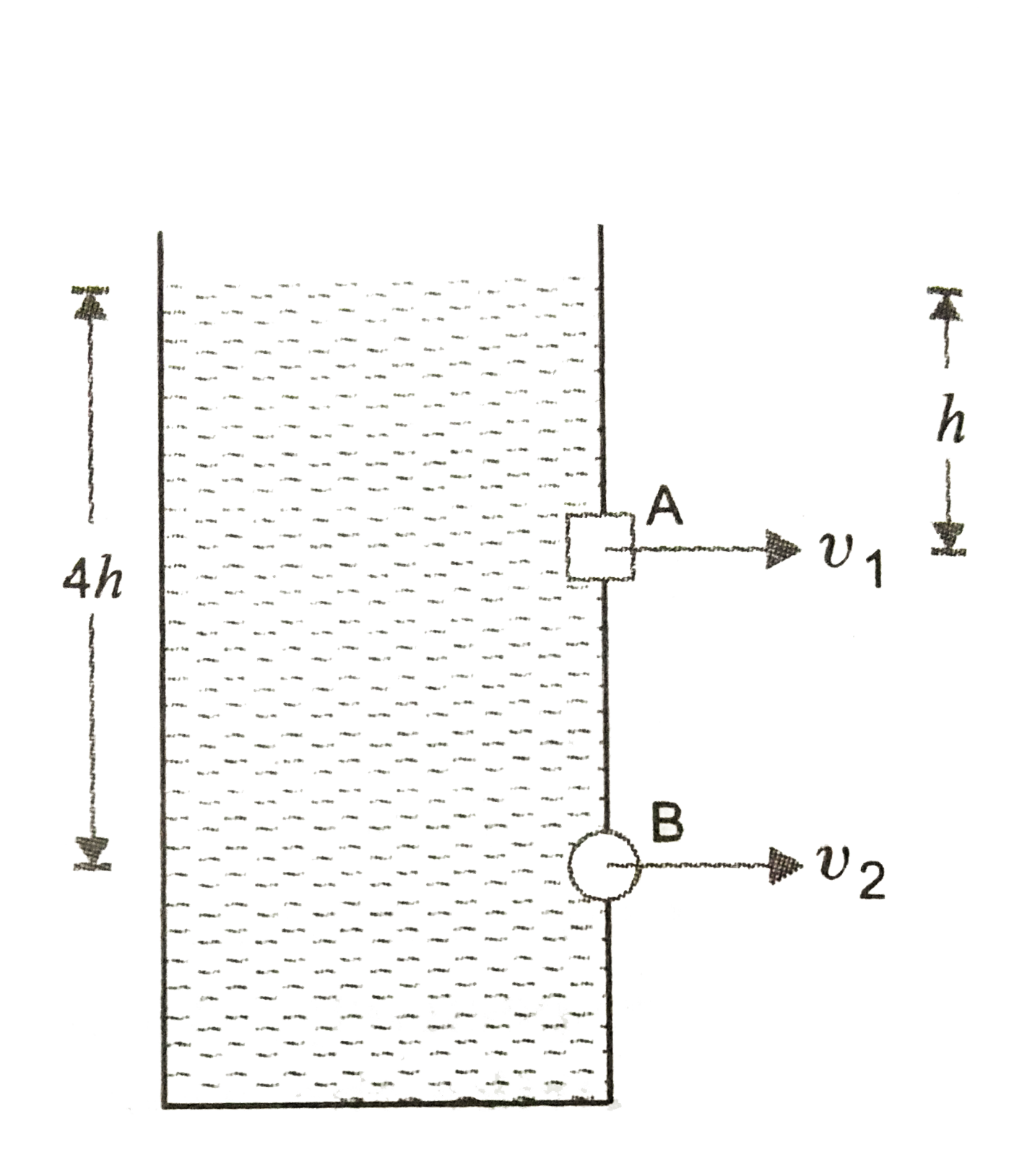 using equation of continuity at A and B, we have A1υ1=A2υ2
using equation of continuity at A and B, we have A1υ1=A2υ2
l2υ1=πr2υ2 …. (i)
Here, υ1=√2ghandυ2=√2g(4h) Putting values in (i) we have l2√2gh=πr2×√8ghorr=l/(√2π)
Topper's Solved these Questions
- PROPERTIES OF BULK MATTERBook:PRADEEPChapter:PROPERTIES OF BULK MATTERExercise:Value Based Question
Explore 5 Videos
- PROPERTIES OF BULK MATTERBook:PRADEEPChapter:PROPERTIES OF BULK MATTERExercise:Test Your Grip A
Explore 10 Videos
- PROPERTIES OF BULK MATTERBook:PRADEEPChapter:PROPERTIES OF BULK MATTERExercise:NCERT Questions
Explore 73 Videos
- PHYSICAL WORLD AND MEASUREMENTBook:PRADEEPChapter:PHYSICAL WORLD AND MEASUREMENTExercise:Competiton Focus Jee Medical Entrance
Explore 18 Videos
- RAY OPTICSBook:PRADEEPChapter:RAY OPTICSExercise:Problem For Practice(a)
Explore 25 Videos
Similar Questions
Two holes are made at a depth of h and 5 h from the water surface in a water container kept on a horizontal surface as shownn in figure. For which value of r, equalt amount of water will come out of the two holes? Here, l is the length of side of the square hole and r is the radius of circular hole.
View SolutionIn a vessel of water a hole is made at a depth of 3.5 m from the free surface. What would be the velocity of efflux ?
View Solution
Knowledge Check
A tank is filled to a height H. The range of water coming out of a hole which is a depth H/4 from the surface of water level is
A2H√3B√3H2C√3HD3H4A tank if filled with water upto height H. When a hole is made at a distance h below the level of water. What will be the horizontal range of water jet ?
A2√h(H−h)B4√h(H+h)C4√h(H−h)D2√h(H+h)A water tank has a hole in its wall at a distance of 40 m below the free surface of water. Compute the velocity of flow of water from the hole. If the radius of the hole is 1 mm. find the rate of flow of water
A26m/s,8.8×10−5m3/sB28m/s,8.8×10−5m3/sC28m/s,6.8×10−5m3/5D26m/s,9.8×10−5m3/s
A large open tank has two holes in the wall. One is a square hole of side L at a depth h from the top and the other is a circular hole of radius r at a depth 4h from the top. Whwn the tank is completely filled with water, the quantity of water flowing out per second from both the holes are the same. What is the value of r?
View SolutionIn a vessel containing water, a hole is made at a depth of 0.10 m from the free surface. What would be the velocity of efflux ?
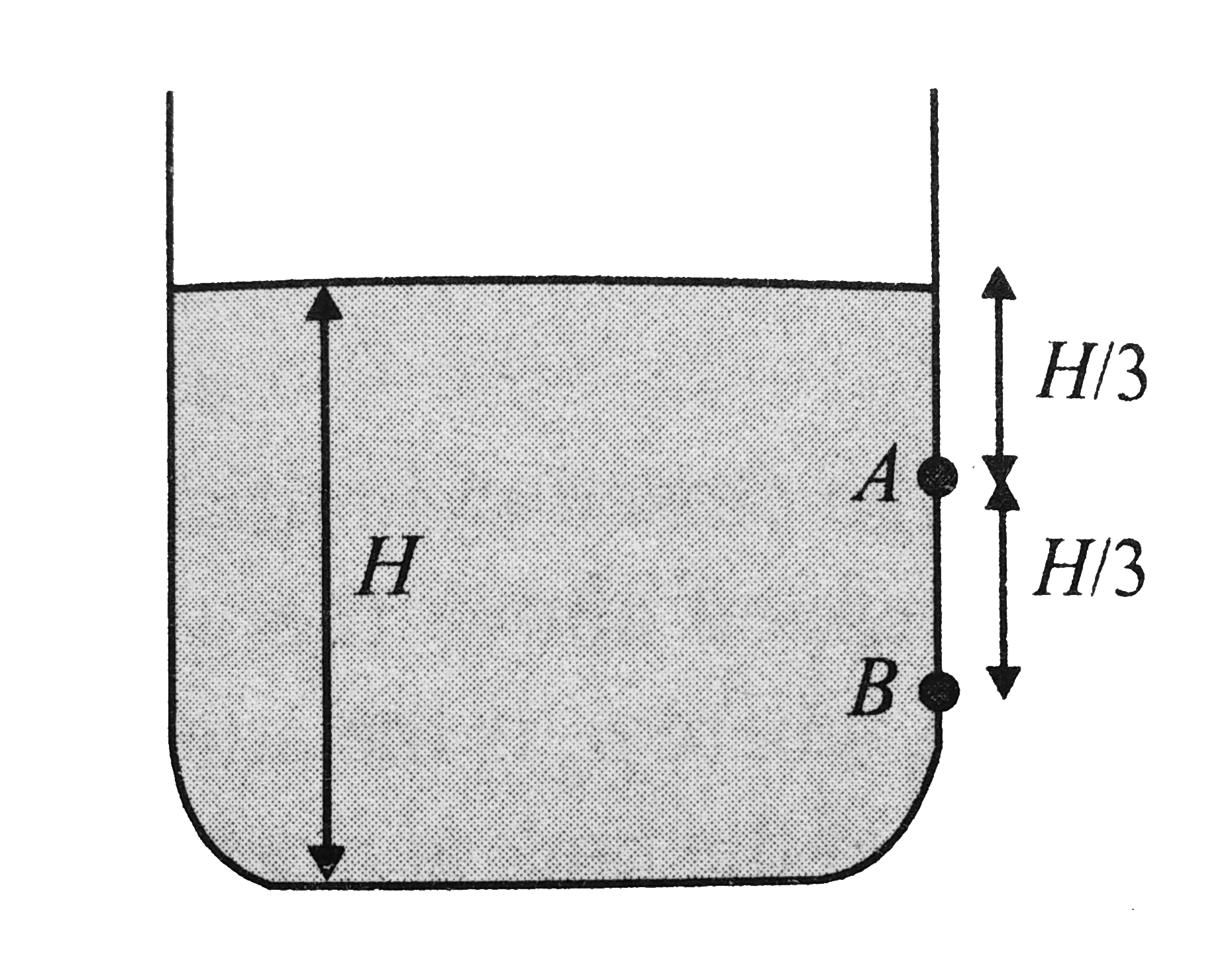
View SolutionThe area of two holes A and B are 2a and a, respectively, The holes are at height (H/3)and(2H/3) from the surface of water. Find the correct option(s):
View SolutionA cylinderical vessel is filled with water up to height H. A hole is bored in the wall at a depth h from the free surface of water. For maximum range h is equal to
View SolutionA large open tank has two holes in the wall. The top hole is a square hole of side L at a depth y from the surface of water. The bottom hole is a circular hole of radius R at a depth 4y from surface of water . If R=L/2√π, find the correct graph. V1 and V2 are the velocities in top and bottom holes. Area of the square & curcular holes are a1 and a2
View Solution
