A stone is dropped from a balloon at an altitude of 300m. How ling will the stone take to reach the ground of (a) the balloon is ascending with a velocity of 5,s−1.(b)theballonisdescend∈gwithavelocityof 5 ms^(-1) (c ) the balloon is shationary ?
To solve the problem of how long a stone takes to reach the ground when dropped from a balloon at an altitude of 300 m, we will consider three scenarios: (a) the balloon is ascending with a velocity of 5 m/s, (b) the balloon is descending with a velocity of 5 m/s, and (c) the balloon is stationary.
Given Data:
- Height (h) = 300 m
- Acceleration due to gravity (g) = 10 m/s² (approximate value)
(a) Balloon ascending with a velocity of 5 m/s
1. Determine Initial Velocity (u):
- Since the balloon is ascending, the initial velocity of the stone when dropped is +5 m/s (upward).
2. Use the equation of motion:
h=ut+12at2
Here, h=−300 m (downward), u=5 m/s (upward), and a=−g=−10 m/s² (downward).
Substituting the values:
−300=5t−12⋅10t2
−300=5t−5t2
Rearranging gives:
5t2−5t−300=0
Dividing the entire equation by 5:
t2−t−60=0
3. Solve the quadratic equation:
Using the quadratic formula t=−b±√b2−4ac2a:
Here, a=1,b=−1,c=−60.
t=−(−1)±√(−1)2−4⋅1⋅(−60)2⋅1
t=1±√1+2402
t=1±√2412
Approximating √241≈15.52:
t=1±15.522
The positive solution:
t≈16.522≈8.26 seconds
(b) Balloon descending with a velocity of 5 m/s
1. Determine Initial Velocity (u):
- The initial velocity of the stone when dropped is -5 m/s (downward).
2. Use the equation of motion:
−300=−5t+12(−10)t2
This simplifies to:
−300=−5t−5t2
Rearranging gives:
5t2+5t−300=0
Dividing by 5:
t2+t−60=0
3. Solve the quadratic equation:
Using the quadratic formula:
Here, a=1,b=1,c=−60.
t=−1±√12−4⋅1⋅(−60)2⋅1
t=−1±√1+2402
t=−1±√2412
Approximating √241≈15.52:
t=−1±15.522
The positive solution:
t≈14.522≈7.26 seconds
(c) Balloon stationary
1. Determine Initial Velocity (u):
- The initial velocity of the stone when dropped is 0 m/s.
2. Use the equation of motion:
−300=0+12(−10)t2
This simplifies to:
−300=−5t2
Rearranging gives:
5t2=300
t2=60
Taking the square root:
t=√60≈7.75 seconds
Summary of Results:
- (a) Time taken when balloon is ascending: 8.26 seconds
- (b) Time taken when balloon is descending: 7.26 seconds
- (c) Time taken when balloon is stationary: 7.75 seconds
- Height (h) = 300 m
- Acceleration due to gravity (g) = 10 m/s² (approximate value)
- Since the balloon is ascending, the initial velocity of the stone when dropped is +5 m/s (upward).
h=ut+12at2
Here, h=−300 m (downward), u=5 m/s (upward), and a=−g=−10 m/s² (downward).
−300=5t−12⋅10t2
−300=5t−5t2
Rearranging gives:
5t2−5t−300=0
Dividing the entire equation by 5:
t2−t−60=0
Using the quadratic formula t=−b±√b2−4ac2a:
Here, a=1,b=−1,c=−60.
t=−(−1)±√(−1)2−4⋅1⋅(−60)2⋅1
t=1±√1+2402
t=1±√2412
Approximating √241≈15.52:
t=1±15.522
The positive solution:
t≈16.522≈8.26 seconds
- The initial velocity of the stone when dropped is -5 m/s (downward).
−300=−5t+12(−10)t2
This simplifies to:
−300=−5t−5t2
Rearranging gives:
5t2+5t−300=0
Dividing by 5:
t2+t−60=0
Using the quadratic formula:
Here, a=1,b=1,c=−60.
t=−1±√12−4⋅1⋅(−60)2⋅1
t=−1±√1+2402
t=−1±√2412
Approximating √241≈15.52:
t=−1±15.522
The positive solution:
t≈14.522≈7.26 seconds
- The initial velocity of the stone when dropped is 0 m/s.
−300=0+12(−10)t2
This simplifies to:
−300=−5t2
Rearranging gives:
5t2=300
t2=60
Taking the square root:
t=√60≈7.75 seconds
- (a) Time taken when balloon is ascending: 8.26 seconds
- (b) Time taken when balloon is descending: 7.26 seconds
- (c) Time taken when balloon is stationary: 7.75 seconds
Topper's Solved these Questions
- BOOK - PRADEEPCHAPTER - KINEMATICSEXERCISE - 3 Problems for practice67videos
KINEMATICS
- BOOK - PRADEEPCHAPTER - KINEMATICSEXERCISE - 4 Problems for practice45videos
KINEMATICS
- BOOK - PRADEEPCHAPTER - KINEMATICSEXERCISE - 1 Problems for practice25videos
KINEMATICS
- BOOK - PRADEEPCHAPTER - GRAVIATIONEXERCISE - Assertion-Reason Type Questions19videos
GRAVIATION
- BOOK - PRADEEPCHAPTER - LAWS OF MOTIONEXERCISE - Assertion- Reason Type Questions17videos
LAWS OF MOTION
Similar Questions
A ball is dropped from an elevator at an altitude of 200m (Fig.4. 39). How much time will the ball take to reach the ground if the elevatior is
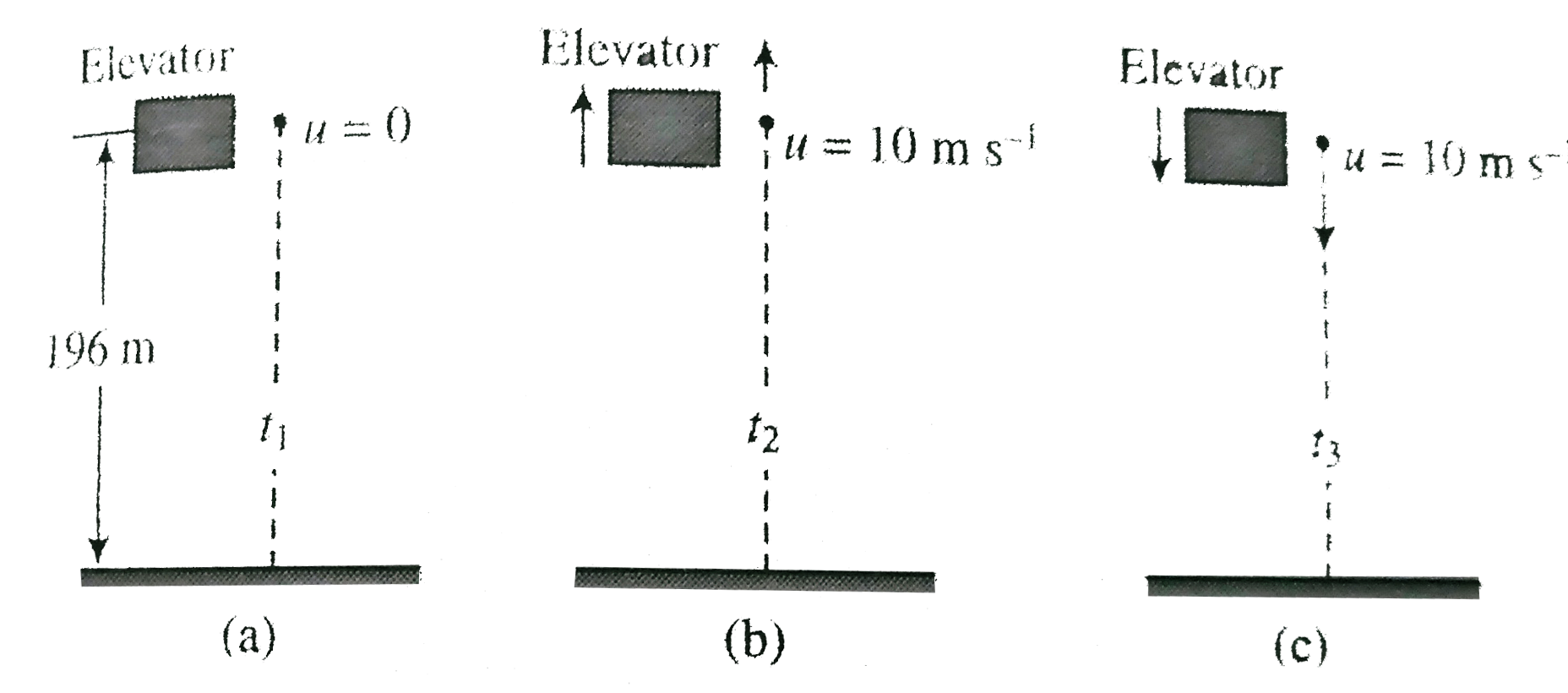 .
.
a. Stationary?
b. Ascending with velocity 10ms−1
c. Descending with velocity 10 m s^(-1)?View SolutionA stone is dropped from a rising balloon at a height of 76 m above the ground and reaches the ground in 6s. What was the velocity of the balloon when the stone was dropped?
View Solution
Knowledge Check
- Question 1 - Select One
A stone is dropped from roof of building takes 5 s to reach the ground. The sound of striking is heard after 5.4 second after the stone is dropped. The velocity of sound is
A312.5ms−1B332.5ms−1C352ms−1D392ms−1 - Question 2 - Select One
From a balloon, ascending with a velocity of 981 cm/sec., a stone is let fall and reaches the ground in 10 seconds. The height of balloon when the stone was dropped was
A392.4 mB400 mC450 mDNone of these - Question 3 - Select One
A balloon is at a height of 40 m and is ascending with a velocity of 10ms−1. A bag of 5 kg weight is dropped from it, when will the bag reach surface of earth ? (g=10m/s2)
A2 secB4 secC6 secD2√2sec
A stone is dropped from a balloon rising upwards with a velocity of 16ms−1. The stone reaches the ground in 4 s. Calculate the height of the balloon when the stone was dropped.
View SolutionA stone is dropped from a ballon rising upwards with a velocity of 16ms−1. The stone reached the ground in 4s. Calculate the height of the ballon when the stone was dropped.
View SolutionA stone is dropped from a balloon ascending with v0=2ms, from a height h=4.8 m .Find the time of flight of the stone.
View SolutionA ballon is ascending at the rate v=12km/h and is being carried horizontally by the wind at vw=20km/h. If a ballast bag is dropped from the balloon at the instant h = 50 m, determine the time needed for it to strike the ground. Assume that the bag was released from the ballon with the same velocity as the balloon. Also, find the speed with which the bag strikes the ground?
View SolutionA stone is dropped from a stationary hot air balloon in the air travels 14.7m in the last second before it hits the ground. Find the height of the balloon from the ground.
View Solution

