A string is wrapped around a cylinder of mass M and radius R. The string is pulled vertically upwards to prevent the centre of mass from falling as the cylinder unwinds the string. The tension in the string is
Mg6
Mg3
Mg2
2Mg3
The correct Answer is:B
To find the tension in the string wrapped around a cylinder of mass M and radius R, we can follow these steps:
Step 1: Identify the forces acting on the cylinder
The forces acting on the cylinder are:
- The weight of the cylinder, W=Mg (acting downwards)
- The tension in the string, T (acting upwards)
Step 2: Write the equation of motion for the cylinder
Since the center of mass of the cylinder is not falling, we can write the net force equation as:
Mg−T=Ma
where a is the downward acceleration of the cylinder.
Step 3: Relate linear acceleration to angular acceleration
The cylinder will also have an angular acceleration α due to the unwinding of the string. The relationship between linear acceleration a and angular acceleration α is given by:
a=αR
Step 4: Write the torque equation
The torque τ about the center of the cylinder due to the tension T is given by:
τ=T⋅R
This torque is also related to the moment of inertia I of the cylinder and its angular acceleration α:
τ=Iα
Step 5: Moment of inertia of the cylinder
For a solid cylinder, the moment of inertia I is given by:
I=12MR2
Step 6: Substitute the torque equation
Substituting the expressions for torque, we have:
T⋅R=12MR2α
Now, substituting α=aR:
T⋅R=12MR2⋅aR
This simplifies to:
T⋅R=12MRa
Dividing both sides by R:
T=12Ma
Step 7: Substitute a in terms of T
From the net force equation Mg−T=Ma, we can express a:
a=Mg−TM
Substituting this expression for a into the tension equation:
T=12M(Mg−TM)
This simplifies to:
T=12(Mg−T)
Multiplying through by 2:
2T=Mg−T
Adding T to both sides:
3T=Mg
Thus, we find:
T=Mg3
Final Answer
The tension in the string is:
T=Mg3
---
The forces acting on the cylinder are:
- The weight of the cylinder, W=Mg (acting downwards)
- The tension in the string, T (acting upwards)
Since the center of mass of the cylinder is not falling, we can write the net force equation as:
Mg−T=Ma
where a is the downward acceleration of the cylinder.
The cylinder will also have an angular acceleration α due to the unwinding of the string. The relationship between linear acceleration a and angular acceleration α is given by:
a=αR
The torque τ about the center of the cylinder due to the tension T is given by:
τ=T⋅R
This torque is also related to the moment of inertia I of the cylinder and its angular acceleration α:
τ=Iα
For a solid cylinder, the moment of inertia I is given by:
I=12MR2
Substituting the expressions for torque, we have:
T⋅R=12MR2α
Now, substituting α=aR:
T⋅R=12MR2⋅aR
This simplifies to:
T⋅R=12MRa
Dividing both sides by R:
T=12Ma
From the net force equation Mg−T=Ma, we can express a:
a=Mg−TM
Substituting this expression for a into the tension equation:
T=12M(Mg−TM)
This simplifies to:
T=12(Mg−T)
Multiplying through by 2:
2T=Mg−T
Adding T to both sides:
3T=Mg
Thus, we find:
T=Mg3
The tension in the string is:
T=Mg3
Topper's Solved these Questions
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - RIGID BODY DYNAMICS 2EXERCISE - Multiple Correct26videos
RIGID BODY DYNAMICS 2
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - RIGID BODY DYNAMICS 2EXERCISE - Linked Comprehension71videos
RIGID BODY DYNAMICS 2
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - RIGID BODY DYNAMICS 2EXERCISE - Subjective19videos
RIGID BODY DYNAMICS 2
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - RIGID BODY DYNAMICS 1EXERCISE - Integer11videos
RIGID BODY DYNAMICS 1
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - SOUND WAVES AND DOPPLER EFFECTEXERCISE - Integer16videos
SOUND WAVES AND DOPPLER EFFECT
Similar Questions
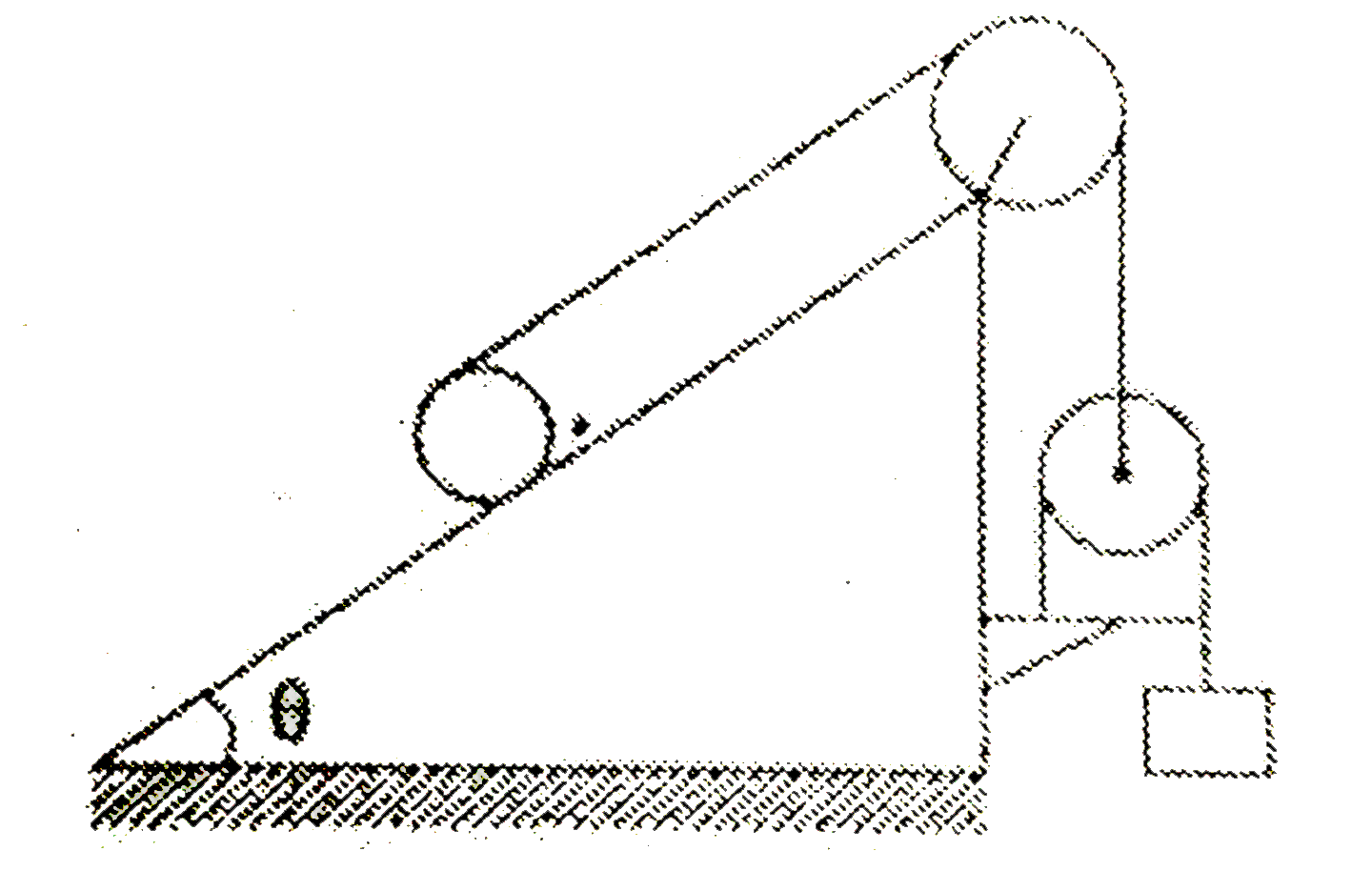
A string is warapped around a cylinder of mass m and radius r. The string is also connected to a block of same mass m with the help of another pulley as shown in figure. The angular acceleration of the cylinder is ( friction is sufficient for rolling ) ( all pulleys are ideal)
 View Solution
View SolutionThe block of mass m is at rest. Find the tension in the string A .
 View Solution
View SolutionA string is wrapped several times on a cylinder of mass M and radius R. the cylinder is pivoted about its adxis of block symmetry. A block of mass m tied to the string rest on a support positioned so that the string has no slack. The block is carefully lifted vertically a distance h, and the support is removed as shown figure.
a. just before the string becomes taut evalute the angular velocity ω0 of the cylinder ,the speed v0 of the falling body, m and the kinetic energy K0 of the system.
b. Evaluate the corresponding quanitities ω1,v1 and K1 for the instant just after the string becomes taut.
c. Why is K1 less than K0? Where does the energy go?
d. If M=m, what fraction of the kinetic energy is lost when the string becomes taut?
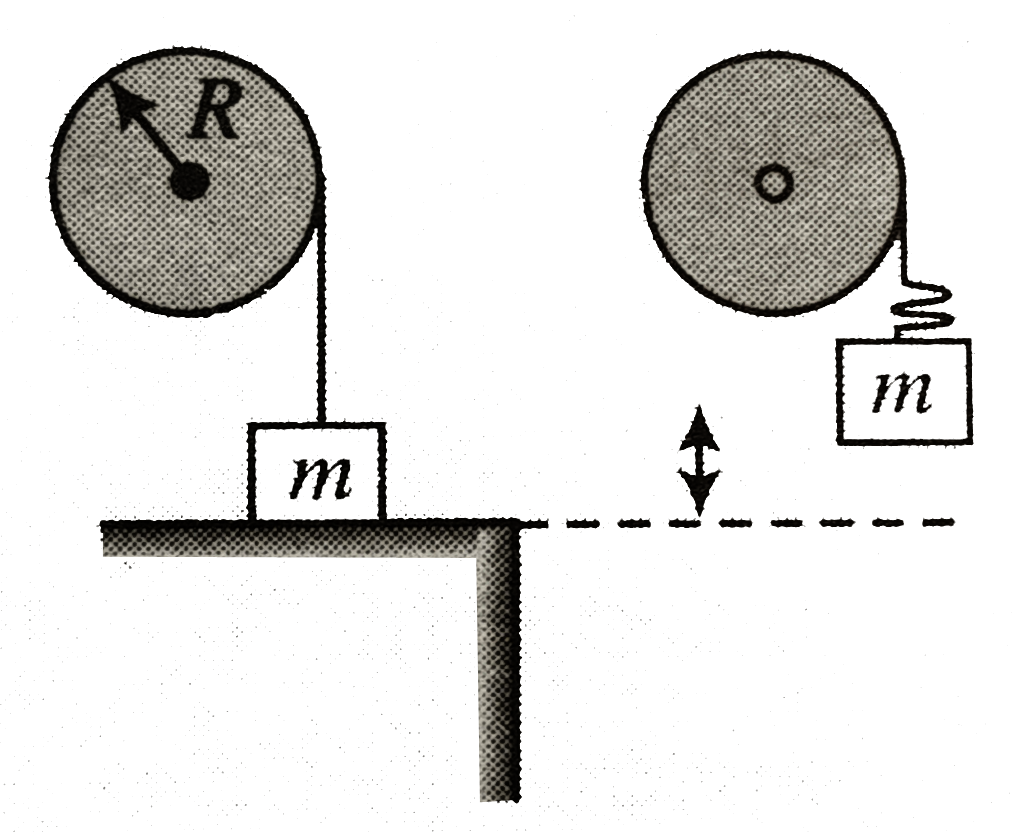 View Solution
View SolutionOne end of a string is tied to a rigid support attached to roof and the other end is wound around a uniform wheel of radius r and mass m. The string is kept vertical and the wheel is released from rest. As the wheel descends show that (i) the tension in the string is one-third the weight of the wheel, (ii) the magnitude of the acceleration of the centre of mass is 2g/3 and (iii) the speed of the centre of mass is (4gh/3)1/2
 View Solution
View SolutionA string is wound around a hollow cylinder of mass 5 Kg and radius 0.5 m. If the string is now pulled with a horizontal force of 40 N, the cylinder is rolling without slipping on a horizontal surface (see figure), then the angular acceleration of the cylinder will be (Neglect the mass and thickness of the string).
 View Solution
View SolutionOne end of a massless string is wound around a cylinder of mass 5 kg and radius 16' cm. The cylinder' is mounted symmetrically on a horizontal axle so 5 that It can freely rotate about its axis, to the other end of the string a mass of 500 gm is attached. Calculate the acceleration of the mass when it is released from rest.
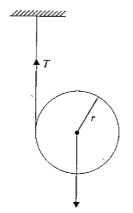
View SolutionOn the surface of a solid cylinder of mass 2 kg and radius 25 cm a string is wound several times and the end of the string is held stationary while the cylinder is released from rest. What is the acceleration of the cylinder and the tension in the string ?
 View Solution
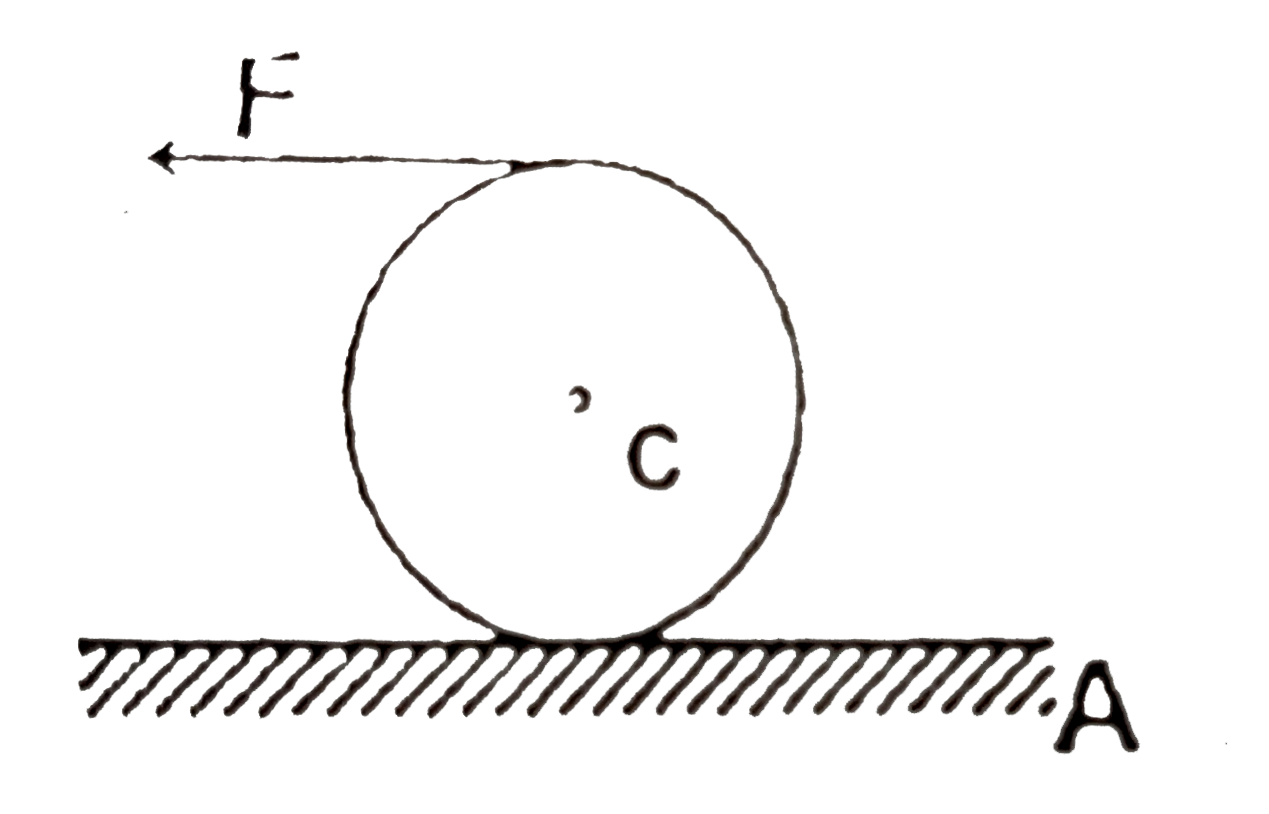
View SolutionA massless string is wrapped around a hollow cylinder having mass m and radius r. The cylinder is kept on a rough horizontal surface (coefficient of friction is μ). A constant force F is applied as shown in the figure. In case of pure rolling, the friction force acting on the bottom most point of the cylinder is
 View Solution
View SolutionThe string of a pendulum is horizontal. The mass of the bob is m. Now the string is released. The tension in the string in the lowest position is -
View Solution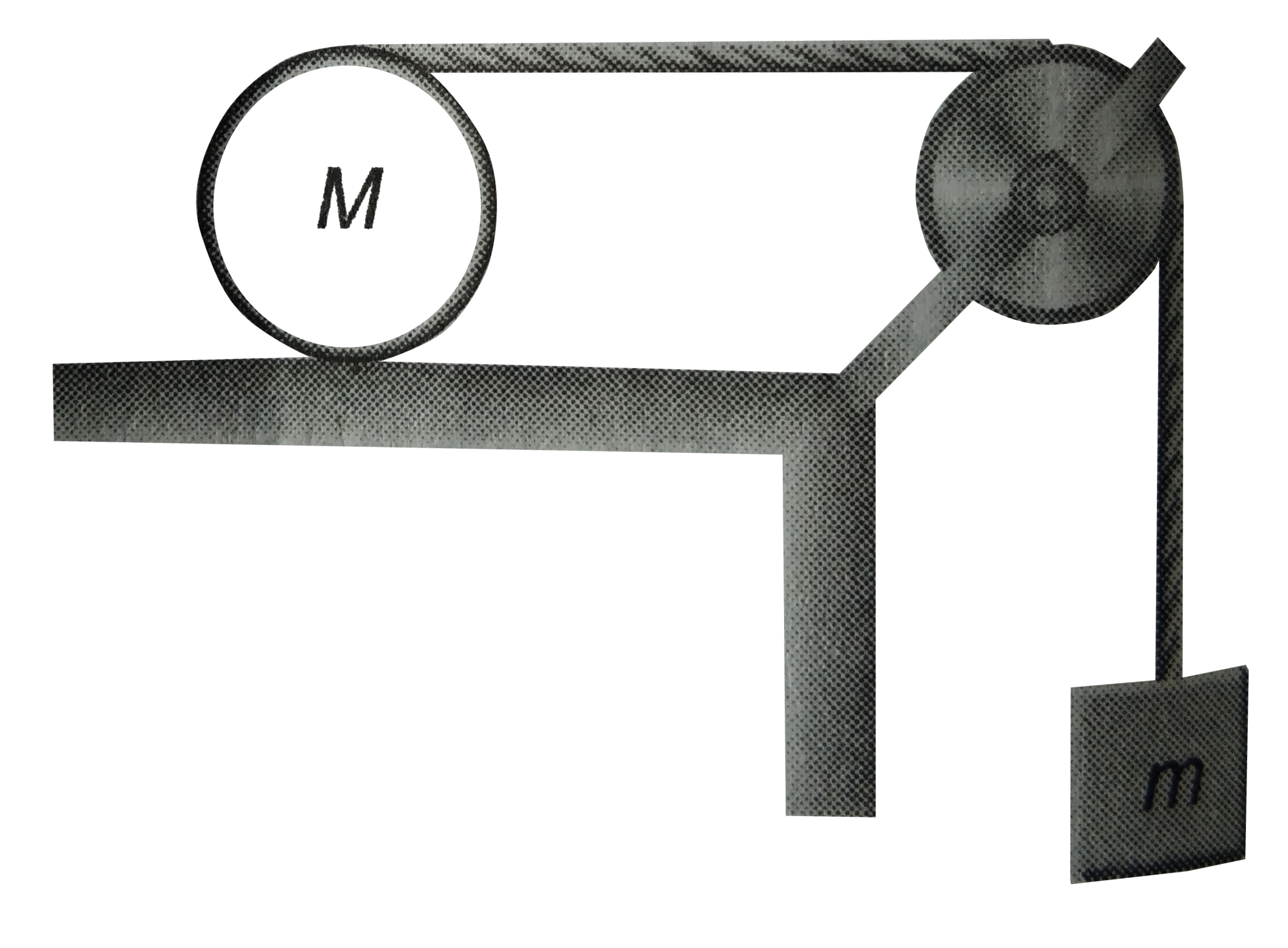
Consider the arrangement shown in figure. The string is wrapped around a uniform cylinder which rolls without slipping. The other end of the string is passed over a masslessm frictionless pulley to a falling weight, determine the acceleration of the falling mass m in terms of only the mass of the cylinder M, the mass m and gView Solution

