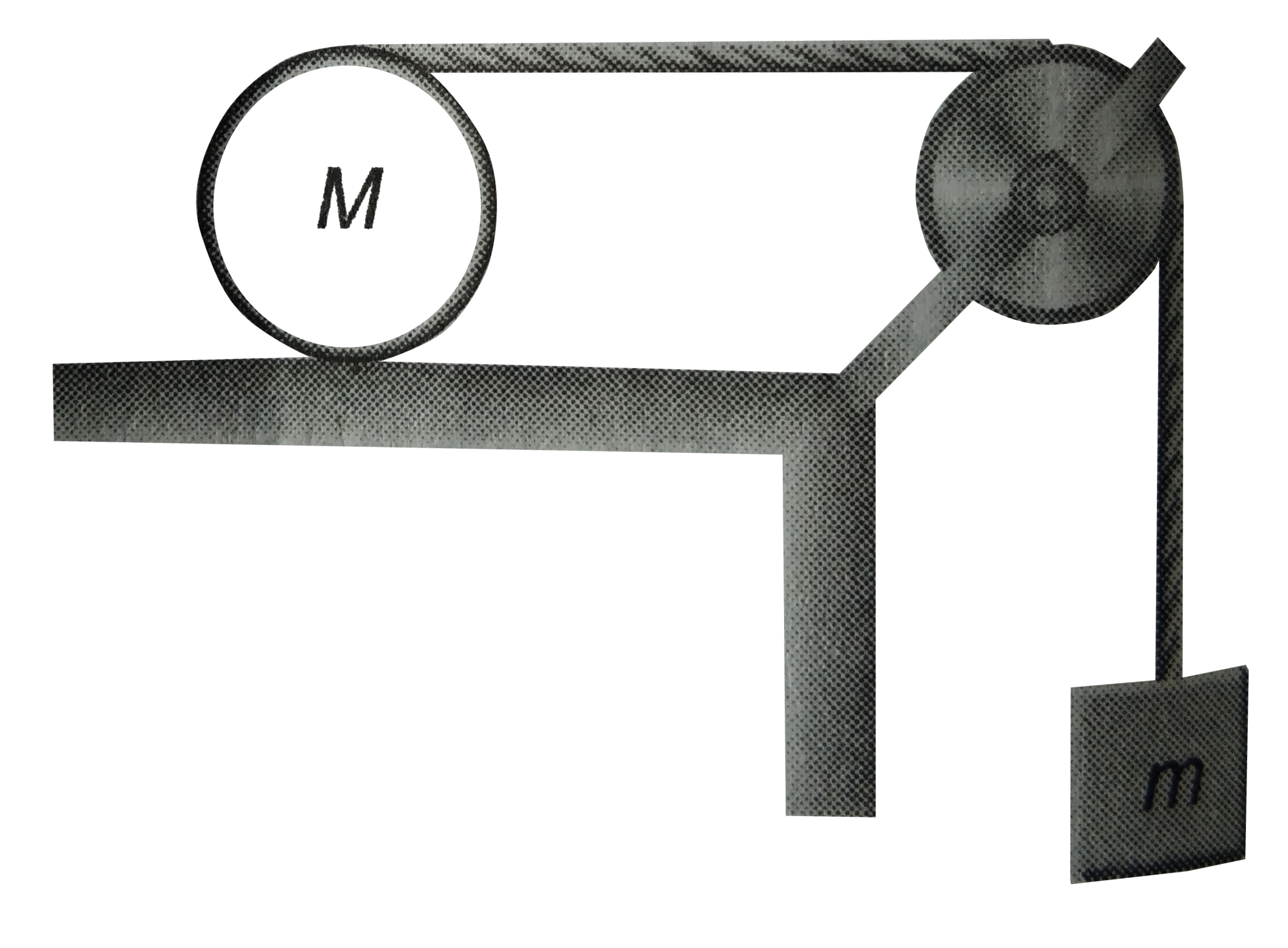
Consider the arrangement shown in figure. The string is wrapped around a uniform cylinder which rolls without slipping. The other end of the string is passed over a masslessm frictionless pulley to a falling weight, determine the acceleration of the falling mass m in terms of only the mass of the cylinder M, the mass m and g
let T be the tension in the string and f the force of (static) friction, between the cylinder and the surface.
a1= acceleration of centre of mass of cylinder
towards right
a2= downward acceleration of block m
α= angular acceleration of cylinder (clockwise)
Acceleration equations
for block mg−T=ma2
for cylinder T+f=Ma1 ..(ii)
α=(T−f)R12MR2 ..(iii)
Contact equations
The string is attached to the mass m at the highest point of the cylinder, hence
vm=vCOM+Rω ..
differentiating we get a2=a1+Rα ..(iv)
We also have (for rolling without slipping)
a1=Rα ..(v)
Solving these equations, we get
a2=8mg3M+8m
alternate solution (energy methode)
since, there is not slipping at all contact mechanical energy of the system will remain conserved.
∴ decrease in gravitational potential energy of block m in time t= increase in translational kinetic energy of block + increase in rotational as well as translational kinetic energy of cylinder
∴mgh=12mv22+12Iω2+12Mv21
or mg(12a2t2)=12m(a2t)2+12(12MR2)(αt)2+12M(a1t)2 ..(vi)
Solving Eqs. (iv) (V) and (vi) we get the same result.
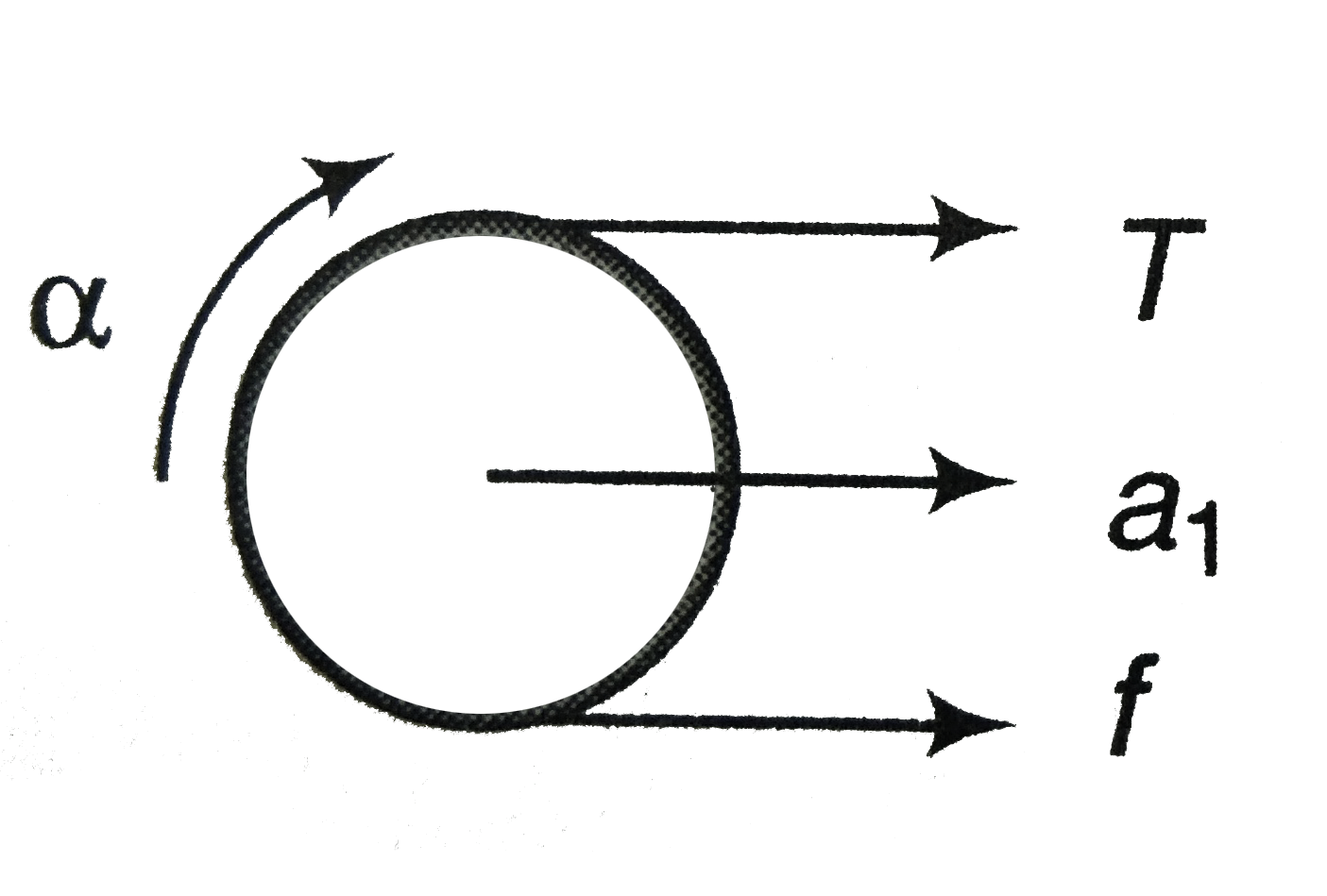

Topper's Solved these Questions
- ROTATIONAL MECHANICSBOOK - DC PANDEY ENGLISHCHAPTER - ROTATIONAL MECHANICSEXERCISE - Miscellaneous Examples
2 Videos
- ROTATIONAL MECHANICSBOOK - DC PANDEY ENGLISHCHAPTER - ROTATIONAL MECHANICSEXERCISE - Solved Example
1 Videos
- ROTATIONAL MECHANICSBOOK - DC PANDEY ENGLISHCHAPTER - ROTATIONAL MECHANICSEXERCISE - Subjective Questions
2 Videos
- ROTATIONBOOK - DC PANDEY ENGLISHCHAPTER - ROTATIONEXERCISE - (C) Chapter Exercises
39 Videos
- ROTATIONAL MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - ROTATIONAL MOTIONEXERCISE - Integer Type Questions
17 Videos
Similar Questions
Two particles of masses m and M(M>m) are connected by a cord that passes over a massless, frictionless pulley. The tension T in the string and the acceleration a of the particles is
View SolutionTwo bodies of mass 4 kg and 6 kg tied to the ends of a massless string. the string passes over a pully which is frictionless (see figure). the acceleration of the system in terms of acceleration due to gravity (g) is:
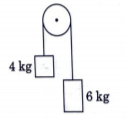
View SolutionTwo bodies of masses 4 kg and 6 kg are tied to the ends of a massless string. The string passes over a frictionless pulley. The acceleration of the system is :
View SolutionTwo masses 2 kg and 4 kg are connected at the two ends of light inextensible string passing over a frictionless pulley. If the masses are released, then find the acceleration of the masses and the tension in the string.
View SolutionTwo blocks of masses m1=4kg and m2=2kg are connected to the ends of a string which passes over a massless, frictionless pulley. The total downwards thrust on the pulley is nearly

View SolutionTwo masses of 3 kg and 4 kg are connected at the two ends of a light inextensible string that passes over a frictionless pulley. Find the acceleration of the masses and the tension in the string, when the masses are released.
View SolutionA spool (consider it as a double disc system joined by a short tube at their centre) is placed on a horizontal surface as shown Fig. A light string wound several times over the short connecting tube leaves it tangentially and passes over a light pulley. A weight of mass m is attached to the end of the string. The radius of the connecting tube is r and mass of the spool is M and radius is R. Find the acceleration of the falling mass m. Neglect the mass of the connecting tube and slipping of the spool.

View SolutionA string is wrapped around a cylinder of mass M and radius R. The string is pulled vertically upwards to prevent the centre of mass from falling as the cylinder unwinds the string. The tension in the string is
View SolutionIf the string & all the pulleys are ideal, acceleration of mass m is :-
View SolutionTwo bodies of masses m1 and m2(m1>m2) respectively are tied to the ends j of a string which passes over a light frictionless pulley. The masses are intitially at rest and released. What is the acceleration of the centre of mass ?
View Solution
