A triangular loop as shown in the figure is started to being pulled out at t=0 form a uniform magnetic field with a constant velocity v. Total resistancee of the loop is constant and equal to R. Then the variation of power produced in the loop with time will be :

power produced in the loop increasing linearly with time till whole loop comes out
power produced in the loop increases parabolically till whole loop comes out
Current in the loop increases linearly with time till whole loop comes out
power produced in the loop will be constant with time
The correct Answer is:B, C
Topper's Solved these Questions
- ELECTROMAGNETIC INDUCTIONBOOK - CENGAGE PHYSICSCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - single correct
10 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - CENGAGE PHYSICSCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - comprehensive
5 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - CENGAGE PHYSICSCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Single Correct Answer Type
26 Videos
- ELECTROMAGENTIC INDUCTIONBOOK - CENGAGE PHYSICSCHAPTER - ELECTROMAGENTIC INDUCTIONEXERCISE - QUESTION BANK
40 Videos
- ELECTRON,PHONTS,PHOTOELECTRIC EFFECT & X-RAYSBOOK - CENGAGE PHYSICSCHAPTER - ELECTRON,PHONTS,PHOTOELECTRIC EFFECT & X-RAYSEXERCISE - dpp 3.3
15 Videos
Similar Questions
A semi-circular loop of radius R is placed in a uniform magnetic field as shown. It is pulled with a constant velocity. The induced emf in the loop is
View SolutionA conducting loop is being pulled with speed v from region I of magnetic field to region II. If resistance of the loop is R, current induced in the loop at the instant shown is
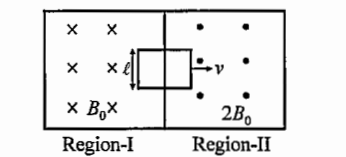
View Solution
Knowledge Check
A triangle loop as shown in the figure is started to being pulled out at t=0 from a uniform magnetic field with a constant velocity v.Total resistance of the loop is constant and equals to R.Then the variation of power produced in the loop with time will be:
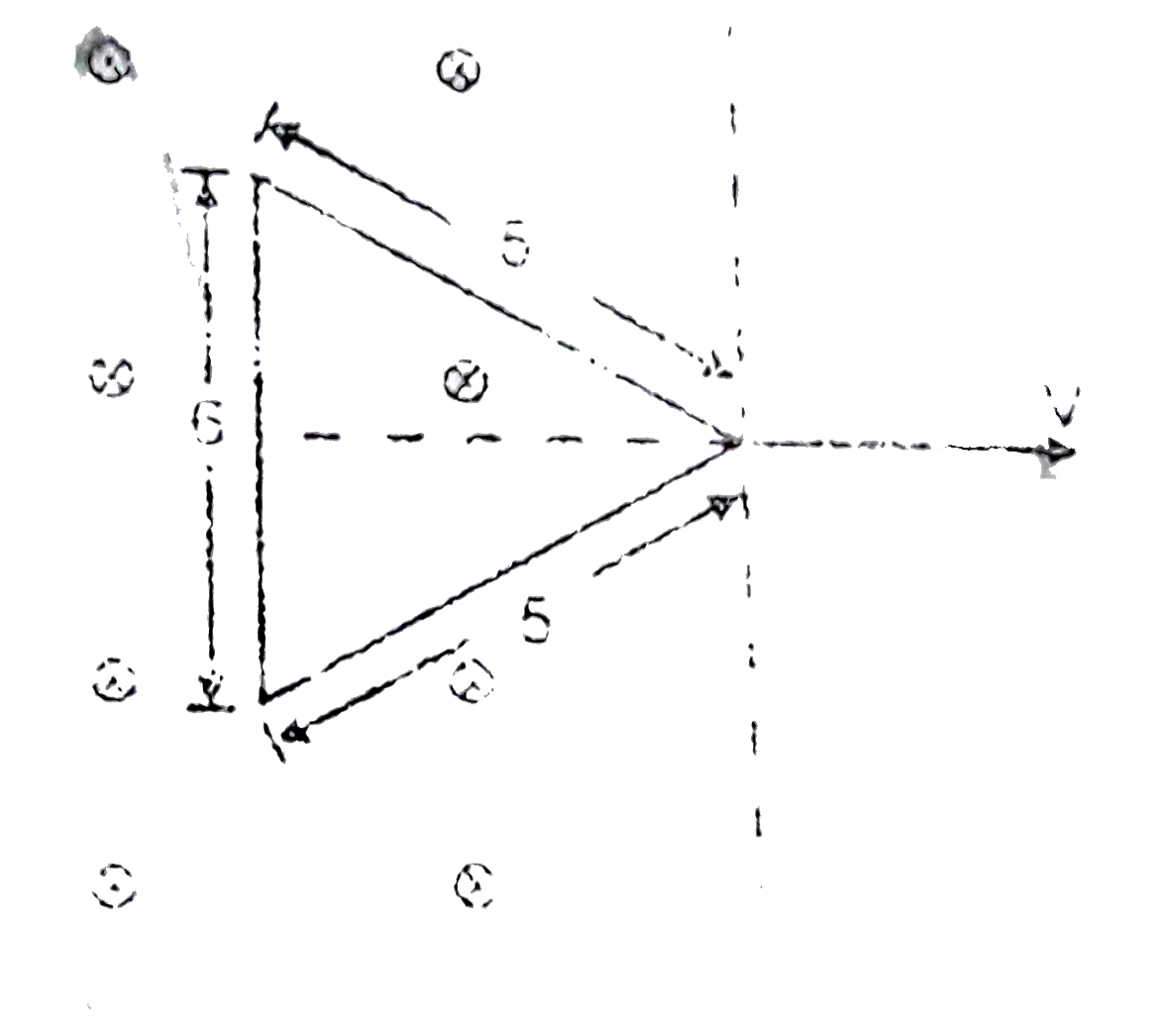 Alinearly increasing with time till whole loop come outBincreases parabolically till whole loop comes outCPαt3 till whole loop come outDwill be constant with time
Alinearly increasing with time till whole loop come outBincreases parabolically till whole loop comes outCPαt3 till whole loop come outDwill be constant with timeA rectangular loop of resistance R, and sides l and X, is pulled out of a uniform magnetic field B with a for maintaining uniform velocity of withdrawal is __
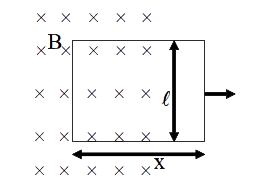 ABl2vRBB2l2vRCB2l2v2RD0
ABl2vRBB2l2vRCB2l2v2RD0An equilateral triangular loop ADC having some resistance is pulled with a constant velocity v out of a uniform magnetic field directed inot the paper. At time t=0, side DC of the loop at is at edge of the magnetic field.
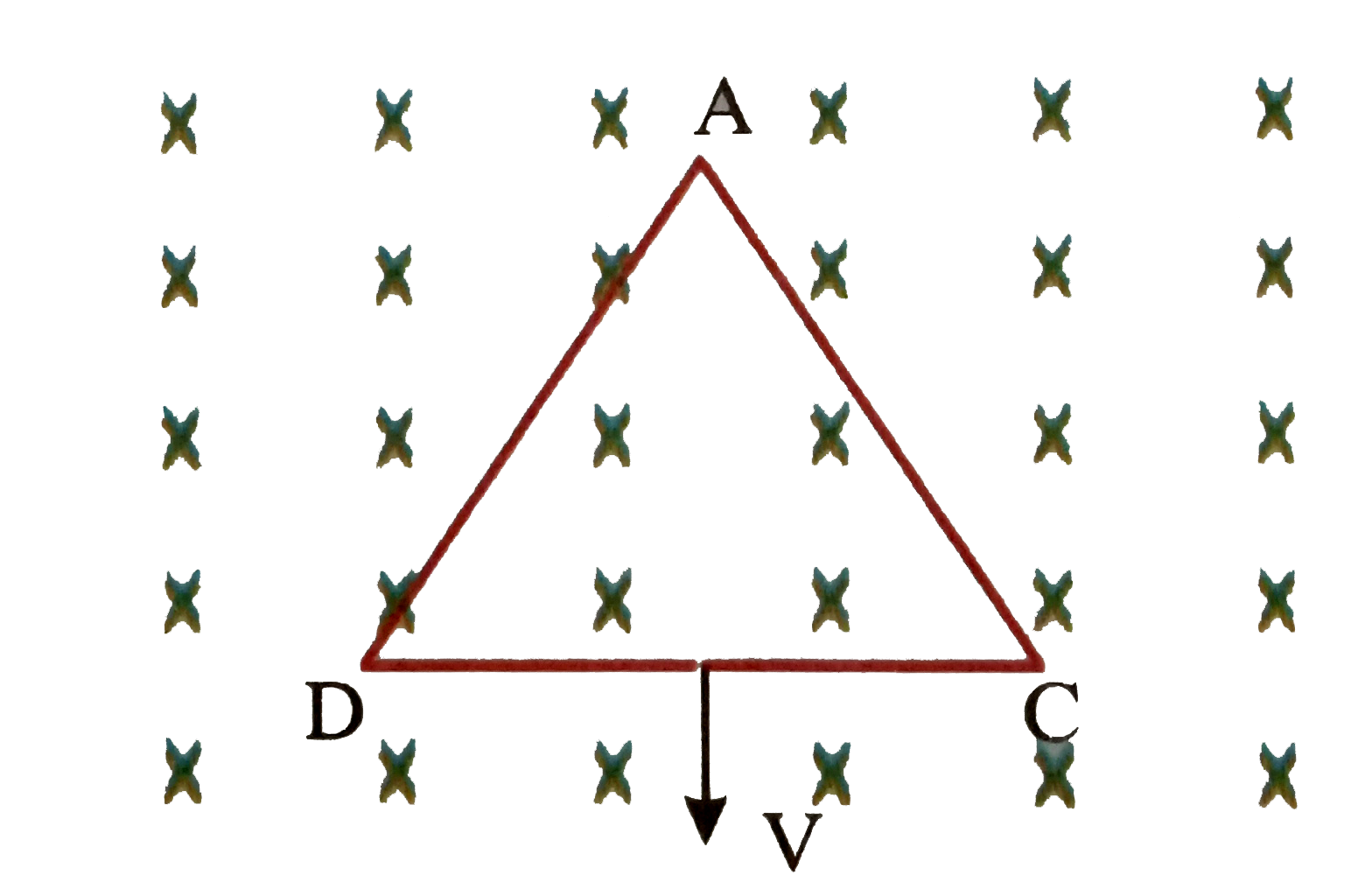
The induced current (i) versus time (t) graph will be asA B
B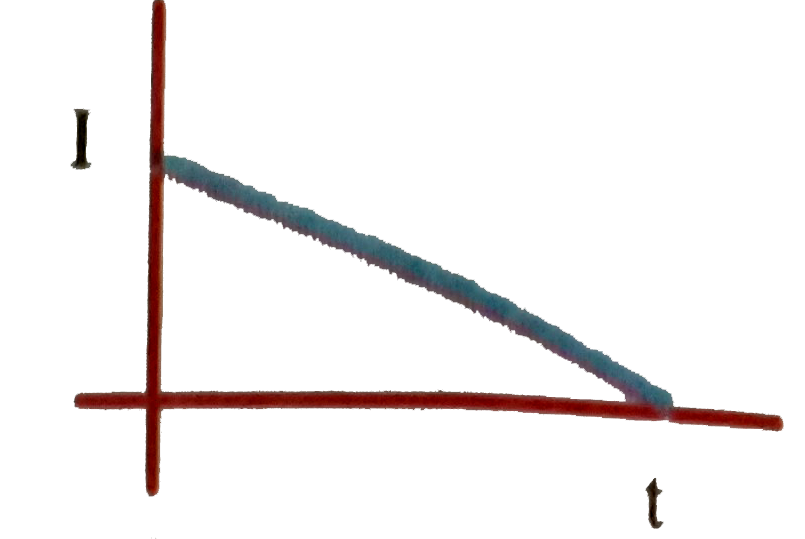 C
C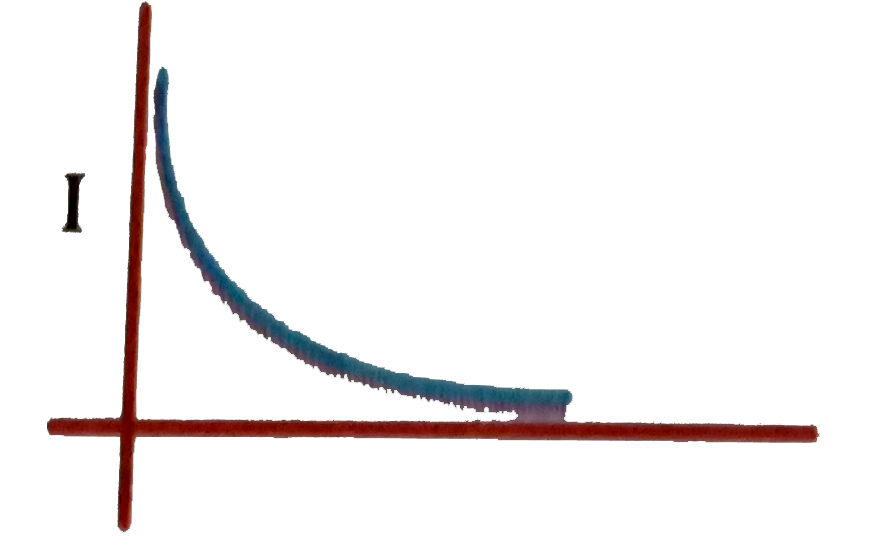 D
D
An equilateral triangular loop ADC having some resistance is pulled with a constant velocity v out of a uniform magnetic field directed in to the paper. At time t=0, side DC of the loop at is at edge of the magnetic field.

The induced current (i) versus time (t) graph will be as
View SolutionA rectangular, a square , a circular and an elliptical loop, all in the (x−y) plane, are moving out of a uniform magnetic field with a constant velocity →v=vˆi . The magnetic field is directed along the negative z-axis direction. The induced emf, during the passage of these loops , out of the field region, will not remain constant for :
View SolutionA circular loop of metal wire of radius r is placed perpendicular to uniform magnetic field B. Half of the loop is folded about the diameter with constant angular velocity ω. If resistance of the loop is R then current in the loop is
View SolutionA rectangular, a square, a circular and an elliptical loop, all in the x-y plane, are moving out of a uniform magnetic field with a constant velocity vcev=v0ˆi. The magnetic field is directed along the negative z-axis direction. The induced emf, during the passage of these loops, out of the field region, will not remain constant for
View SolutionA circular conducting loop of radius R and resistance per unit length λ is pulled out from the regio of uniform magnetic filed with constant velocity v. The situation shown in the figure corresponds to that is at t=0. Mark out the correct statement (s)

View Solution
