A uniform horizontal disc fixed at its centre to an elastic vertical rod performs forced torsional oscillations dur to the moment of forces Nz=Nmcosωt. The oscialltions obey the law φ=φmcos(ωt−α).
(a) the work performed by friction forces acting on the disc during one oscillation period ,
(b) the quality factor of the given oscillator if th emoment of inertia of the disc relative to the axis is equal to I.
The equation of the disc is ..φ+2β.φ+ω20φ=NmcosωtI
Then as before φ=φmcos(ωt−α)
where φ(m)=NmI[(ω20−ω2)2+4β2ω2]1/2,tanα=2βωω20−ω2
(a) Work performed by frictional forces
=−∫Nrdφ whereNr=−2Iβ.φ=−∫T02βI.φ2dt=−2πβωIφ2m
=−πIφ2m[(ω20−ω2)2+4β2ω2]1/2sinα=−πNmφmsinα
(b) The quality factor
Q=πλ=πβT=√ω20−β22β=ω√ω20−β2(ω20−ω2)tanα=1tanα⎧⎨⎩4ω2ω20(ω20−ω2)2−4β2ω2(ω20−ω2)2⎫⎬⎭
=12tanα{4ω2ω20I2φ2mN2mcos2α−tan2α}sinceω20=ω2+NIφmcosα
=12sinα{4ω2ω20I2φ2mN2m−sin2α}1/2
=12sinα{4ω2I2φ2mN2m(ω2+NmcosαIφm)+1−cos2α}1/2
=12sinα{4I2φ2mN2mω4+4IφmNmω2cosα+cos2α−1}1/2=12sinα⎧⎨⎩(2Iφmω2Nm+cosα)2−1⎫⎬⎭1/2
Topper's Solved these Questions
- OSCILLATIONS AND WAVESBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - OSCILLATIONS AND WAVESEXERCISE - Electric Oscillations
56 Videos
- OSCILLATIONS AND WAVESBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - OSCILLATIONS AND WAVESEXERCISE - Elastic Waves
39 Videos
- OPTICSBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - OPTICSEXERCISE - Exercise
2 Videos
- PHYSICAL FUNDAMENTALS OF MECHANICSBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - PHYSICAL FUNDAMENTALS OF MECHANICSEXERCISE - Relativistic Mechanics
49 Videos
Similar Questions
Due to the external vertical force Fx=F0cosωt a body suspended by a spring performs forced steady − state oscillations according to the lawx=acos(ωt−φ). Find the work performed by the force F during one oscillation period.
View SolutionFind the period of small torsional oscillational of a system consisting of two discs slipped oon a thin rod with rod with torsional coefficient k. The moments of inertia of discs relative to the rod's axis are equal to I1 and I2.
View Solution
Knowledge Check
For a particle performing linear SHM, its average speed over one oscillation is (where, a= amplitude of SHM, n=frequency of oscillation)
A2 anB4 anC6 anD8 an
A body performes torsional oscillations according to the law φ=φ0e−βtcosωt. Find : (a) the angular velocity .φ and the angular acceleration ..φ of the body ar the moment t=0,
(b) the moment of time at which the angular velocity becomes maximum.
View SolutionA uniform disc of mass m and radius r is suspended through a wire attached to its centre. If the time period of the torsional oscillations be T, what is the torsional constant of the wire?
View SolutionWhat is a torsional pendulum ? Name the factors on which its period of oscillation depends ?
View SolutionA uniform disc of mass m and radius r is suspended through a wire attached to its Centre. If the time period of the torsional oscillations be T, what is the torsional constant of the wire?
View SolutionA disc of mass 'm' is suspended at a point R/2 above its centre. Find its period of oscillation.
View SolutionA ball of mass m suspended by a weightless spring can perform vertical oscillations with damping coefficient β. The natural oscillation frequency is equal to ω0. Due to the external vertical force varying as F=F0cosωt the ball performs steady − state harmonic oscillations. Find :
(a) the mean power (:P:) , develocped by the force F. averaged over one oscillations perod,
(b) the frequency ω of the force F at which (:P:) is maximum, what is (:P:)max equal to ?
View SolutionA disc A of radius R suspended by an elastic threat between two stationary plane ( figure ) performs torsional oscillations about its axis OO′ . The moment of inertia of the disc relative to that axis is equal to I, the clearance between the disc and each of the planes is equal to h, with h<<R. Find the viscosity of the gas surrounding the disc A if the oscilaltion period of the disc equals T and the logarithmic damping decrement, λ.
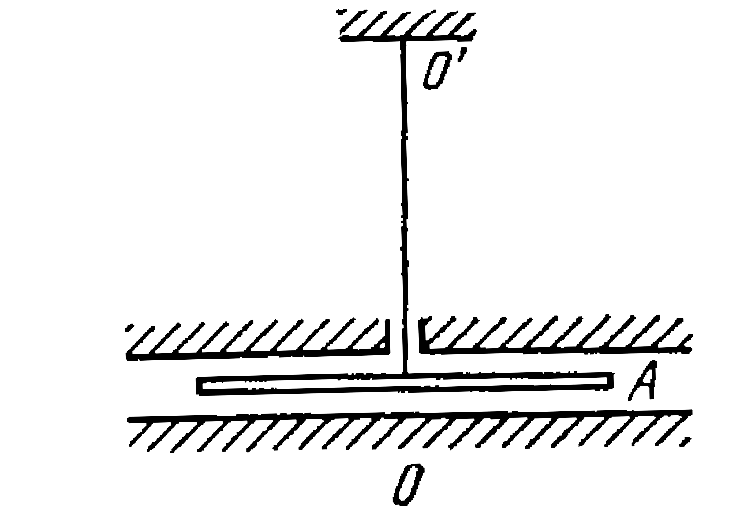
View Solution
